…+ s²_B)/B. The ratio of to is the same as the distribution of namely a Student’s t_v (v degrees of freedom), so the two-sample t statistic is distributed as a (central) Student’s t on v degrees of freedom:

The FBI criterion for a match on this one element can be written

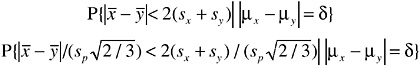
Because E(s_x) = E(s_y) = 0.8812σ, and E(s_p) ≈ σ if v > 60, this reduces very roughly to

The approximation is very rough because E(P{t < S}) ≠ P{t < E(S)}, where t stands for the two-sample t statistic and S stands for But it does show that if δ is very large, this probability is virtually zero (very small false match probability because the probability that the sample means would, by chance, end up very close together is very small). However, if δ is small, the probability is quite close to 1.

The equivalence t test proceeds as follows. Assume

where H₀ is the null hypothesis that the true population means differ by at least δ, and the alternative hypothesis is that they are within δ of each other. The two-sample t test would reject H₀ in favor of H₁ if the sample means are too close, that is, if where K_α(n,δ) is chosen so that does not exceed a preset per-element risk level of α (in Chapter 3, we used α = 0.30). Rewriting that equation, and writing K_α for K_α(n,δ),

When v is large s_p ≈ 0, and therefore the quantity