7
Evolution of Individuality During the Transition from Unicellular to Multicellular Life
RICHARD E. MICHOD
Individuality is a complex trait, yet a series of stages each advantageous in itself can be shown to exist allowing evolution to get from unicellular individuals to multicellular individuals. We consider several of the key stages involved in this transition: the initial advantage of group formation, the origin of reproductive altruism within the group, and the further specialization of cell types as groups increase in size. How do groups become individuals? This is the central question we address. Our hypothesis is that fitness tradeoffs drive the transition of a cell group into a multicellular individual through the evolution of cells specialized at reproductive and vegetative functions of the group. We have modeled this hypothesis and have tested our models in two ways. We have studied the origin of the genetic basis for reproductive altruism (somatic cells specialized at vegetative functions) in the multicellular Volvox carteri by showing how an altruistic gene may have originated through cooption of a life-history tradeoff gene present in a unicellular ancestor. Second, we ask why reproductive altruism and individuality arise only in the larger members of the volvocine group (recognizing that high levels of kinship are present in all volvocine algae groups). Our answer is that the selective pressures leading to reproductive altruism stem from the increasing cost of reproduction with increasing group size. Concepts from population genetics and evolutionary biology
Department of Ecology and Evolutionary Biology, University of Arizona, Tucson, AZ 85721.
appear to be sufficient to explain complexity, at least as it relates to the problem of the major transitions between the different kinds of evolutionary individuals.
The theme of this article, which could well be the theme of this Colloquium, is that evolutionary biology can explain complexity. I will consider the problem of explaining the “major transitions” between the different kinds of evolutionary individuals that make up the familiar hierarchy of life: genes, bacteria-like cells, cells-in-cells (eukaryotic cells), multicellular organisms, and societies (Maynard Smith and Szathmáry, 1995). Evolutionary individuals are integrated and indivisible wholes with the property of heritable variation in fitness so that they may evolve adaptations at their level of organization. Being wholes, evolutionary individuals may be thought to be irreducibly complex, but this has not been the case during evolutionary history; a series of stages, each advantageous in itself, may be shown to exist allowing evolution to get from one kind of individual to another. The evolutionary concepts we use to understand evolutionary transitions in individuality involve fitness and its reorganization, fitness tradeoffs (especially the cost of reproduction to survival) and their roles in life-history evolution, and kin selection and altruism and their roles in social evolution. We focus on the transition from unicellular to multicellular life, but the points made apply more generally to the other transitions (Michod, 1999).
Our understanding of life is being transformed by the realization that evolution occurs not only through the standard processes operating within populations, but also during evolutionary transitions in individuality, when groups of individuals become so integrated that they evolve into new higher-level individuals. Indeed, the major landmarks in the diversification of life and the hierarchical organization of the living world are consequences of a series of evolutionary transitions: from genes to gene networks to the first cell, from prokaryotic to eukaryotic cells, from cells to multicellular organisms, from asexual to sexual populations, and from solitary to social organisms. Such transitions require the reorganization of fitness, by which we mean the transfer of fitness from the old lower-level individual to the new higher level, and the specialization of lower-level units in fitness components of the new higher-level individual. It is a major challenge to understand why (environmental selective pressures) and how (underlying genetics, population structure, physiology, and development) the basic features of an evolutionary individual, such as fitness heritability, indivisibility, and evolvability, shift their reference from the old level to the new level.
The evolution of multicellular organisms is the premier example of the integration of lower-level individuals (cells) into a new higher-level
individual. How does a cell group evolve into a multicellular individual? This is the central question asked in this article. Although kinship has long been appreciated as a necessary precondition for the transition to multicellularity (Maynard Smith, 1988, 1991; Maynard Smith and Szathmáry, 1995; Michod, 1999), there are colonial species with high degrees of kinship that have not evolved true individuality (based on specialization of cells at reproductive and vegetative functions). For example, in all colonial members of the volvocine green algae (Fig. 7.1), all cells in the colony are clonally derived from a single cell, often by just a few cell divisions, yet true individuality based on specialization of reproductive and somatic functions emerges only in the larger colonies. What additional factors are required for the evolution of reproductive altruism, that is, specialization at vegetative somatic functions? Specialization of reproductive and vegetative viability-enhancing functions, what we term germ soma
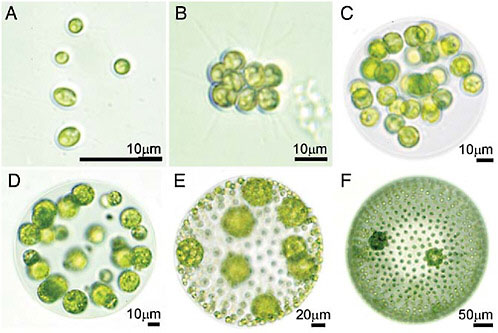
FIGURE 7.1 Examples of volvocine species varying in cell number, colony volume, degree of specialization, and proportion of somatic cells. (A) Chlamydomonas reinhardtii, a unicell. (B) Gonium pectorale, a flat or curved sheet of 8–32 undifferentiated cells. (C) Eudorina elegans, a spherical colony of 16–64 undifferentiated cells. (D) Pleodorina californica, a spherical colony with 30–50% somatic cells. (E) Volvox carteri. (F) Volvox aureus. Where two cell types are present (D–F), the smaller cells are somatic cells and the larger cells are reproductive cells. Photos were taken by C. Solari (University of Arizona).
specialization, is a major factor in the conversion of cell groups into true multicellular individuals. Once cells specialize in fitness components, they cannot survive and reproduce on their own: the group becomes indivisible and, hence, an individual.
The individuality of multicellular groups is a complex trait. Following Darwin and his approach in The Origin of Species to understanding an organ of such complexity as the human eye, we reduce the complexity to a set of evolutionary steps involving simpler traits, each advantageous by itself. In the case of the evolution of multicellular individuals, these stages might involve the formation of cell groups, the increase of cooperation within cell groups, the evolution of conflict mediators to protect the group against cheaters, the increase in group size, the specialization of cells in essential fitness components of the group, and the spatial organization of these specialized cell types.
Evidently this has happened many times. Multicellularity arose in the myxobacteria some 2,000 mya (Shimkets, 1990) and has evolved in several of the major eukaryotic groups. In the animals and plants, multicellularity evolved between 600 and 1,000 mya. Studying the factors involved in these ancient origins of multicellularity is difficult because the events are obscured by hundreds of millions of years of subsequent evolution. The protists provide a useful group for studying the stages identified above. The volvocine green algae, which by some estimates are between 38 and 70 million years old, present a nearly continuous array of differentiated stable forms representing each of the stages given above. There have been at least three independent origins of individuality based on specialization of reproductive and vegetative functions in this group (Herron and Michod, in prep.).
The volvocine green algae are flagellated, photosynthetic, facultatively sexual haploid eukaryotes with varying degrees of complexity stemming from differences in colony size, colony structure, and specialization of reproductive and vegetative cells (Fig. 7.1). This informal grouping includes the “colonial volvocines” (the families Tetrabaenaceae, Goniaceae, and Volvocaceae) and their close unicellular relatives in the genera Chlamydomonas (Fig. 7.1A) and Vitreochlamys. Colonial forms are generally small clumps or sheets of up to 32 cells such as Gonium (Fig. 7.1B) or spheres with cells arranged on the periphery, such as Eudorina (Fig. 7.1C), Pleodorina (Fig. 7.1D), and Volvox (Fig. 7.1 E and F).
The volvocine algae readily form groups by keeping the products of mitosis together through the use of extracellular materials. There are several adaptive reasons to form groups, and to increase in group size, such as to avoid predators, maintain greater homeostasis in the group, and/or to acquire new specialized cell functions. In addition, there may be a covariance effect described in Eq. 1 in which the fitness of the group
is augmented over the average fitness of member cells. This chapter takes for granted the advantages of larger group size and considers instead the associated costs of groups and how these costs may be ameliorated so as to enhance the benefits of group living. We wish to understand how groups become individuals. The central idea motivating our hypothesis is that by coping with the fitness tradeoffs and the challenges of group living, the group evolves into a new evolutionary individual.
There are several hypotheses for the evolution of cell specialization. The first involves the evolution of cooperation (versus defection). To cooperate, cells presumably must specialize at particular behaviors and functions. The evolution of costly forms of cooperation, altruism, is fundamental to evolutionary transitions, because altruism exports fitness from a lower level (the costs of altruism) to a higher level (the benefits of altruism). The evolution of cooperation sets the stage for defection, and this leads to a second kind of hypothesis for the evolution of specialized cells involving conflict mediation. If the opportunities for defectors can be mediated, enhanced cooperativity of cells will result in more harmonious functioning of the group. A variety of features of multicellular organisms can be understood as “conflict mediators,” that is, adaptations to reduce conflict and increase cooperation among cells (Michod, 2003): high kinship as a result of development from a single cell, lowered mutation rate as a result of a nucleus, self-policing of selfish cells by the immune system, parental control of cell phenotype, programmed cell death of cells depending on signals received by neighboring cells, determinate body size, and early germ soma separation. These different kinds of conflict mediators require different specialized cell types. The third hypothesis for specialization involves the advantages of division of labor and the synergism that may result when cells specialize in complementary behaviors and functions. The most basic division of labor in organisms is between reproductive and vegetative or survival-enhancing functions.
This chapter is primarily concerned with the division of labor and cooperation hypotheses. As a model system, we are considering volvocine algae cell groups that are of high kinship because they are formed clonally from a single cell. Hence, the opportunity for conflict should be low in these groups. Nevertheless, the opportunity for conflict can increase with the number of cell divisions and can depend on the type of development (e.g., rapid cell divisions, as in some volvocine algae, might not allow enough time for DNA repair). For these reasons, the conflict mediation hypothesis may help explain the early sequestration of the germ line in some volvocine lineages (Michod et al., 2003).
Evolutionary individuals must have heritable variation in fitness-related traits. The fitness of any evolutionary unit can be understood in terms of its two basic components: fecundity (reproduction) and viability
(survival). As embodied in current theory, tradeoffs between fitness components drive the evolution of diverse life-history traits in extant organisms (Stearns, 1992; Roff, 2002). In the present chapter we are primarily concerned with the cost of reproduction to viability and how this cost scales with colony size. Fitness tradeoffs gain special significance during the transition from unicellular to multicellular life for several related reasons (Michod, 2006; Michod et al., 2006): (i) fitness tradeoffs often create a covariance effect at the group level so that group fitness is augmented beyond the average fitness of component cells (see Eq. 1); (ii) fitness tradeoffs based on preexisting life-history variation provide a basis for the origin of altruistic interactions within the group (see Origin of Reproductive Altruism); and (iii) fitness tradeoffs between survival and reproduction, if of convex curvature, may select for cells specialized for reproductive and survival-related functions of the group (see Cost of Reproduction and Covariance Effect).
How do groups become individuals? Our hypothesis is that fitness tradeoffs drive the transition of a cell group into a multicellular individual through the evolution of cells specialized at reproductive and vegetative functions of the group. We have modeled this hypothesis (Michod, 2005, 2006; Michod et al., 2006) and have tested our models in two ways. We first ask whether a life-history gene present in the unicellular ancestor was coopted to be an altruistic gene in the multicellular Volvox carteri (Fig. 7.1E) (Nedelcu and Michod, 2006). By answering this question we address how an altruistic gene may originate, that is, by cooption of an existing life-history tradeoff gene. Second, we ask why reproductive altruism arises only in the larger members of the volvocine group. Our answer is that the selective pressures leading to reproductive altruism stem from the increasing cost of reproduction with increasing group size (Solari et al., 2006a,b).
ORIGIN OF REPRODUCTIVE ALTRUISM
Altruism refers to a behavior or interaction that benefits other individuals at a cost to the individual exhibiting the behavior. Altruism is widely appreciated to be the central problem of social evolution. It is also central to the reorganization of fitness during evolutionary transitions, as already mentioned, because altruism trades fitness from the lower level, the costs of altruism, to the higher level, the benefits of altruism.
In the multicellular green alga V. carteri, reproductive altruism is a property of the small flagellated somatic cells. V. carteri consists of ≈2,000 permanently biflagellated somatic cells and up to 16 nonflagellated reproductive cells. Terminal differentiation of somatic cells in V. carteri involves the expression of regA, a master regulatory gene that encodes
a transcriptional repressor (Kirk et al., 1999) thought to suppress several nuclear genes coding for chloroplast proteins (Meissner et al., 1999). Consequently, the cell growth (dependent on photosynthesis) and division (dependent on cell growth) of somatic cells are suppressed. Because they cannot divide, they do not participate directly in the offspring but contribute to the survival and reproduction of the colony through flagellar action (Short et al., 2006; Solari et al., 2006a,b). In other words, the somatic cells express an altruistic behavior, and regA [whose expression is necessary and sufficient for this behavior (Kirk et al., 1999)] is an altruistic gene. Which cells express regA and differentiate into somatic cells is determined early in development through a series of asymmetric cell divisions. The asymmetric divisions ensure that some cells (i.e., the germ-line precursors) remain above the threshold cell size associated with the expression of regA (Kirk, 1995). As with all forms of cooperation, this altruistic behavior is also susceptible to defection and selfish mutants; indeed, mutations in regA result in the somatic cells regaining reproductive abilities, which in turn results in them losing their flagellar capabilities (Kirk et al., 1987). Because motility is important for these algae (flagellar activity is required to maintain themselves in the water column at an optimum position relative to sunlight intensity), the survival and reproduction of V. carteri individuals in which such mutant somatic cells occur are negatively affected (Solari et al., 2006b).
How can an altruistic gene such as regA originate, and can its evolutionary origin be traced back to the unicellular ancestor of this group? The basic life cycle in Chlamydomonas reinhardtii (presumed to be similar to the unicellular ancestor of this group) involves a flagellated and motile vegetative stage, during which the cell grows in size, followed by absorption of the flagella and cell division to produce daughter cells. It seems reasonable to expect that life-history genes would exist in C. reinhardtii that would allocate effort to these different stages depending on environmental conditions and, in particular, allocate effort away from reproduction toward survival in conditions not promoting growth. Such a gene could become altruistic in the context of a cell group if it was turned on developmentally in some cells and if its vegetative functions also benefited the group.
Nedelcu and Michod (2006) showed that reproductive altruism (i.e., a sterile soma) in the multicellular green alga V. carteri (Fig. 7.1D) evolved via the cooption of a life-history gene whose expression in the unicellular ancestor was conditioned on an environmental cue (as an adaptive strategy to enhance survival at an immediate cost to reproduction) through shifting its expression from a temporal (environmentally induced) into a spatial (developmental) context as summarized in Fig. 7.2. The regA-like gene in C. reinhardtii (Fig. 7.1A) belongs to a diverged and structurally heterogeneous multigene family sharing a SAND-like domain (a DNA-
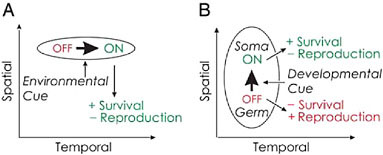
FIGURE 7.2 Change in expression of a life-history gene in space and in time. Expression of genes is indicated by the thick arrows. The effect on fitness is also specified when the gene is off and on. (A) In a unicellular individual, the gene is expressed in response to an environmental cue in a temporal context and has the effect of increasing survival while decreasing effort at reproduction. (B) This same gene is expressed in a spatial context within a multicellular individual in response to a developmental cue. The cells in which the gene is expressed increase their effort at survival and decrease their effort at reproduction. This figure was modified from Nedelcu and Michod (2006).
binding module involved in gene transcription regulation). This example is perhaps the only example of a social gene specifically associated with reproductive altruism, whose origin can be traced back to a solitary ancestor.
COST OF REPRODUCTION
Having considered how an altruistic gene might originate (by cooption of a life-history gene in a unicellular ancestor), we now ask why this happens, that is, what are the selective forces favoring soma and reproductive altruism. We wish to understand why it is that soma evolves only in the larger members of this lineage, given that in all species the groups are clonally derived from a single cell and hence of high genetic relatedness. We hypothesize that the selective pressure for soma stems from the increasing cost of reproduction to survival as colonies increase in size.
Flagellar action is an important component of survival. Volvocine algae are denser than water and need flagellar beating to avoid sinking and to find nutrients. These algae are found in quiet, standing waters of transient vernal puddles or in permanent lakes when thermal stirring stops and the lake becomes stratified (Reynolds, 1984; Kirk, 1998). For example, Volvox colonies migrate vertically several meters at night, presumably in search of higher phosphorous concentrations (Sommer and Giliwicz, 1986). In addition to motility, flagellar action provides for mixing
the surrounding medium to aid in uptake of metabolites and elimination of waste (Short et al., 2006; Solari et al., 2006a).
The first factor that leads to a cost of reproduction to flagellar action is the so-called “flagellation constraint” (Koufopanou, 1994). The flagellation constraint refers to the fact that, because of their rigid cell wall, the basal bodies cannot take the position expected for centrioles during cell division while still remaining attached to the flagella (as they do in naked green flagellates). The flagellation constraint becomes critical at the 32-cell colony size, because a flagellum may beat for up to five cell divisions without the basal bodies attached. The second factor leading to a tradeoff between reproduction and motility is that the increasing mass of the reproductive cells and embryos during reproduction decreases motility by increasing drag (Solari et al., 2006b). This increasing mass is especially noticeable in the larger species.
Large germ cells are required to form large colonies because of the unusual and likely ancestral form of cell division found in most volvocine species, known as palintomy or multiple fission. Instead of growing to twice their initial size and dividing in two, reproductive cells in palintomic species grow to many times their initial size before undergoing up to ≈13 rounds of division in rapid succession, with little or no growth between divisions. For a reproductive cell to undergo d rounds of (symmetric) division without interspersed growth, it must begin mitosis at a minimum of 2d times the initial size of the daughter cells.
Koufopanou (1994) argued for the volvocine green algae that soma evolved to keep larger colonies afloat and motile while reproductive cells divide and develop. She showed that the soma-to-reproductive-cell ratio increases with colony size and that the investment in somatic tissue increases twice as fast with colony size as does the investment in germ tissue. However, no direct evidence was given as to why a higher investment in somatic cells is needed for motility as colony size increases. Although the between-species trend is consistent with an increasing cost of reproduction with increasing group size, what selective factors operate within species?
We have modeled the hypothesis that life-history tradeoffs drive evolutionary transitions in individuality by selecting for cell specialization by considering how cells should change their allocation to reproduction and viability as colony size increases (Michod, 2006; Michod et al., 2006). Our theoretical results predict that in unicellular organisms the tradeoff curve between viability and fecundity should be concave, but as groups form and increase in size the curve should become increasingly convex (Fig. 7.3A) as a result of the increasing cost of reproduction to survival as colonies increase in size (Fig. 7.3 B and C). A central focus of Solari’s hydrodynamic work (Solari, 2005; Solari et al., 2006b) is to quantify this hypothesized increasing cost of reproduction.
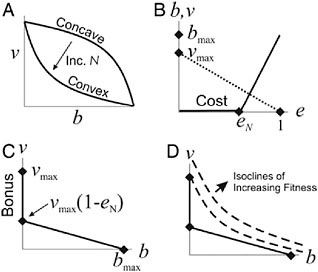
FIGURE 7.3 Fitness tradeoffs. Contribution to viability (v) on y axis and reproduction (b) on x axis. (A) A concave curve changes to a convex curve as group size increases. The piece-wise linear reproduction curve (solid line in B) with linear viability curve (dotted line in B) approximates a convex tradeoff curve (C) at the cell level. (D) Isoclines of group fitness are plotted with this convex tradeoff curve at the cell level. The reproductive effort eN in B is the cost of reproduction, which increases with group size N, and in C vmax − vmax(1 − eN) is the “bonus” of soma specialization. This bonus can be obtained only by groups. Alternatively, the bonus of specialization in soma may be viewed as the initial cost of somatic cells dedifferentiating into reproductive cells.
To illustrate how an increased cost of reproduction creates convex curvature, we construct a convex tradeoff curve between viability v and fecundity b in a piecewise linear fashion as shown in Fig. 7.3 B and C. The cost of reproduction eN is defined as the effort needed to produce an offspring colony of size N. In volvocine algae (Fig. 7.1) this effort depends on the time, energy, and resources needed to grow and divide the embryo so as to produce a daughter colony with N cells. In Fig. 7.3D the convex tradeoff curve from Fig. 7.3C is plotted with isoclines of the additional fitness to the group contributed by a newly added cell. The construction of Fig. 7.3D illustrates qualitatively a prediction of our model (Michod, 2006; Michod et al., 2006), which is that the greater the cost of reproduction (eN), the more likely the isocline touches the tradeoff curve at vmax (meaning the new cell will be soma-specialized; b = 0) as opposed to touching at an intermediate value 0 < b < bmax. Soma-specialized cells get a bonus to viability by virtue of their not paying the cost of reproduction
indicated in Fig. 7.3 B and C. This bonus can be obtained only in groups and is the basis for the synergistic effects of specialization according to our hypothesis. Alternatively, the bonus of specialization in soma may be viewed as an initial cost when somatic cells dedifferentiate into reproductive cells. Below we present evidence for this cost in terms of decreased flagellar force in regA mutants in which somatic cells have flagella for a day before dedifferentiating into reproductive cells.
Solari and colleagues developed a hydrodynamics approach using videotaping of colonies to understand motility and its determinants in volvocine algae (Solari, 2005; Solari et al., 2006b). The swimming force exerted by a single motile cell for the benefit of group motility can be calculated for different species and mutants by these techniques. Single gene mutations in life-history traits can be a powerful approach to understanding the cost of reproduction and tradeoffs between life-history traits (Reznick, 1985; Roff, 2000, 2002). In the V. carteri regA mutant, ≈235 cells change their phenotype from being somatic (S) with no reproductive function back to the ancestral state of having both somatic and reproductive functions (being flagellated first and then absorbing the flagella and reproducing). As a result of these changes in reproductive effort at the cell level, the size and motility capacities of the group change. The striking result is that as specialized somatic cells (cells with b = 0 in Fig. 7.3) prepare to exert reproductive effort (cells with b > 0), there is not only a large decrease in colony motility, but there is a large decrease in the motility force contributed by a single flagellated cell. For example, the average force exerted for group motility by a single motile cell is approximately half in the regA– mutant of what it is in wild type (4.9 × 10−8 dynes versus 8.0 × 10−8 dynes). The cost of reproduction to motility that underlies the convex nature of the fitness tradeoffs (Fig. 7.3) is real and directly measurable in these organisms and is attributable to a change in the effort exerted by single cells within the cell group. There is a caveat in that we do not know whether there are genetic differences (other than a mutation at the regA locus) between the regA− mutant strain we have obtained from the Culture Collection of Algae at the University of Texas (Austin, TX) and the wild-type strain.
In summary, comparative data indicate that reproductive effort increases with colony size and that as the investment in reproduction increases, motility declines. The regA mutant indicates that flagellar force declines if somatic cells are to dedifferentiate and start reproducing. In addition, during development, as reproductive cells increase in size, motility does not change for small species, but declines for the larger species (Solari, 2005; Solari et al., 2006b). Apparently, because the length of the flagella increases as cells increase in size, this allows the smaller
species to maintain their motility as they increase in size during development (Solari, 2005; Solari et al., 2006a).
COVARIANCE EFFECT
Tradeoffs among the contributions of cells to the fitness components of the group leads to the “covariance effect,” whereby the fitness of the group, W, is greater than the average fitness of its members, ![]() by the magnitude of the covariance among fitness components (Michod, 2006; Michod et al., 2006) as given in Eq. 1.
by the magnitude of the covariance among fitness components (Michod, 2006; Michod et al., 2006) as given in Eq. 1.
[1]
In Eq. 1, Cov[v, b] < 0 expresses a tradeoff, and ![]() The viability and fecundity of cell i (or its contribution to group viability and fecundity) are vi and bi, respectively, and i = 1,… N, where N is group size. We take fitness as the product of viability and fecundity, as is appropriate for organisms with discrete generations such as the volvocines. For groups to obtain the benefit of the covariance effect, cells must vary in their reproductive effort. As already mentioned, under a convex curvature of the tradeoff function, there is an advantage of cells specializing in different fitness components (Fig. 7.3).
The viability and fecundity of cell i (or its contribution to group viability and fecundity) are vi and bi, respectively, and i = 1,… N, where N is group size. We take fitness as the product of viability and fecundity, as is appropriate for organisms with discrete generations such as the volvocines. For groups to obtain the benefit of the covariance effect, cells must vary in their reproductive effort. As already mentioned, under a convex curvature of the tradeoff function, there is an advantage of cells specializing in different fitness components (Fig. 7.3).
Convexity or concavity of tradeoffs between fitness components is a basic issue in life-history theory (Levins, 1968; Schaffer, 1974; Michod, 1978; Reznick, 1985; Stearns, 1992; Benkman, 1993; Carriere and Roff, 1995; Takada and Nakajima, 1996; Benson and Stephens, 1996; Strohm and Linsenmair, 2000; Kisdi, 2001; Sato, 2002; Roff, 2002; Blows et al., 2004; Rueffler et al., 2004). For a convex function v(b) the second derivative is positive, and for a concave function v(b) the second derivative is negative, so if we take a particular point b* and two points equidistant below and above b*, b− and b+, then v(b−) + v(b+) > [<] 2 v(b*). If b is reproduction and v(b) is viability, then convexity of v implies there is an advantage to specializing in the two fitness components. Despite the central relevance of this issue to life-history theory, a recent review (Rueffler et al., 2004) states, “Unfortunately, there is no study known to us which has revealed the details of this curvature for any life-history tradeoff in a specific organism. However, these curvatures are central in life-history theory which indicates a major gap between theory and empirical knowledge.” We have addressed this difficult empirical problem by viewing a convex curve in a piece-wise linear fashion (Fig. 7.3) and quantifying the initial cost of reproduction to motility shown in Fig. 7.3C and as discussed in Cost of Reproduction.
The particular mathematical representation of the covariance effect given in Eq. 1 depends on additivity of fitness effects as described in
Michod et al. (2006). Additivity of fitness effects is the simplest assumption possible, and it corresponds to group selection of type 1 in the terminology of Damuth and Heisler (1988) and likely applies early in evolution as groups first start forming. For example, in the volvocine green algae, flagellar action is a main adaptive capacity underlying viability, and the forces contributed by cells to group motility are nearly additive as cells start forming groups (Roff, 2002; Michod et al., 2006). Nevertheless, the assumption of additivity of the contributions of cells to the viability of the group may be relaxed, and the general point underlying the covariance effect still holds (Michod et al., 2006).
As illustrated in Fig. 7.4, if one cell has a high reproductive effort (and hence a low viability and a low cell fitness), this may be compensated for by another cell with high viability (and hence a low fecundity and a low cell fitness) (Michod et al., 2006). Consequently, even though each of these cells by itself would have a low fitness, together they can bring a high fitness to the group, especially under conditions of convexity of the tradeoff. This kind of joint effect, whereby multiple cells may contribute more to the group than could each alone, does not require additivity (Michod et al., 2006). Also, this kind of joint effect would not be possible if group fitness were simply assumed to be the average of the cell fitnesses.
Concerning the transition from single cells to cell groups, the model predicts the following. Single cells must be generalists as far as their

FIGURE 7.4 Two cells jointly specializing in reproduction and viability. Cell i specializes in reproductive effort, bi, with less effort put into vegetative functions, vi. Cell j does the reverse. Alone they would each have low fitness, but together in a group they may have high fitness if the tradeoff between reproduction and viability is convex.
components of fitness regardless of the curvature of the tradeoff curve. However, stability of the single-cell life habit to groups requires a concave tradeoff in unicells. In cell groups, if the tradeoff remains concave, cells will not specialize, and there will be no variance to speak of and no covariance effect. However, if the tradeoff becomes convex, as a result of, for example, an increasing cost of reproduction, then cells should start specializing in viability and fecundity leading to an increased group fitness according to the covariance effect.
HOW DOES A GROUP BECOME AN INDIVIDUAL?
Let us return to the basic question asked at the beginning of the chapter: How is it that a group becomes an individual? In answering this question we assume that there is a selective benefit for forming groups and for increasing group size. We also assume there is a means of forming groups, such as by cells sticking together after cell division. According to our hypothesis, as colonies increase in size, the costs of reproduction increase and the curvature of the tradeoff between reproduction and viability goes from concave to convex. This convexity of the tradeoff curve selects for specialization in reproductive and vegetative viability-enhancing functions (germ soma specialization). As cells specialize in these essential fitness components, the fitness of the cells declines while the fitness of the group increases. The covariance effect further enhances the fitness of the group. As a result of the specialization of the cells, fitness is transferred from the cell to group level and the group becomes indivisible and an individual.
Underlying this process is high kinship among the cells, which is fundamental to, but not sufficient for, the emergence of individuality (as the volvocine algae teach us). The evolution of altruism within groups trades fitness from the lower level to the higher level, and the evolution of conflict mediation further enhances cooperation while restricting the opportunity for defecting mutants. How does a gene become altruistic? The hypothesis we have tested in the volvocine algae is that life-history genes in unicells may be coopted for reproductive altruism in the group. What are the selective factors involved, and, in particular, why doesn’t altruism originate in the smaller-sized groups? The hypothesis we have tested is that tradeoffs between reproduction and survival become increasingly convex with increasing size selecting for reproductive altruism, that is, soma. In the case of the volvocine algae, soma benefits the group both by enhancing motility and by mixing the surrounding medium allowing for more effective transport of nutrients and waste than would be possible by diffusion alone (Solari et al., 2006a; Short et al., 2006).
In this way, using the concepts of fitness, fitness reorganization, fitness tradeoffs, altruism, kin selection, life history evolution, and social evolution, we can explain a major evolutionary transition in individuality: the evolution of complex multicellular individuals from unicellular and colonial ancestors.
ACKNOWLEDGMENTS
This chapter reviews published work in which my colleagues had principal roles. Aurora Nedelcu led the experimental work on the origin of regA. She also helped me organize the lecture on which this article is based. Cristian Solari led the hydrodynamic work on motility. Yannick Viosatt had a principal role in the development of the covariance effect. I am grateful to Aurora Nedelcu, Cristian Solari, Yannick Viosatt, Matt Herron, John Kessler, and Ray Goldstein for many discussions and comments. I thank Matt Herron for his comments on the manuscript. Support from the University of Arizona College of Science is greatly appreciated.
















