Below is the uncorrected machine-read text of this chapter, intended to provide our own search engines and external engines with highly rich, chapter-representative searchable text of each book. Because it is UNCORRECTED material, please consider the following text as a useful but insufficient proxy for the authoritative book pages.
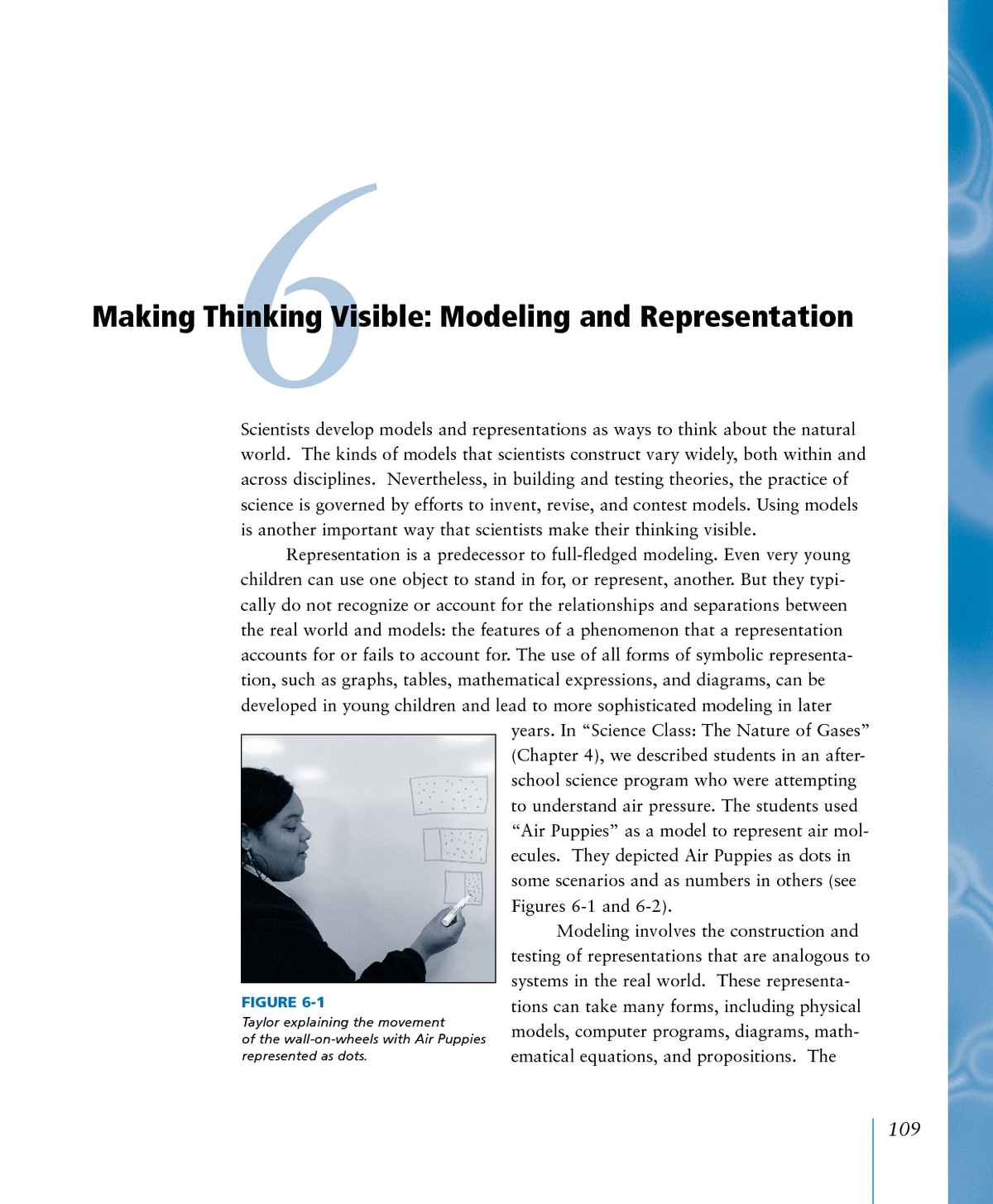
6 Making Thinking Visible: Modeling and Representation Scientists develop models and representations as ways to think about the natural world. The kinds of models that scientists construct vary widely, both within and across disciplines. Nevertheless, in building and testing theories, the practice of science is governed by efforts to invent, revise, and contest models. Using models is another important way that scientists make their thinking visible. Representation is a predecessor to full-fledged modeling. Even very young children can use one object to stand in for, or represent, another. But they typi- cally do not recognize or account for the relationships and separations between the real world and models: the features of a phenomenon that a representation accounts for or fails to account for. The use of all forms of symbolic representa- tion, such as graphs, tables, mathematical expressions, and diagrams, can be developed in young children and lead to more sophisticated modeling in later years. In âScience Class: The Nature of Gasesâ (Chapter 4), we described students in an after- school science program who were attempting to understand air pressure. The students used âAir Puppiesâ as a model to represent air mol- ecules. They depicted Air Puppies as dots in some scenarios and as numbers in others (see Figures 6-1 and 6-2). Modeling involves the construction and testing of representations that are analogous to systems in the real world. These representa- FIGURE 6-1 tions can take many forms, including physical Taylor explaining the movement of the wall-on-wheels with Air Puppies models, computer programs, diagrams, math- represented as dots. ematical equations, and propositions. The 109
objects depicted in a model, as well as their behavior and relationships to each other, represent theoretically important objects, behavior, and relationships in the natural world. Models allow scientists to summa- rize and depict the known features of a physical system and predict out- comes using these depictions. Thus, they are often important tools in the development of scientific theories. FIGURE 6-2 A key concept for students to Mitchell and Antwaune show Air Puppies in and understand is that models are not outside a bottle as numbers (100 calm and 100 excited puppies). meant to be exact copies. Instead, they are deliberate simplifications of more complex systems. This means that no model is completely accurate. For example, in modeling air molecules with Air Puppies, certain characteristics of molecules are represented, such as the fact that they move constantly without intention, and other characteristics are not, such as their being composed of hydrogen and oxygen atoms. Students need guidance in recognizing what characteristics are included in a model and how this helps fur- ther their understanding of how a system works. When first introduced to the Air Puppies model, students often ask, âDo Air Puppies breathe air? Do they sleep? Do they die?â They need to figure out which aspects of Air Puppies are useful for understanding how air molecules work. Mathematics For the past 200 years, science has moved toward increasing quantification, visu- alization, and precision. Mathematics provides scientists with another system for sharing, communicating, and understanding science concepts. Often, expressing an idea mathematically results in the discovery of new patterns or relationships that otherwise might not be seen. In the grade-level representation activities that follow, third-grade children investigating the growth of plants wondered whether the shoots (the part of the plant growing above the ground) and the roots grow at the same rate. When they plotted the growth on a coordinate graph that displayed millimeters of growth 110 Ready, Set, SCIENCE!
per day, students noticed immediately that the rates of growth were not the same. However, one student pointed out that the curves for both the roots and the shoots showed the same S-shape. This S-shape appeared again on graphs describ- ing the growth of tobacco hornworms and populations of bacteria on a plate. Students came to recognize this shape as a standard graph pattern that indicated growth. This similarity in patterns would not have been noticeable without the mathematical representation afforded by the graph. Given the importance of mathematics in understanding science, elementary school mathematics needs to go beyond arithmetic to include ideas regarding space and geometry, measurement, and data and uncertainty. Measurement, for example, is a ubiquitous part of the scientific enterprise, although its subtleties are almost always overlooked. Students are usually taught procedures for mea- suring but are rarely taught a theory of measure. Educators often overestimate childrenâs understanding of measurement, because measuring toolsâlike rul- ers and scalesâresolve many of the conceptual challenges of measurement for children. As a result, students may fail to understand that measurement entails the use of repeated constant units and that these units can be partitioned. Even upper elementary students who seem proficient at measuring lengths with rulers may believe that measuring merely entails counting the units between boundar- ies. If these students are given unconnected units (say, tiles of a constant length) and asked to demonstrate how to measure a length, some of them almost always place the units against the object being measured in such a way that the first and last tiles are lined up flush with the end of the object measured, leaving spaces among the units in between. These spaces do not trouble a student who holds this âboundary-fillingâ conception of measurement. Data Data modeling is central to a variety of scientific enterprises, including engi- neering, medicine, and natural science. Scientists build models with an acute awareness of the data that are required, and data are structured and recorded as a way of making progress in articulating a scientific model or deciding among rival models. Students are better able to understand data if as much attention is devoted to how they are generated as to their analysis. First and foremost, students need to understand that data are constructed to answer questions, not provided in a Making Thinking Visible 111
finished form by nature. Questions are what determine the types of informa- tion that will be gathered, and many aspects of data coding and structuring also depend on the questions asked. Data are inherently abstract, as they are observations that stand for con- crete events. Data may take many forms: a linear distance may be represented by a number of standard units, a video recording can stand in for an observation of human interaction, or a reading on a thermometer may represent a sensation of heat. Collection of data often requires the use of tools, and students often have a fragile grasp of the relationship between an event of interest and the operation or output of a tool used to capture data about the event. Whether that tool is a microscope, a pan balance, or a simple ruler, students often need help understand- ing the purpose of the tool and of measurement. Some students, for example, accustomed to relying on sensory observations of âfelt weight,â may find a pan balance confusing, because they do not, at first, understand the value of using one object to determine the weight of another. Data do not come with an inherent structure. Rather, a structure must be imposed on data. Scientists and students impose structure by selecting categories with which to describe and organize the data. However, young learners often fail to grasp this as evidenced in their tendency to believe that new questions can be addressed only with new data. They rarely think of querying existing data sets to explore questions that were not initially conceived when the data were collected. For example, earlier we described a biodiversity unit in which children cataloged a number of species in a woodlot adjacent to their school. The data generated in this activity could later be queried to determine the spread of a given population or which species of plants and animals tend to cluster together in certain areas of the woodlot. Finally, data are represented in various ways to see, understand, or com- municate different aspects of the phenomenon being studied. For example, a bar graph of childrenâs height may provide a quick visual sense of the range of heights. In contrast, a scatterplot of childrenâs height by childrenâs age would yield a linear relationship between height and age. An important goal for studentsâone that extends over several yearsâis to come to understand the conventions and proper- ties of different kinds of data displays. There are many different kinds of repre- sentational displays, including tables, graphs of various kinds, and distributions. Not only should students understand the procedures for generating and reading displays, but they should also be able to critique them and to grasp the advantages and disadvantages of different displays for a given purpose. 112 Ready, Set, SCIENCE!
Interpreting data often entails finding and confirming relationships in the data, and these relationships can have varying levels of complexity. Simple linear relationships are easier to spot than inverse relationships or interactions. Students may often fail to consider that more than one type of relationship may be present. For example, children investigating the health of a population of finches may wish to examine the weight of birds in the population. The weight of adult finches is likely to be a nonlinear relationship. That is, as both low weight and high weight are disadvantageous to survival, one would expect to find a number of weights in the middle, with fewer on both ends of the distribution. The desire to interpret data may lead to the use of various statistical mea- sures. These measures are a further step of abstraction beyond the objects and events originally observed. For example, understanding the mean requires an understanding of ratio. If students are merely taught to âaverageâ data in a proce- dural way, without having a well-developed sense of ratio, their performance often degrades, mistakenly, into procedures for adding and dividing that make no sense. However, with good instruction, middle and upper elementary students can learn to simultaneously consider the center and the spread of the data. Students also can generate various mathematical descriptions of error. This is particularly true in the case of measurement: they can readily grasp the relation- ships between their own participation in the act of measuring and the resulting variation in measures. Scale Models, Diagrams, and Maps Scale models, diagrams, and maps are additional examples of modeling. Scale models, such as a model of the solar system, are widely used in science education so that students can visualize objects or processes that they cannot perceive or handle directly. The ease with which students understand these models depends on the com- plexity of the relationship being communicated. Even preschoolers can under- stand scale models used to depict location in a room. Elementary school students can look beyond the appearance of a model to investigate the way it functions. However, extremely large and small-scale models often pose serious challenges for students. For example, middle school students may struggle to work out the posi- tional relationships of the earth, the sun, and the moon, which involves not only reconciling different perspectives (what one sees standing on the earth, what one Making Thinking Visible 113
would see from a hypothetical point in space) but also visualizing how these per- spectives would change over days and months. Students are often expected to read or produce diagrams and integrate information from the diagram with accompanying text. Understanding dia- grams seems to depend less on a studentâs problem-solving abilities than on the specific design and content of the diagram. Diagrams can be difficult to understand for many reasons. Sometimes the desired information is missing. Sometimes a diagram does not appear in a familiar or recognizable context. And sometimes features of a diagram can create confusion. For example, the common misconception that the earth is closer to the sun in the summer than in the winter may be due, in part, to the fact that two-dimensional representations of the three-dimensional orbit make it appear as if the earth is indeed closer to the sun at some points than at others. Studentsâ understanding of maps can be particularly challenging, because maps preserve some characteristics of the place being representedâfor instance, relative position and distanceâbut may omit or alter features of the actual landscape. Recall the mapping done by Mr. Walkerâs class in the case study on biodiversity in Chapter 2, in which the students learned to develop a more sys- tematic plan for mapping the distribution and density of common species. Young children especially have a much easier time representing objects than representing large-scale space. Students may also struggle with orientation, perspective (the traditional birdâs eye view), and mathematical descriptions of space, such as polar coordinate representations. Modeling and Learning Progressions In a study involving biological growth, Richard Lehrer and Leona Schauble observed characteristic shifts in the understanding of modeling over the span of the elementary school grades.1 They developed a learning progression that emphasized different and increasingly complex ideas in different grade bands. Each had a different curriculum and tasks: ⢠Early elementary: Growth of flowering bulbs: A focus on difference ⢠Middle elementary: Growth of Wisconsin Fast Plants2: A focus on ratio ⢠Late elementary: Growth of population: A focus on distribution 114 Ready, Set, SCIENCE!
They observed that primary grade studentsâ initial representations of growth were typically focused on endpoints, for example: âHow tall do plants grow?â Studentsâ questions about plant height led to related concerns about identifying the attributes of a plant that could best represent height and how those attributes should be measured. As one might expect, studentsâ resolutions to these problems varied by grade. First-Grade Representations First graders represented the heights of plants grown from flowering bulbs, using green paper strips to depict the plant stems at different points in the growth cycle (see Figure 6-3). Consistent with the claim that young children try to create mod- els that closely resemble real or known objects, the students at first insisted that FIGURE 6-3 A display with the paper strips be adorned with flowers. detailed drawings However, as the teacher repeatedly focused studentsâ attention on succes- of individual plants that include flowers sive differences in the lengths of the strips, students began to make the conceptual and colors. transition from thinking of the strips as âpresentingâ height to ârepresentingâ height (see Figure 6-4). Reasoning about changes in the height differences of the FIGURE 6-4 strips, students identified times when their plants grew âfasterâ and âslower.â Displays of plant height depicted in Their study of the plant heights was firmly grounded in prior discussions about bar graphs. what counted as âtallâ and how to measure it reliably. Making Thinking Visible 115
Third-Grade Representations In the third grade, children integrated math into their representations of Wisconsin Fast Plants in a variety of ways. They developed âpressed plantâ silhouettes that recorded changes in plant morphology over time, coordinate graphs that related plant height and time, sequences of rectangles representing the relationship between plant height and canopy âwidth,â and various three-dimensional forms to capture changes in plant volume. As the diversity in types of studentsâ representations increased, a new question emerged: Was the growth of roots and shoots the same or different? Comparing the height and depth of roots and shoots, students noticed that, at any point in a plantâs life cycle, the differences in measurement were apparent. However, they also noted that graphs displaying the growth of roots and shoots were characterized by similar shapes: an S-shaped logistic curve (see Figure 6-5). Finding similarities in the shape of data describing roots and shoots but not the measurements of roots and shoots, students began to wonder about the sig- nificance of the similarity they observed. Why would the growth of two different FIGURE 6-5 A display of plant height over time depicted in an S-shaped curve. 116 Ready, Set, SCIENCE!
plant parts take the same form on the graph? When was the growth of the roots and shoots the fastest, and what was the functional significance of those periods of rapid growth? Students became competent at using a variety of representational forms as models. For example, students noted that growth over time x,y-coordinate graphs of two different plants looked similar in that they were equally âsteep.â Yet the graphs actually represented different rates of growth, because the students who generated the graphs used different scales to represent the height of their plants. The discovery that graphs might look the same and yet represent different rates of growth influenced the studentsâ interpretations of other graphs in this and other contexts throughout the year. Fifth-Grade Representations In the fifth grade, children again investigated growth, this time in tobacco horn- worms (Manduca), but their mathematical resources now included ideas about distribution and sample. Students explored relationships between growth factors: for example, different food sources and the relative dispersion of characteristics in the population at different points in the life cycle of the hornworms. Questions posed by the fifth graders focused on the diversity of charac- teristics within populationsâfor example, length, circumference, weight, and days to pupationârather than simply shifts in central tendencies of attributes (see Figure 6-6 on page 120). As the studentsâ ability to use different forms of representation grew, so, too, did their consideration of what might be worthy of investigation. Shifts in Understanding In sum, over the span of the elementary school grades, these researchers observed characteristic shifts from an early emphasis on models that used literal depic- tion toward representations that were progressively more symbolic in charac- ter. Increased competence in using a wider range of representational types both accompanied and helped promote conceptual change. As students developed and used new mathematical means for character- izing growth, they understood biological change in increasingly dynamic ways. For example, once students understood the mathematics of changing ratios, they began to conceive of growth not as a simple linear increase but as a patterned rate of change. These shifts in both conceptual understanding and forms of Making Thinking Visible 117
written or graphic representation appeared to support each other, opening up new paths of inquiry. Students noticed similarities and differences among graphs and wondered whether plant growth was similar to animal growth and whether the growth of yeast and bacteria on a Petri dish was similar to that of a single plant. Students studying the growth of such organisms as plants, tobacco hornworms, and populations of bacteria noted that when they graphed changes in heights over a life span, all the organisms studied produced an S-shaped curve on the graph. However, making this connection required a prior understanding of a Cartesian coordinate system. In this case and in others, explanatory models and data mod- els worked together to further conceptual development. At the same time, growing understanding of concepts led to increased sophistication and diversity of repre- sentational resources. Current instruction often underestimates the difficulty of connecting repre- sentations with reasoning about the scientific phenomena they represent. Students need support in both interpreting and creating data representations that carry meaning. Students learn to use representations that are progressively more sym- bolic and mathematically powerful. Teachers need to encourage this pro- cess over multiple grades. Letâs take a closer look at how children develop scientific represen- tations. In the following case, also taken from the work of Lehrer and Schauble, we examine a group of fifth graders working on an investigation of plant growth. They are challenged to develop representations of their data in order to reach particular goals in com- municating. 118 Ready, Set, SCIENCE!
Science Class REPRESENTING DATA3 Students need opportunities to build models and representations that suit particular explanatory and communica- tive purposes. They need experience refining and improving models and representations, experience that can be facilitated by critically examining the qualities of multiple models or representations for a given purpose. In the following example we visit a fifth-grade classroom in which students are studying species variation. Having tracked the growth of Wisconsin Fast Plants over a period of 19 days, they are grappling with the best way to rep- resent their data. Hubert Rohling, the teacher, has posted a list of unordered measures that the students had taken over the previous 18 days on chart paper at the front of the class. He has asked them to consider two questions: (1) how they might organize the data in a way that would help them consider typical height on the 19th day and (2) how to characterize how spread out the heights were on this day. He chose to have the students focus on these qualities of their representation in order to draw their attention to critical aspects of representing data sets. Mr. Rohling understood that his students would need to grapple with how best to portray data and to practice doing so as a purposeful activity. Rather than assigning children particular data displays to use in capturing data, he asked them to invent displays. He introduced additional uncertainty into the assignment by asking students to identify typical values. Often the approach to learning about typical values is to teach children different measures of central tendency and to assign children to calculate means, or identify the modal or median values in a data set. Mr. Rohlingâs interest, however, was to push children to wrestle with the notion of typicality and articulate their understanding through creating and critiquing data displays. In the process students would be forced to grapple with the value of maintaining regular intervals between data points (thus providing a visual cue as to the quantitative relationship among points) and sampling distribution. (What aspect of the data provides a fair sense of the overall shape of the data set?) Students would confront the same kinds of problems that scientists do in the course of their work. They must find meaningful ways to organize information to reveal particular characteristics of the data. The students had previously been assigned to seven working teams of three to four students each. The students in each group worked to construct a data display that they believed would support answers to Mr. Rohlingâs two questions. Mr. Rohling encouraged each group to come up with its own way to arrange the data, explaining that it was important that the display, standing alone, make apparent the answer to the two questions about typicality and spread of heights. The studentsâ solutions were surprisingly varied. From the seven groups, five substantively different representa- tional designs were produced. Over the next two days, students debated the advantages and trade-offs of their representational choices; their preferences shifted as the discussion unfolded. To encourage broad participation in critical discussion of displays, Mr. Rohling assigned pairs of students to present displays that their classmates had developed. And following this he facilitated discussions which drew in display authors, presenters, and other class- mates. Despite the opportunity to exchange ideas with their peers, students did not easily or simply adopt conven- tions suggested by others. Instead, there was a long process of negotiation, tuning, and eventually convergence toward a shared way of inscribing what students came to refer to as the shape of the data. Making Thinking Visible 119
The first display discussed is shown in Figure 6-6. One of the students, Will, and his team- mate presented this graph on large, easel- sized graph paper. As the figure shows, the authors first developed a scale (along the left side of the graph) to include all the observed heights of the plants. Then they simply drew lines to that scale, representing the height of each plant, ordered from the shortest to the tallest. As the class considered this display, Will tried to explain how this graph could be used to answer Question 1: âWhat is a typical height on Day 19?â Will: âThe tops of the lines represent height, and you have to see which lines stop and go along on one level. Itâs . . . itâs the same number.â [He points toward a space in the middle of the graph where all the lines appear to be about the same height.] Mr. Rohling: âSo youâre looking for a flat FIGURE 6-6 line to tell you what typical is?â A data display representing individual specimen height with a vertical line. Will: âYes, then you can tell how many of those there are.â difficult to read, especially from the back of the room. Will volunteered that the authors might Mr. Rohling: âWhat about Question 2: How consider alternating colors for different values, to spread out are the plants on Day 19?â make it easier to discern small changes in contigu- Will: âYou can look at the graph and see that ous values. it starts low down here on the left and goes The authors of the second display (Figure 6-7) up on the right.â simply ordered the values from lowest to high- est and then wrote them along the bottom of the Mr. Rohling: âIf the data werenât spread out, paper, stacking the values that occurred multiple what would it look like?â times. The chart makers apparently ran out of room Will: âOne flat horizontal line.â along the bottom of the page and, to avoid start- ing over, placed the remaining four values (200, 205, This exchange shows that Will understood that 212, 255) on the upper left, surrounded by a box. âplateausâ on the graph denote clumps in the data. Although the values are separated by commas, this However, he went on to admit that the graph was display, like the display previously discussed, fails to 120 Ready, Set, SCIENCE!
preserve interval. That is, the authors did not use Matt: âI think this part on the top shouldnât spaces to indicate missing values. Therefore, linear be there [pointing to the âleftoverâ num- distance does not accurately represent spread in the bers in the box]. Itâs kind of confusing. data. Keith and Matt interpreted this graph. Those numbers on the top, they ran out of room.â Keith: âThe typical number is, like, the one that goes higher than the others. You can just The third display (Figure 6-8) presented values tell. The most common one is the highest col- stacked in âbinsâ of 10. This display preserves each umn [the typical value.] The next question, case value as well as the interval (the bin) as each for how spread out the data is . . . we just plant height is written above its âbinâ in ascending took the lowest number here . . . it was 30 . . . order. This form of display was used the previous year and subtracted it from 255. We got 225.â in a rocket investigation, and the students may have had at least a vague memory of its form. Mr. Rohling: âSo does the graph itself help Looking at the display, Julia and Angelique you see that? Or do you have to do some- identified the mode as the âtypical value,â pointing thing with the numbers?â out that most of the values were in the 160s col- Keith: âYou can tell the graph is pretty umn. However, one student found the graph con- spread out from 30 to 255.â [He sweeps his fusing. She asked, âHow come itâs all grouped by hand across the line.] tens?â Julia replied, âThatâs just how they did it.â Instead of letting this answer stand, Mr. Rohling Mr. Rohling: âWhat could you do to show pushed the discussion further. He wanted the stu- typical and spread better?â dents to think about why âbinningâ the values might produce different views (shapes of data) of typicality and spread. To raise these issues, he asked the class to think about a contrast, between the simple, ordered list (Figure 6-7) and the display currently under consideration (Figure 6-8). Of Figure 6-7, he asked, âHow did this group bin them?â A student replied, âOne value per bin.â Another student asked, referring to Figure 6-8, âWhy did you select bins of 10?â Tanner and Erica, the authors of the graph, explained their reasoning: FIGURE 6-7 A display featuring ordered values of plant heights. Making Thinking Visible 121
Tanner: âWe wanted to make our numbers bigger and easier to see, so we didnât want to waste a bunch of room.â Erica: âWe also thought it would be easier to answer the two questions this way.â Mr. Rohling: âSo youâre saying that binning them helps you see whatâs typical?â Erica: âYes, and how spread out they are.â Mr. Rohling: âHow does binning help you do that?â Tanner: âTypical is from 160 to 169. Itâs not that there is a typical FIGURE 6-8 number; itâs the typical group, I A data display using âbinsâ of ten. would say. This idea of a typical group or typical region would come to play an increasingly central role over the subse- quent weeks of instruction, especially as the class began to discuss sampling. For the time being, Mr. Rohling decided to go on to the next display (Figure 6-9). This display listed values in ascending order from left to right, starting at the top left and moving down the page in rows, with repeated values stacked together. Katie and Greg, the present- ers, noted that the authors had writ- ten their proposed typical value on the lower right of the display and that they had also marked out the 160s in their FIGURE 6-9 display, presumably to indicate that these were the A data display with rows of ascending values and repeated values selected as typical. However, Katie and Greg values stacked. 122 Ready, Set, SCIENCE!
thought that this graph made it difficult to answer Mr. Rohling: âSo, Julia, do you think if I wrote the question about âspread.â the number 555 right here [he appends the value 555 immediately at the end of the Katie: âThis graph is a little more clumped ordered list on Figure 6-7], it would be the eas- up than the others (Figures 6-6 and 6-8 for iest graph to see that this has a lot of spread?â example). Itâs not in a line, so itâs a little harder to see. They were doing it in rows, but Katie: âI think probably this graph [Figure they did columns, too. That was kind of hard 6-8] would probably be better for spread to figure out.â because they still leave the spaces there, even if thereâs nothing there. So you can really see Mr. Rohling: âSo youâre saying if you just had how spread out it is. You can see how much to use the graph data. . .â space there is.â Keith: âWeâd be way off.â Mr. Rohling: âYouâre saying if it was 555, Mr. Rohling then returned to the previous dis- weâd figure 555 would be out here? [He plays to juxtapose two different approaches to indicates a space way off the right edge of spread, one focusing on ordered cases (Figure 6-7) the graph.] Then the graph would actually and the other on interval (Figure 6-8). He employed look like itâs spread? What helps you see the an imagined value (555) to highlight the difference spread, then?â between interval and order. Isaac: âNot just the numbers that we actu- Mr. Rohling: âIâm wondering which graph ally measured that are in between, but would show the spread better? Letâs ignore empty spaces in all the numbers that are in 255 for a minute [the highest value on both between.â graphs] and assume that the highest value is At this point, the students appeared to reach more like 555 [he opens his hands wider]. Does agreement that if a display is to show the spread in that feel quite a bit different than 255? If we the data, it is necessary to scale the graph in a way include that number, that would become a that preserves intervals, even intervals for which no much bigger spread. So letâs pretend that the values have been observed. Although few of the high value is 555. Which graph would help us original displays met this criterion, all of the displays see that itâs more spread out? What about the made after the discussion did so. one with the bins [Figure 6-8]? Is there a graph Other displays were also presented (see Figures up there that would help?â 6-10 and 6-11). And, as the discussion progressed, it Julia: âI think this one [Figure 6-9] might be was clear that there were two competing value sys- harder to read from far away. They put the tems in the air that were driving the studentsâ display data in a square instead of a line.â preferences. On one hand, studentsâ own designs or those made by close friends were especially favored, At this point, one of the authors of that graph and novelty and creativity were also highly prized. protested, âWe wanted people to be able to see the For example, as the presenters explained Figure 6-10, numbers. If theyâre small, theyâre hard to read. If we murmurs of âOh, thatâs cool!â and âYou guys are so had more paper, weâd have done it on a line.â Making Thinking Visible 123
cool!â were heard from about half the class. On the other hand, about half the students expressed concerns that the âcoolâ solution did not seem to provide an illustration of either typicality or spread. The display depict- ed in Figure 6-11 was deemed even âcoolerâ but, as more than one classmate noted, did not surrender its design logic readily. It took two full days of discussion before students finally surrendered their focus on novelty of design and gravitated instead toward criteria favoring clarity of the mathematical ideas. FIGURE 6-10 A data display on a two- dimensional coordinate grid. FIGURE 6-11 A data display showing median at the apex of a pyramid. 124 Ready, Set, SCIENCE!
As this case illustrates, elementary school students can create representations that have clear communicative features. The representations themselves and the rich discussions they support offer an important window into how students are thinking about representation and about the phenomena being studied. Generating multiple representations and critiquing their utility for a particular goal can com- pel elementary school students to develop a clearer sense of the considerations that go into developing representations. In addition to supporting studentsâ skill at creating and using representation, modeling data through displays is fertile ground for advancing all four strands of science learning. In the case above, for example, children developed their substan- tive understanding of plant growth and population as they discussed and critiqued the data representations (Strand 1). They developed facility with graphing and making sense of data as they constructed representations of plant heights that conveyed information about the data spread and typical values (Strand 2). They embraced science as a dynamic undertaking and reflected on the adequacy of their representations. Over time their ideas changedâfavoring âcoolâ displays slowly gave way to favoring displays that communicated clearly. Students whose previ- ous displays did not retain intervals used intervals in subsequent displays, building on the cumulative insight of the group (Strand 3). Finally, their arguments and approaches to revising their models were governed by the goals and norms of sci- ence. As they analyzed and discussed the data displays, they practiced scientific norms by critically appraising each otherâs displays and explicitly reasoning about how well the displays accomplished the intended communicative goals (Strand 4). Importantly, learning in each of the strands did not take place in isolation. Rather, advances in one strand supported and were catalyzed by advances in the other strands. This underscores a key point established in previous chapters: sci- ence is complex and learning science takes time and practice. The sophistication of students in the case above is the result of engaging in a rich investigative task, but also of many months and even years of science instruction that supported their knowledge and skill across all four strands. Some important generalizations can be drawn from the examples of represen- tation discussed in this chapter. Graphs, tables, computer-based tools, and math- ematical expressions are examples of important symbolic and communication tools used in modeling. Scientists, as well as students of science, use representations to Making Thinking Visible 125
convey complex ideas, patterns, trends, or proposed explanations of phenomena in compressed, accessible formats. These tools require expertise to understand and use. Teachers can help students reflect on the features and purposes of rep- resentations by asking them to generate and critique their own representational solutions to problems, by encouraging them to interpret the representations developed by other students, and by asking them to consider what a representa- tion shows and hides so that they come to understand representational choices as trade-offs. Although working with representations poses challenges for learners, it also can help bridge between the knowledge and skills they bring to the classroom and more sophisticated scientific practices. For Further Reading Lehrer, R., and Schauble, L. (2004). Modeling natural variation through distribution. American Educational Research Journal, 41(3), 635-679. McNeill, K.L., Lizotte, D.J., Krajcik, J., and Marx, R.W. (2006). Supporting studentsâ construction of scientific explanations by fading scaffolds in instructional materials. Journal of the Learning Sciences, 15(2), 153-191. National Research Council. (2007). Teaching science as practice. Chapter 9 in Committee on Science Learning, Kindergarten Through Eighth Grade, Taking science to school: Learning and teaching science in grades K-8 (pp. 251-295). R.A. Duschl, H.A. Schweingruber, and A.W. Shouse (Eds.). Center for Education, Division of Behavioral and Social Sciences and Education. Washington, DC: The National Academies Press. 126 Ready, Set, SCIENCE!