Consider the effect on this predictive value of enrolling one additional person in a watch list of length n, assuming the pattern of presentations to the list is fixed at proportion p of previous enrollees. In addition to comparisons with the slightly shorter previous list, the presenter is now compared to the new enrollee. This cannot increase and may decrease P(true match|enrolled), because each comparison offers an additional opportunity for an enrolled presenter to be erroneously matched with the wrong enrollee by matching more closely with someone else’s stored data than with his or her own. Similarly, both denominator terms cannot decrease and may increase, because the new comparison offers any presenter an oppportunity of falsely matching with an extra enrollee.

Hence the ratio, PPV(p), cannot increase and may decrease with watch-list length. Using the subscript to indicate watch-list length, PPV_n+1(p) ≤ PPV_n(p) for any specific p. Thus, the posterior means for the two list sizes over the distribution F(p) must hold the same relationship:

These expectations are the marginal probabilities that a claimed match is correct for the different list sizes, so increasing list length by one enrollee cannot increase and may be expected to decrease the confidence warranted by a watch-list identification. Iterating this point shows that lengthening the list by any amount must have the same implications. However, this argument depends on decoupling the presentation distribution F(p) from enrollee characteristics. In a finite population setting, where increasing enrollment increases p, a much more complicated argument might be required, with the outcome dependent on the specifics of functional relationships. A general argument that would work in such a setting is not obvious.

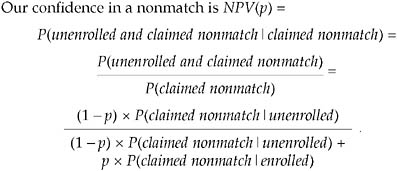
As noted above, increasing watch-list size by one new enrollment without changing p offers an additional opportunity for each unenrolled presenter to falsely match. Thus, P(claimed nonmatch|unenrolled) can-