3
Physics Education Research
as a Foundation for
Improving Education
Many of the advances in undergraduate physics education that are highlighted in Chapter 2 were the direct result of physics education research (PER). This chapter places the studies mentioned earlier into a broader context of scholarship. It must be emphasized that teaching is a complex process in which the intuition, experience, and enthusiasm of individual instructors play an important role that is not diminished by findings from research. Instead, systematic investigations of how students learn provide instructors with essential information and tools, much as fundamental research in the health sciences is a critical component of medical care but is not a replacement for clinical judgment, compassion, and dedication. PER can thus be thought of as one of the pillars that support physics education: not sufficient on its own, but necessary for promoting effectiveness.
Since the field of PER emerged in the 1970s, the PER community has made significant advances in understanding how students learn physics. Several hundred researchers are now tackling problems with both immediate and long-term implications for undergraduate physics education. As part of the preparation for the recent National Research Council (NRC) report Discipline-Based Education Research: Understanding and Improving Learning in Undergraduate Science and Engineering (2012), both a history of PER (Cummings, 2010) and an extensive synthesis of results (Docktor and Mestre, 2010) were prepared. The NRC report also discusses the role that PER has played in advancing education research as a scholarly pursuit for academic scientists in other disciplines.
The paper prepared by Docktor and Mestre (2010) includes 450 unique citations. A recent review by Meltzer and Thornton (2012) of findings related to active
learning in undergraduate physics includes 173 unique citations. These reports are not replicated or summarized here. Below, a very brief overview of PER sets the stage for a discussion of key findings and current priorities in six major areas of research. Taken together, these items constitute a research agenda that responds to pressing needs in undergraduate physics education and also encourages foundational research that may drive improvements in courses and programs in the future.
A BRIEF OVERVIEW OF THE FIELD OF PHYSICS EDUCATION RESEARCH
As a field of investigation, PER has grown significantly since the 1970s, a decade in which the first Ph.D. degrees in physics in the United States were awarded for research on learning and teaching of physics. Since that time, PER has produced results that provide a foundation for improving both the efficiency and the effectiveness of student learning. Perhaps the most important finding of the past four decades of PER is that a variety of specific teaching methods can lead to improved student understanding, compared to the frequently used lecture method.
The effectiveness of different teaching methods has often been established by measuring what students are able to do at different points in instruction; for example, giving students pretests and post-tests before and after a specific intervention or an entire course. The pretests and post-tests typically consist of questions that require students to apply what they have been taught to situations that are not exactly the same as any they have seen before and that are not susceptible to formula manipulation.
Methods for assessing the degree to which changes to instruction bring about improvements in student understanding are part of a process of applied research that leads to the development of methods and materials that can be adopted by faculty at other institutions. Typically, initial design is based on a set of principles such as those listed in Chapter 2, including knowledge of common student ideas in the topic area. Successive refinements are suggested by post-test results, classroom observations, and further in-depth research (e.g., interviews). Eventually, testing takes place at other institutions to ensure that the methods or materials are transportable and to determine the conditions needed for effective implementation. While this is not by any means the only framework employed in PER, it is emphasized here because many of the innovative methods and materials mentioned in this document resulted from some variation on this procedure.
A broad range of student audiences have benefited from the improvements in instruction that have resulted from research-driven development so far. The majority have been students in introductory calculus and algebra-based courses. Research-based strategies for these courses are too numerous and too varied to summarize here; a brief discussion is presented in Chapter 2, and a good recent review can be found in Meltzer and Thornton (2012).
Similar methods have been used to achieve improvements in upper-division courses on electricity and magnetism (Chasteen et al., 2011; Pollock, 2009), classical mechanics (Ambrose, 2004), thermal physics (Cochran and Heron, 2006; Meltzer, 2004), and quantum mechanics (Singh, 2001; Cataloglu and Robinett, 2002; Zollman et al., 2002). Courses for elementary and secondary teachers have also been addressed (Etkina, 2010; McDermott et al., 2006, 1996; Goldberg et al., 2008; Zollman, 1990, 1996).
One of the difficulties of measuring improvements in instruction is that physics faculty and physics courses represent a variety of instructional goals that are rarely carefully articulated. Developing student understanding of physics concepts and developing student problem-solving abilities are often the top-stated goals for physics courses. Yet, the precise articulation and pursuit of these goals in terms of measurable student outcomes is rarely done.
Additional information on PER and research-based instructional methods can be found at PER Central (http://www.compadre.org/per/) and the PER User’s Guide (http://perusersguide.org). Box 3.1 lists some short books that include additional information on using research-based methods in instruction. A series of articles published in the American Journal of Physics by winners of the AAPT’s Oersted Medal and Millikan Award provides overviews of research and the development of research-based instructional materials and methods and includes articles by Lillian McDermott, Edward Redish, Priscilla Laws, Fred Goldberg, Frederick Reif, Carl Wieman, and Alan Van Heuvelen, among others.
KEY FINDINGS FROM PHYSICS EDUCATION RESEARCH
One of the most robust findings from PER is that traditional, lecture-style introductory courses have little long-lasting effect on students’ erroneous notions about the physical world (McDermott, 1991; Hake, 1998). This can be assessed by asking students simple questions such as making a prediction or drawing an inference about a physical situation. Memorization of formulas or even a relatively high level of skill at solving traditional end-of-chapter problems is inadequate for reasoning in these situations. Further, research has determined that students’ responses to such questions are typically not random and idiosyncratic. Instead, a small number of erroneous reasoning patterns are documented among a large variety of students. For example, when asked about the forces acting on a coin tossed straight up (and told to neglect air resistance), many students cite “a steadily decreasing upward force,” possibly reasoning that upward motion implies an upward force and a decreasing velocity implies a decreasing force (Clement, 1982). Common student ideas such as this have been identified in almost all areas of physics.
Early research seeking to identify such ideas typically involved one-on-one interviews in which students were asked to apply the physics that they had learned
BOX 3.1
Important Initial Resources
Practical applications of physics education research (PER) are numerous in the literature. Authors of a few short books have collected a variety of research-based techniques and discussed how they can be used in the physics classroom. The books listed below provide applications of PER as well as references to many of the findings of the field.
Knight, R., 2002. Five Easy Lessons: Strategies for Successful Physics Teaching. Addison-Wesley, San Francisco, Calif.
Experienced physics instructor and textbook author Knight discusses some of the core findings of physics education research that directly apply to teaching an introductory quantitative physics course. After a brief overview of some general instructional principles, the rest of the book contains Knight’s recommendations for teaching each of the core content areas of an introductory physics course. His recommendations are based both on the available research literature as well as his extensive teaching experience. Sample activities and homework and test problems are provided.
Mazur, E., 1997. Peer Instruction: A User’s Manual. Prentice Hall, Upper Saddle River, N.J.
Peer Instruction and related techniques are widely known and use research-based instructional strategy for teaching introductory physics. In this book, peer instruction developer Mazur describes the philosophy behind the technique as well as detailed instructions for its implementation. Much of the book contains ConcepTests (multiple-choice conceptual questions to be used during lecture) and conceptually oriented exam questions that can be used by instructors. Mazur uses peer instruction with electronic student response systems (clickers); others have successfully used the strategy with flashcards (Meltzer and Manivannan, 1996).
Redish, E.F., 2003. Teaching Physics with the Physics Suite. John Wiley and Sons, Hoboken, N.J.
Experienced physics instructor and physics education researcher Redish discusses a variety of research-based tools for improving teaching and learning in introductory physics. After summarizing some of the relevant findings from cognitive science, the majority of the book is a discussion about what is involved in the implementation of 11 research-based instructional strategies. Assessing instructional effectiveness and assessing student learning are emphasized.
to a new situation, often supplemented with short written problems that require explanation. (See, for example, Goldberg and McDermott, 1987.) Researchers continue to use this method, along with videotaped group discussions among students or between students and a teacher, to draw inferences about how students are thinking about physics. However, the development of multiple-choice, research-validated conceptual evaluation instruments in the 1980s and 1990s allowed the rapid gathering of data from different colleges and universities, helping to establish the generality of earlier results. The most widely known multiple-choice instrument, the Force Concept Inventory (FCI), was developed based on students’ answers to free-response questions (Hestenes et al., 1992). An example
of the use of the FCI in assessment was presented in Chapter 2. Assessments have been developed in many other areas in physics, such as electricity and magnetism (Ding et al., 2006; Maloney et al., 2001), graphical interpretation (Beichner, 1994), and quantum mechanics (Zhu and Singh, 2012; McKagan et al., 2010; Cataloglu and Robinett, 2002).
Many physics instructors were (and still are) shocked to find that students can obtain high scores in their courses but, when faced with certain tasks (such as the coin-toss problem mentioned above), still express ideas that directly contradict what they have been taught. These results have been replicated in courses taught by experienced professors at a broad spectrum of institutions, including community colleges, large public research universities, and selective private colleges (McDermott and Redish, 1999; Duit, 2009) as well as at a range of universities outside the United States. (See, for example, Schecker and Gerdes, 1999; Duprez and Méheut, 2003; Hartmann and Niedderer, 2005; Bao et al., 2009a, 2009b; and Duit, 2009.) Moreover, similar results have been found in nearly every area of physics taught at the introductory level.
Taken together, the results of systematic research indicate that many students’ success in introductory courses reflects the development of procedural skills with algorithmic methods without an understanding of the physics that is the foundation for those methods, the derivation of which they do not understand. Why do carefully prepared and delivered lectures, well-written textbooks, and experiments that validate the laws of physics lead to such disappointing results? The evidence suggests that in general the fault does not lie solely with poor mathematical preparation or poor study habits. Instead, the findings point to an intrinsic weakness in the methods of instruction employed in typical physics courses.
Significant improvement is possible. The second robust finding from PER highlighted here is that student understanding and performance can be greatly enhanced with approaches to learning that are more similar to the way scientists learn and do science: (1) students must be actively engaged in their own learning (an engagement that is often facilitated by classroom interactions with peers and instructors) and (2) instruction must attend to students’ own reasoning (both their preexisting ideas about how the world works and those that develop as they try to integrate new ideas during instruction).
The validity of these two principles has been established in a variety of studies (see Meltzer and Thornton, 2012, for a comprehensive collection), and they are consistent with more general findings from cognitive science studies, as discussed in the NRC report How People Learn (2000). Redish and Steinberg’s (1999) study also showed that students who completed courses using one of two different research-based curricula increased their scores on the FCI significantly more than students in traditional classes. (See Figure 3.1.) Classes that used Tutorials in Introductory Physics (McDermott et al., 1998, 2002) to supplement instruction in lecture and
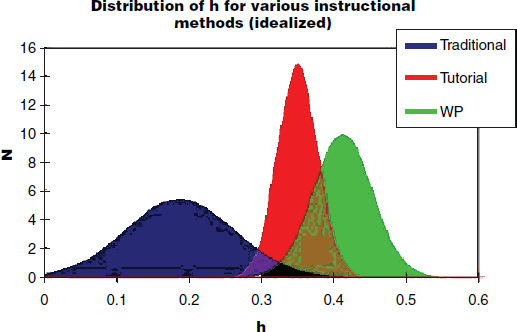
FIGURE 3.1 Gaussian fit to histograms of Force Concept Inventory gains in traditionally taught classes, in classes using UW Tutorials (McDermott et al., 1991, 2002; Redish and Steinberg, 1999) and cooperative group problem solving (GPS) techniques (Heller et al., 1992; Heller and Hollabaugh, 1992), and in classes using Workshop Physics (Laws, 1991, 1997). A total of eight institutions are represented. The horizontal axis represents normalized learning gains (“h” = [post-test score – pretest score]/[total possible score – pretest score]). The gain is higher in research-based learning environments than it is in traditional learning environments. SOURCE: Reprinted with permission from E.F. Redish and R.N. Steinberg, Teaching physics: Figuring out what works, Physics Today 52:24-30, 1999, Figure 4b, Copyright 1999, American Institute of Physics.
laboratories had greater learning gains than the traditionally taught course. Classes that used Workshop Physics (Laws, 1991, 1997), a studio-style course in which all instruction takes place in a laboratory-like setting, had still greater gains. Pollock (2012) reported learning gains from 8 years of introductory courses taught by a variety of instructors who used active engagement methods. Similar results have been found in a large study of introductory astronomy courses (Prather et al., 2009).
PER-based instructional methods have been tested extensively by faculty at institutions other than those where initial development took place. For example, Pollock and Finkelstein (2008) compared scores on conceptual questions on midterm exams for courses using Tutorials in Introductory Physics (McDermott et al., 1996, 2002) and replicated results from the University of Washington, where the materials were developed (Box 3.2). Francis et al. (1998) found that the same
BOX 3.2
Tutorials in Introductory Physics
Tutorials in Introductory Physics, developed at the University of Washington, is a set of research-based instructional materials that focus on active participation of students in the learning of physics in introductory classes (McDermott et al., 1998, 2002). In one study of the effectiveness of these instructional materials, student scores on conceptual questions in midterm exams of introductory physics classes at the University of Colorado (CU) and University of Washington (UW) were compared. Figure 3.2.1 shows the percentage of correct answers for students at the two universities who used the tutorials and those who did not. The students who used tutorials performed about equally well at both universities and significantly better than those students who had instruction that was not based on physics education research.
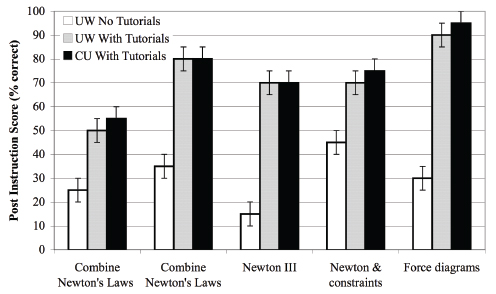
FIGURE 3.2.1 Post-test results on conceptual questions asked on midterm exams for students who used and did not use tutorials. Results from the University of Washington (UW) and University of Colorado (CU) are shown. SOURCE: Figure 4b from S.J. Pollock and N.D. Finkelstein, Sustaining educational reforms in introductory physics, Physics Review Special Topics—Physics Education Research 4:010110, 2008, Copyright 2008, The American Physical Society.
students can obtain high scores on the FCI several years after completion of a physics course that used interactive engagement methods.
Although active engagement and attending to students’ reasoning is common among the methods used in the research discussed here, no single method stands out from this body of work as the definitive way to teach undergraduate physics.
Differing goals, resources, constraints, and student populations all may require subtle differences in approach. Nonetheless, taken together, the many studies that assess the effectiveness of different research-based instructional approaches demonstrate that there are general principles that work, and providing significantly more effective instruction is a realistic goal for all physics departments.
Conceptual understanding has been an important but not an exclusive focus in PER. Important findings address other aspects of education as well. As discussed in Chapter 2, studies have revealed that students form attitudes and expectations regarding knowledge and learning in physics courses that are at odds with those of physicists (Redish et al., 1998; Adams et al., 2006; Halloun, 1996). These studies have also found that introductory physics instruction generally exacerbates the problem: students who complete such a course almost invariably express attitudes that are less, rather than more, aligned with those of physicists, than when they began the course (Box 3.3). Improving this situation is a matter of current investigation, but seems to involve direct and explicit attention to students’ views about learning science (Lindsey et al., 2012; Brewe et al., 2009; Redish and Hammer, 2009; Otero and Gray, 2008; Elby, 2001).
In addition to having positive impacts on student achievement, methods of interactive engagement have resulted in substantial improvements in the retention of underrepresented populations in physics. In particular, changes that have replaced the lecture with active learning have measured improved retention beyond the introductory course for both women and minority students (Brewe et al., 2010; Brahmia, 2008; Beichner, 2008). Other studies have come to conflicting conclusions about whether interactive methods alone can be responsible for a significant reduction of the gender gap (Lorenzo et al., 2006; Kost et al., 2009).
AREAS OF CURRENT AND EMERGING EMPHASIS IN RESEARCH
Many of the research themes that have been discussed in this report so far continue to be vital, with greater depth of understanding being achieved even in areas that have been under study for many years. At the same time, the field is diversifying, with researchers tackling increasingly interdisciplinary issues. The seven major areas of work in PER that are discussed below include applied research, with near-term practical implications for instruction, and basic research, aimed at developing a foundation of knowledge about the mechanisms of learning and reasoning. As in other areas of this report, this is not an attempt to include every topic that is or has been the subject of investigation. The choices reflect the report’s overall emphasis on improving undergraduate physics education; thus, some areas that are more highly speculative are not discussed. (The committee also favors studies conducted in U.S. institutions because of their more immediate relevancy.) However, as in other areas of research, it is often difficult to tell at early stages whether
BOX 3.3
Improved Conceptual Understanding at What Cost?
As the discussion in this chapter makes clear, much of PER-based instruction emphasizes the development of conceptual understanding. In some (but not all) courses that use PER-based methods, the introduction of activities aimed at developing concepts came at the expense of class time devoted to quantitative problem solving. In some laboratories, time spent on careful experimental technique and calculating uncertainties gave way to time spent addressing misconceptions. The question naturally arises: Do the observed improvements in performance on conceptual assessments come at the expense of expertise in quantitative problem solving or other important course goals? The few studies that have addressed this issue directly suggest that the answer is no (Ambrose et al., 1999; Crouch and Mazur, 2001). That is, there is typically a net gain in ability. There is not, however, an automatic improvement in student ability to solve end-of-chapter problems, either. These findings suggest that the methods traditionally used to teach problem solving were not optimally effective (see Hsu et al., 2004 for more discussion) such that reduction in time spent on them does not necessarily have a detrimental effect on students. These findings also suggest that the reasoning involved in applying concepts in qualitative problem solving and in quantitative problem solving are not as tightly linked for students as they are for physicists. However, problem solving when taught using PER methods, such as cooperative group problem solving, has been shown to be effective for both conceptual learning and problem solving (Redish, 1998; Cummings et al., 1999; Heller et al., 1992). As discussed in Chapter 3, improving student ability to solve problems is an area of ongoing investigation.
For some physics faculty, another concern raised is that the introduction of PER-based instructional methods will be detrimental to the students who make up the current population of physics majors (and by extension, the future leaders in the field). It has been suggested that these students will be bored and/or alienated by the emphasis on basic concepts and collaboration during class. There is no evidence that capable students who are initially intending to pursue physics tend to change their minds as a result of exposure to PER-based methods, although this issue has not been studied systematically. A relevant study in the context of an upper-division physics course did not find students resistant to PER-based methods but found significant support for them (Perkins and Turpen, 2009). There is also evidence that the top students benefit from these methods as much as, or more than, any other group of students (Ding, 2011; Heller et al., 1992; Meltzer, 2002; Singh, 2005; Steinberg, 1996; Vokos et al., 2000).
certain lines of inquiry will prove to have practical implications. Therefore, this report emphasizes that fundamental research is important, even if applications are not immediately apparent.
Below are brief accounts of current priorities for the following areas:
• Student conceptual understanding, reasoning, and problem solving;
• How students learn how to learn—in other words, how they learn how to “think like physicists”;
• The impact of the physical and social environment on learning in physics courses;
• Participation and achievement of students from groups traditionally underrepresented in physics;
• The preparation of future teachers of physics;
• The assessment of progress; and
• Scaling and sustaining research-supported instructional strategies.
Student Learning: Understanding, Reasoning, and Problem Solving
Student understanding of fundamental physics concepts has been, and continues to be, a major focus for PER. A considerable body of research that serves as a resource for instruction has been established (Docktor and Mestre, 2010; Duit, 2009; McDermott and Redish, 1999). The ability of students to do the reasoning necessary to develop, interpret, and apply concepts, especially in solving quantitative problems, is also a long-standing focus of investigation. Areas of current and emerging emphasis include the following:
• The nature and origins of conceptual difficulties in learning physics. Investigators are currently examining student understanding of physics topics at all levels of undergraduate instruction. Even in introductory physics, many topics have not been investigated as thoroughly as have the typical first-semester topics of kinematics and dynamics. The fundamental nature of difficulties themselves continues to be a topic of debate. In particular, the field has not yet reached consensus on the degree to which common conceptual errors stem from the application of “misconceptions” that are robust and stable or from the “in the moment” application of cognitive elements that exist at a much finer grain-size (Brown and Hammer, 2008; Minstrell, 1992; diSessa, 1993).
• The promotion of reasoning abilities. Since the earliest days of PER, reasoning skills have been an important focus (Reif, 1995; Renner and Lawson, 1973; McKinnon and Renner, 1971). Research has demonstrated that solving traditional end-of-chapter problems does not necessarily promote the ability to discuss or reason with underlying physics principles, although evidence suggests that these abilities are teachable (Leonard et al., 1996). Efforts to develop more effective strategies and problem sets include designing instructional approaches that promote the development of broadly applicable reasoning skills (e.g., Boudreaux et al., 2008) and nontraditional problem sets that emphasize conceptual reasoning in realistic scenarios. Such problem sets might be context-rich (Heller and Hollabaugh, 1992; Ogilvie, 2009), based on experiments (Van Heuvelen, 1995; Van Heuvelen et al., 1999), or related to real-world issues (http://relate.mit.edu/RwProblems/). The impact of students’ initial basic reasoning abilities on their learning in physics courses is also emerging as an important area of investigation (Bao et al., 2009a; Coletta et al., 2007; Moore and Rubbo, 2012).
• Factors affecting students’ ability to solve problems. A large body of research on students’ solutions to traditional quantitative problems exists (see Hsu et al., 2004, for a comprehensive discussion of problem-solving research). These studies reveal a wide gulf between what most physicists consider to be appropriate approaches to solving problems and the approaches taken by many students. Moreover, as discussed in the previous section, solving many problems does not necessarily lead to enhanced conceptual understanding (Kim and Pak, 2002). The converse also appears to be true: an increased emphasis in instruction on conceptual understanding does not automatically lead to improved problem-solving ability (although it typically does not lead to a decrease). The University of Minnesota Cooperative Group Problem Solving instructional strategy, however, has demonstrated that a curriculum heavily based on having students solve problems can lead to improved problem-solving abilities as well as improved conceptual learning (Redish et al., 1998; Cummings et al., 1999; Heller et al., 1992). This work employs the use of context (context-rich problems) and social learning (cooperative groups) and is one of the most widespread pedagogies in physics, although it still spans only a small fraction of physics classes. Explicitly modeling an organized set of problem-solving steps and reinforcing this framework in the course itself has also shown to result in higher course performance (Huffman, 1997; Heller and Reif, 1984; Wright and Williams, 1986; van Weeren et al., 1982).
In upper-level courses, efforts to improve problem-solving capabilities include adding laboratory experiments, more strongly linking mathematics and physics in problems, and introducing computational examples and problems (McGrath et al., 2008). These have been shown not only to improve problem-solving abilities, but also to improve retention of physics majors in the program (Manogue et al., 2001).
One area of current emphasis is the role of context. Evidence indicates that the statement of a problem may strongly influence the reasoning students use to solve it. For example, students often display appropriate conceptual understanding when responding to one problem statement, yet a seemingly identical problem framed slightly differently can trigger erroneous reasoning patterns (Brookes et al., 2011; Dufresne et al., 2002; Steinberg and Sabella, 1997). Ability to apply knowledge flexibly across contexts (broadly known as transfer of learning) has been a goal in cognitive science and PER for decades but remains elusive (Mestre, 2003, 2005; Nguyen and Rebello, 2011).
A second area of current emphasis is the role that mathematics plays in problem solving and conceptual understanding. In physics courses, in contrast to mathematics courses, mathematical expressions and symbols have conceptual meaning and describe relationships among physical quantities. Studying how students interpret and use those mathematical constructs provides a window into understanding how students attempt to solve problems (Sherin, 1996, 2001)
and how they learn, or fail to learn, the underlying physics (Hammer et al., 2005; Tuminaro and Redish, 2007).
• A more precise understanding of the role of interactive engagement on learning. As reported earlier, the available evidence supports the conclusion that interactive engagement methods lead to greater student learning. However, there are cases in which instructional strategies that at least superficially would be considered interactive have not led to significant conceptual learning gains (Cummings et al., 1999; Loverude et al., 2003). As a result, researchers are still pursuing a more precise understanding of the nature of interventions (and the critical elements of learning contexts) that result in improved learning. Moreover, most studies have taken place in actual classrooms with at least some uncontrolled variables.
There have not been many studies of the impact on students beyond the context of the reformed instruction itself. One was a study (Pollock, 2009) showing evidence of lasting benefits of conceptual understanding for students who took a reformed introductory physics course in electricity and magnetism using Tutorials in Introductory Physics (McDermott et al., 2002): In their junior year, students who had used the tutorials as freshmen scored significantly higher on a conceptual exam than students who had not. Another study (Etkina et al., 2010) showed evidence that students’ work in ISLE design laboratories (Etkina and Van Heuvelen, 2007) helped them in subsequent, non-ISLE novel experimental tasks.
While much progress has been made in the area of conceptual understanding, far less is known about the broad and less well defined objective of helping students learn to “think like physicists.” That is, much more is known about how to help students develop an understanding of concepts than about how to help them address open-ended, novel, challenging questions in ways that build toward professional expertise. Many physics faculty would agree these are important goals, and AIP surveys indicate that they are for employers. Yet such goals are seldom primary targets of physics instruction until students conduct research in later undergraduate years or in graduate school.
Evidence suggests that even instructional approaches that produce conceptual gains may leave students reliant—and expecting to be reliant—on guidance from instructors (Redish et al., 1998). Students do not expect to be able to address situations they have not encountered before or to judge for themselves when an answer makes sense. Instead, students’ principal method for assessing their understanding is to check that their answers to exercises align with the published solutions. In these respects, what students take away from physics courses systematically contradicts practices within the discipline. The enterprise of physics is learning about
the physical world; physicists are professional learners. Physicists do their work without the benefit of an authority who can tell them when they have things right.
Abundant evidence from surveys, interviews, and observations of students in introductory courses shows that most students think of learning physics as a matter of remembering and rehearsing facts, formulas, and computational techniques (Adams et al., 2006; Halloun and Hestenes, 1998; Hammer, 1994; Kortemeyer, 2007; May and Etkina, 2002; Van Heuvelen, 1991). These findings are in contrast with research that extensively documents young children’s abilities and inclinations to have and express their own ideas, to assess their ideas for consistency and fit with evidence, and, in general, to seek a coherent, mechanistic understanding of natural phenomena (Gopnik and Schulz, 2004; Koslowski, 1996; Lehrer, 2009; Metz, 2011; NRC, 2007).
Why would a pursuit of understanding that begins with such promise in young children all but disappear in students by the time they get to college physics courses? A likely conjecture is that science instruction guides students to focus on achieving fidelity to a canon of ideas specified by teachers and textbooks, but as yet no strong evidence supports that conjecture, such as might be provided by longitudinal studies. Accounts of science instruction have highlighted how goals of students learning to reason for themselves and goals of their arriving at particular conclusions may be in tension (Hodson, 1988), but little research provides guidance on how to improve the situation.
Increasing attention within PER is being paid to how students may learn to adopt and develop facility in disciplinary practices of learning—of having and articulating their own ideas; assessing the quality of those ideas for explanatory and predictive power; designing and conducting their own experimental tests; and identifying and reconciling theoretical inconsistencies (Elby, 2001; Hammer et al., 2005; Etkina, 2010).
The Role of Physical and Social Environments in Learning Physics
The recent emergence of new technologies, including newly pervasive social and informational media and online courses, as well as more specifically pedagogical online simulations, tutoring, homework, and interaction systems, are changing the environment for learning and instruction. Lectures can be recorded on video and published; texts can be interactive; classes can meet and interact in virtual spaces, and massive online open courses are finding a presence in the lives of students and teachers. The pace of this change is rapid. It affects both the design of instruction and the expectations of students with respect to how they obtain knowledge and skills. Yet little is known about its implications for learning complex material. One thing is clear: schools are no longer essential as sources of information. Many educators argue that their role needs to shift toward helping students
learn how to assess and make use of the information they can access through the Internet (which is for many available on the mobile devices in their pockets).
Understanding how physical and social spaces for learning (both online and onsite) are best organized to meet the needs of today’s students has become an important research priority. Research has been done on how physical classroom arrangements (e.g., seating arrangements, the use of clickers or dry erase whiteboards, and so on) can influence learning (Price et al., 2011; Beichner et al., 2007). This research connects closely with research on social arrangements, both designed and emergent, in which students speak with each other, for example, rather than only to the instructor; have the privilege (or obligation) to influence what questions and ideas the class will discuss; or participate in assessing their own learning or that of their classmates. Previous research shows how physical and social aspects of the environment interact with each other as well as with progress toward learning goals (Otero, 2004; Duit et al., 1998). For instance, some environments are more conducive to students engaging in scientific behaviors (such as making sense of physical phenomena, making inferences on the basis of evidence, argumentation, asking empirical questions, and so on) than others (Goldberg et al., 2010; Driver et al., 2000). Other studies have found that some environments are more inclusive than others (Ross and Otero, 2012; Brahmia and Etkina, 2001; Lee and Fradd, 1998) and suggest that some environments actually alienate a significant fraction of the students enrolled in the class (e.g., Lemke, 2001). Cooperative group-learning instructional environments have been shown to be capable of improving student learning for a broad range of students and are an important component of many PER-based instructional strategies.
A meta-analysis of studies that compared face-to-face and online instruction concluded that online environments are at least as effective as face-to-face classroom environments, but environments that combined face-to-face with online instruction showed statistically significant higher learning outcomes than those with only face-to-face instruction (see DOE, 2010, for meta-analysis). Less work has been done in combining what is known about effective active-engagement strategies with social aspects of online instructional environments.
Participation and Achievement of Students from Groups Traditionally Underrepresented in Physics
As an academic discipline, physics has a significant underrepresentation of women and ethnic/racial minorities as faculty, graduate students, undergraduate majors, and students in calculus-based courses (NCSES, 2011). Therefore, many of those entrusted with designing and teaching physics courses often have little or no experience in physics contexts involving a heterogeneous and diverse group of students. It is perhaps not surprising, then, that the largest participation and
achievement gaps observed in science occur in introductory physics (http://www.aip.org/statistics/). Yet, as noted in Chapter 2, a growing number of students from diverse racial and ethnic backgrounds are enrolling in introductory physics, in part because populations in other disciplines that require a physics course are becoming increasingly diverse. Thus, the number of students from groups traditionally underrepresented in physics courses is greater than ever before and increasing.
A variety of studies have explored why achievement gaps continue to exist in science and why various groups of students continue to be underrepresented in physics (Kost et al., 2009; Hazari et al., 2007). Evidence shows that the achievement gap is not explained by student-specific characteristics, such as attitudes, motivation, or family support, nor is the achievement gap fully explained by poor academic preparation (Kost et al., 2009; McCullough, 2002).
Increasing evidence indicates that “self-efficacy,” or the belief in one’s own ability to succeed in a subject, is an important component to success in other science fields (Bandura, 1986; Zeldin and Pajares, 2000; Miyake et al., 2010). Specific activities, such as participating in learning communities and participating in undergraduate research, have been shown to greatly impact the retention of underrepresented students in science and engineering (Watkins and Mazur, 2013). Physics-specific research publications in these areas are few but are starting to appear. As mentioned earlier, evidence suggests that active learning environments increase performance for students from groups traditionally underrepresented in science (Brewe et al., 2010). Increasing attention in PER is being paid to studying how external characteristics, such as course format and participation in university-based activities, impact the performance and retention of students traditionally underrepresented in physics.
Preparation of Future Physics Teachers
Colleges and universities have long been the locus of physics teacher preparation. However, at a majority of U.S. universities, neither the college of education nor the physics department typically takes full responsibility for the preparation of physics teachers (National Task Force Report on Teacher Education in Physics, 2013; Buck et al., 2000). This lack of clarity concerning where physics teacher preparation should take place has led to a set of challenges both in the preparation of physics majors to teach physics and in the research associated with this preparation. Research on physics teacher preparation has proven to be challenging, due to the relatively small number of programs that prepare physics teachers specifically (National Task Force on Teacher Education in Physics, 2013); a lack of consensus on how to determine high-quality teaching and teacher preparation (NRC, 2010); and the great diversity of students, the variety of contexts, and the rapidly changing environments in which teachers work (NRC, 2010). Nonetheless, evidence
from physics education research points to features of programs that are effective for preparing physics teachers (Meltzer, 2011). Current research emphasizes how classroom teaching is affected by the instruction and experiences of teacher candidates in their physics and teacher preparation programs (NRC, 2010). While more research is needed, key points for physics and other science teachers are that they need college-level study in the field that they will be teaching that is suitable to their students’ age groups, an understanding of the objectives for students’ learning science and developing science proficiency, and a command of the various instructional approaches designed to meet those objectives.
Related research concerns the preparation of teaching assistants (TAs) for their roles in instruction, both in their graduate programs and in their future roles as faculty. The development of TA attitudes toward teaching and their parallel development of expertise have been the focus of programs at several institutions, such as the University of Colorado and the University of Minnesota, and is the subject of current research by several groups.
As faculty and departments move more toward improving their courses, nationally normed assessment instruments for specific learning objectives provide convincing motivation for change as well as evidence that change is successful. Researchers also rely significantly on assessment instruments when developing new instructional methods. It is clear that assessment instruments like the FCI and FMCE—and more recently the MPEX and the Colorado Learning Attitudes about Science Survey—have brought conceptual knowledge and student attitudes into common discussion among physicists, revealing deficiencies in conceptual outcomes of traditional courses and motivating the adoption of many of the pedagogical innovations discussed throughout this report. Along with basic data on demographics (e.g., recruitment of majors, retention of underserved groups in an introductory course, and so on), these assessments provide the majority of the quantitative evidence cited in this report.
The tremendous impact of the few nationally normed assessment instruments currently available underscores the imperative for education researchers to develop and validate assessments that are easy for nonspecialists to administer and interpret. It is particularly important to develop ways to assess problem-solving ability, critical thinking in physics, scientific communication skills, student engagement, and so on, as well as developing assessments for other desirable objectives, such as predicting future learning or measuring teacher or TA preparation. In some of these areas there exists very little conclusive research on which to draw, implying the need for new basic research, some of which may involve novel approaches to assessment (e.g., Baker et al., 2011).
Scaling and Sustaining Research-Supported Instructional Strategies
To aid in the process of putting research results into practice, the PER community has engaged in substantial dissemination and implementation efforts. Curriculum developers frequently prepare publications—both for peer-reviewed journals and for classroom use—to make presentations and present at workshops. With respect to introductory algebra and calculus-based physics, currently almost all physics faculty (87 percent) say that they are familiar with one or more PER-based instructional strategy, and approximately half (48 percent) say that they currently use at least one PER-based strategy (Henderson and Dancy, 2009). The Workshop for New Physics and Astronomy Faculty, mentioned in Chapter 2, is a special effort to disseminate the results of PER to new physics faculty. Workshop participants report large increases in knowledge about and use of PER-based materials (Henderson, 2008; Henderson et al., 2012).
PER-based efforts have made significant headway into undergraduate physics education, but at the same time, they are not as broadly implemented as they could be. Research has shown that PER-based strategies are frequently not implemented as described by developers, and many faculty who try a PER-based strategy eventually discontinue use for a variety of reasons (Henderson and Dancy, 2009). Research is just beginning to shed light on the complex dynamics that are required for implementing, scaling and sustaining instructional changes (Yerushalmi et al., 2007; Henderson, 2007; and Henderson et al., 2012).
Establishing and maintaining effective practices and curricula in physics departments is currently a poorly understood challenge. Historically, change agents, usually curriculum developers, would work with individual faculty to support them in adopting various curricula with the expectation that the curricula would be adopted with minimal changes. However, factors that impede adoption of research-based materials seem to depend on a range of issues, including the type of institution and the orientation of the department. The size of classes, the support of colleagues, and the pressures to conduct research and obtain external funding for that research are all variables that affect the commitment to making changes in instruction. At some research-oriented institutions, tenure-track faculty might be discouraged from spending “too much time” in teaching innovations while establishing their research credentials. At others, innovative teaching could be part of the expectation for tenure. These issues increase the complexity of the models for development and dissemination of research-based instructional materials and practices. Thus, research aimed at the development of models of how to go from evidence-based knowledge in PER into practice is beginning to be recognized as important for the future.
While a few physicists may be naturally talented teachers who can reach a broad spectrum of students using instinct alone, most physics faculty can improve their teaching just as they improve other scholarly efforts, by incorporating practices based on scientific evidence. Over the past few decades, physics education research has provided a new perspective on issues related to the teaching and learning of physics. This research, which uses as a foundation the methods of physics and is conducted primarily by physicists, has collected data and built models to help us begin to understand what is happening in our classrooms, including why talented students turn away from physics. By incorporating research-based practices into teaching efforts, the physics community can reach a broader, more diverse audience and make the learning of physics a more productive and enjoyable experience for all students.
While research indicates that no easy or best teaching methods exists, it continually returns to one fundamental conclusion: Faculty need to actively engage students in the learning process, paying attention to their spontaneous ways of thinking and the models of the natural world that they obtain from everyday life. With this fundamental principle in mind, PER has developed a number of strategies that can help fix some of the problems that are faced. However, PER as a discipline is quite young. As it develops, and as students and society change, one can expect the need for research on issues that are only beginning to emerge as important or that are not yet anticipated.
The lines of research identified in this chapter have both short- and long-term implications for undergraduate physics education. The increasing availability of technological tools—ranging from systems for collecting high-quality classroom video to tracking eye movements and fMRI—are opening up previously unexplored areas for investigation. Talented faculty, graduate students and postdoctoral students are being attracted to the field of PER and are motivated by the discovery potential common to all fundamental research and by the prospect of conducting research that can have a powerful impact on people’s lives. The successes outlined in this report demonstrate that PER has established a viable model for transforming insights about how people learn physics into significant improvements in classroom instruction. On this foundation the field is poised to help address the urgent problems facing physics education identified in this document. However, a number of practical challenges, some of them common to all research fields and others specific to discipline-based education research, threaten to hinder progress. Chapter 4 offers recommendations for promoting the vitality of the field of PER and thereby supporting advances in physics education.
Adams, W., Perkins, K., Podolefsky, N., Dubson, M., Finkelstein, N., and Wieman, C. 2006. A new instrument for measuring student beliefs about physics and learning physics: The Colorado Learning Attitudes about Science Survey. Physical Review Special Topics—Physics Education Research 2:10101-1.
Ambrose, B.S. 2004. Investigating student understanding in intermediate mechanics: Identifying the need for a tutorial approach to instruction. American Journal of Physics 72:453.
Ambrose, B.S., Shaffer, P.S., Steinberg, R.N., and McDermott, L.C. 1999. An investigation of student understanding of single-slit diffraction and double-slit interference. American Journal of Physics 67:146.
Baker, R.S.J.D., Gowda, S., and Corbett, A. 2011. Automatically detecting a student’s preparation for future learning: Help use is key. Pp. 179-188 in Educational Data Mining Conference Proceedings 2011. Available at http://educationaldatamining.org/EDM2011/wp-content/uploads/proc/edm11_proceedings.pdf, accessed on April 23, 2013.
Bandura, A. 1986. Social Foundations of Thought and Action: A Social Cognitive Theory. Prentice Hall, Englewood Cliffs, N.J.
Bao, L., Cai, T., Koenig, K., Fang, K., Han, J., Wang, J., Liu, Q., Lin, D., Cui, L., Luo, Y., Wang, Y., and Wu, N. 2009a. Learning and scientific reasoning. Science 323:586-587.
Bao, L., Fang, K., Cai, T., Wang, J., Yang, L., Cui, L., Han, J., Ding, L., and Luo, Y. 2009b. Learning of content knowledge and development of scientific reasoning ability: A cross culture comparison. American Journal of Physics 77:1118.
Beichner, R.J. 1994. Testing student interpretation of kinematics graphs. American Journal of Physics 62:750-762.
———. 2008. The SCALE-UP Project: A Student-Centered Active Learning Environment for Undergraduate Programs. A commissioned paper for the National Research Council’s Evidence on Promising Practices in Undergraduate Science, Technology, Engineering, and Mathematics (STEM) Education Workshop 2, October 13-14, 2008. Available at http://sites.nationalacademies.org/DBASSE/BOSE/DBASSE_071087; accessed on April 23, 2013.
Beichner, R.J., Saul, J.M., Abbott, D.S., Morse, J.J., Deardorff, D.L., Allain, R.J., et al. 2007. The Student-Centered Activities for Large Enrollment Undergraduate Programs (SCALE-UP) project. In Reviews in Physics Education Research, Volume 1: Research-based Reform of University Physics (E.F. Redish and P.J. Cooney, eds.). American Association of Physics Teachers, Washington, D.C. Available at http://www.per-central.org/per_reviews/.
Boudreaux, A., Shaffer, P.S., Heron, P.R.L., and McDermott, L.C. 2008. Student understanding of control of variables: Deciding whether or not a variable influences the behavior of a system. American Journal of Physics 76:163.
Brahmia, S. 2008. Improving learning for underrepresented groups in physics for engineering majors. PER Conference Invited Paper. Pp. 7-10 in 2008 Physics Education Research Conference. AIP Conference Proceedings 1064. Available at http://www.compadre.org/Repository/document/ServeFile.cfm?ID=7981&DocID=698; accessed on February 19, 2013.
Brahmia, S., and Etkina, E. 2001. Switching students on to science: An innovative course design for physics students. Journal of College Science Teaching 31:183-187.
Brewe, E., Kramer, L., and O’Brien, G. 2009. Modeling instruction: Positive attitudinal shifts in introductory physics measured with CLASS. Physical Review Special Topics—Physics Education Research 5:013102.
Brewe, E., Sawtelle, V., Kramer, L., O’Brien, G., Rodriguez, I., and Pamela, P. 2010. Toward equity through participation in modeling instruction in introductory university physics. Physical Review Special Topics—Physics Education Research 6:010106.
Brookes, D.T., Ross, B.H., and Mestre, J.P. 2011. Specificity, transfer, and the development of expertise. Physical Review Special Topics—Physics Education Research 7:010105.
Brown, D.E., and Hammer, D. 2008. Conceptual change in physics. Pp. 127-154 in International Handbook of Research on Conceptual Change (S. Vosniadou, ed.). Routledge, New York, N.Y.
Buck, G.A., Hehn, J.G., and Leslie-Pelecky, D.L., eds. 2000. The Role of Physics Departments in Preparing K-12 Teachers. American Institute of Physics, College Park, Md.
Cataloglu, E., and Robinett, R.W. 2002. Testing the development of student conceptual and visualization understanding in quantum mechanics through the undergraduate career. American Journal of Physics 70:238-251.
Chasteen, S.V., Pepper, R.E., Pollock, S.J., and Perkins, K.K. 2011. But does it last? Sustaining a research-based curriculum in upper-division electricity and magnetism. 2011 Physics Education Research Conference. AIP Conference Proceedings 1413. Available at http://proceedings.aip.org/resource/2/apcpcs/1413/1/139_1?isAuthorized=no; accessed on April 23, 2013.
Clement, J.J. 1982. Students’ preconceptions in introductory mechanics. American Journal of Physics 50:66-71.
Cochran, M.J., and Heron, P.R.L. 2006. Development and assessment of research-based tutorials on heat engines and the second law of thermodynamics. American Journal of Physics 74:734.
Coletta, V.P., Phillips, J.A., and Steinert, J.J. 2007. Why you should measure your students’ reasoning ability. The Physics Teacher 45:235.
Crouch, C.H., and Mazur, E. 2001. Peer instruction: Ten years of experience and results. American Journal of Physics 69:970-977.
Cummings, K. 2010. A Developmental History of Physics Education Research. Commissioned paper for the National Research Council on Status, Contributions, and Future Direction of Discipline-Based Education Research; available at http://www7.nationalacademies.org/bose/DBER_Cummings_October_Paper.pdf, accessed March 27, 2012.
Cummings, K., Marx, J., Thornton, R.K., and Kuhl, D. 1999. Evaluating innovation in studio physics. American Journal of Physics—Physics Education Research Supplement 67:S38-S44.
Ding, L., Chabay, R., Sherwood, B., and Beichner, R. 2006. Evaluating an electricity and magnetism assessment tool: Brief electricity and magnetism assessment. Physical Review Special Topics—Physics Education Research 2:010105.
Ding, L., Reay, N., Lee, A., and Bao, L. 2011. Exploring the role of conceptual scaffolding in solving synthesis problems. Physical Review Special Topics—Physics Education Research 7:020109.
diSessa, A.A. 1993. Toward an epistemology of physics. Cognition and Instruction 10:105-225.
Docktor, J.L., and Mestre, J.P. 2010. A Synthesis of Discipline-Based Education Research in Physics. Commissioned paper for the National Research Council on Status, Contributions, and Future Direction of Discipline-Based Education Research; available at http://www7.nationalacademies.org/bose/DBER_Docktor_October_Paper.pdf, accessed October 8, 2011.
DOE (U.S. Department of Education). 2010. Evaluation of Evidence-Based Practices in Online Learning: A Meta-Analysis and Review of Online Learning Studies. Office of Planning, Evaluation, and Policy Development, Washington, D.C., available at http://www.ed.gov/about/offices/list/opepd/ppss/reports.html
Driver, R., Newton, P., and Osborne, J. 2000. Establishing the norms of scientific argumentation in classrooms. Science Education 84:287-312.
Dufresne, R.J., Leonard, W.J., and Gerace, W.J. 2002. Making sense of students’ answers to multiple choice questions. The Physics Teacher 40:174-180.
Duit, R. 2009. Bibliography—Students’ and Teachers’ Conceptions and Science Education. Available at http://www.ipn.uni-kiel.de/aktuell/stcse/stcse.html, accessed October 8, 2011.
Duit, R., Roth, W., Komorek, M., and Withers, J. 1998. Conceptual change cum discourse analysis to understand cognition in a unit on chaotic systems: Toward an integrative perspective on learning in science. International Journal of Science Education 20:1059-1073.
Duprez, C., and Méheut, M. 2003. About some of the difficulties in learning thermodynamics at the university level. Pp. 99-106 in Science Education Research in the Knowledge-Based Society (D. Psillos, P. Kariotoglou, V. Tselfes, E. Hatzikraniotis, G. Fassoulopoulos, and M. Kallery, eds.). Kluwer Academic Publishers, Dordrecht, The Netherlands.
Elby, A. 2001. Helping students learn how to learn. American Journal of Physics, Physics Education Research Supplement 69:S54-S64.
Etkina, E. 2010. Pedagogical content knowledge and preparation of high school physics teachers. Physical Review Special Topics—Physics Education Research 6:020110.
Etkina, E., and Van Heuvelen, A. 2007. Investigative science learning environment—A science process approach to learning physics. Research-Based Reform of University Physics. American Association of Physics Teachers, Washington, D.C.
Etkina, E., Karelina, A., Ruibal-Villasenor, M., Jordan, R., Rosengrant, D., and Hmelo-Silver, C. 2010. Design and reflection help students develop scientific abilities: Learning in introductory physics laboratories. Journal of the Learning Sciences 19(1):54-98.
Francis, G.E., Adams, J.P., and Noonan, E.J. 1998. Do they stay fixed? The Physics Teacher 36:488.
Goldberg, F.M., and McDermott, L.C. 1987. An investigation of student understanding of the real image formed by a converging lens or concave mirror. American Journal of Physics 55:108.
Goldberg, F., Robinson, S., and Otero, V. 2008. Physics and Everyday Thinking. It’s About Time, Armonk, N.Y.
Goldberg, F., Otero, V., and Robinson, S. 2010. Design principles for effective physics instruction: A case from physics and everyday thinking. American Journal of Physics 78:1265.
Gopnik, A., and Schulz, L. 2004. Mechanisms of theory-formation in young children. Trends in Cognitive Science 8:8.
Hake, R.R. 1998. Interactive-engagement versus traditional methods: A six-thousand-student survey of mechanics test data for introductory physics courses. American Journal of Physics 66:64-74.
Halloun, I. 1996. Views about science and physics achievement: The VASS story. Pp. 605-613 in Proceedings of the International Conference on Undergraduate Physics Education (E.F. Redish and J.S. Rigden, eds.). American Institute of Physics, Washington D.C.
Halloun, I., and Hestenes, D. 1998. Interpreting VASS dimensions and profiles. Science and Education 7:553-577.
Hammer, D. 1994. Epistemological beliefs in introductory physics. Cognition and Instruction 12:151-183.
Hammer, D., Elby, A., Scherr, R.E., and Redish, E.F. 2005. Resources, framing, and transfer. Pp. 89-120 in Transfer of Learning: Research and Perspectives (J. Mestre, ed.). Information Age Publishing, Greenwich, Conn.
Hartmann, S., and Niedderer, H. 2005. Parallel conceptions in the domain of force and motion. Pp. 471-482 in Research and the Quality of Science Education (K. Boersma, M. Goedhart, O. De Jong, and H. Eijkelhof, eds.). Springer, Dordrecht, The Netherlands.
Hazari, Z., Tai, R.H., Sadler, P.M. 2007. Differences in introductory university physics performance: The influence of H.S. physics preparation and affective factors. Science Education 91(6):847-876.
Heller, P., and Hollabaugh, M. 1992. Teaching problem solving through cooperative grouping—2: Designing problems and structuring groups. American Journal of Physics 60:637-644.
Heller, P., Keith, R., and Anderson, S. 1992. Teaching problem solving through cooperative grouping—1: Group versus individual problem solving. American Journal of Physics 60:627-636.
Heller, T.L., and Reif, F. 1984. Prescribing effective human problem-solving processes: Problem solving in physics cognition and instruction. Cognition and Instruction 1(2):177.
Henderson, C. 2007. Evaluation of the Physics and Astronomy New Faculty Workshop. Contributed talk for the AAPT 2007 Summer Meeting, Greensboro, N.C., July 30, 2007. Available at http://homepages.wmich.edu/~chenders/Publications/HendersonAAPTSummer2007.pdf; accessed on May 7, 2013.
———. 2008. Promoting instructional change in new faculty: An evaluation of the physics and astronomy new faculty workshop. American Journal of Physics 76:179-187.
Henderson, C., and Dancy, M. 2009. The impact of physics education research on the teaching of introductory quantitative physics in the United States. Physical Review Special Topics—Physics Education Research 5:020107.
Henderson, C., Dancy, M., and Niewiadomska-Bugaj, M. 2012. Use of research-based instructional strategies in introductory physics: Where do faculty leave the innovation-decision process? Physical Review Special Topics—Physics Education Research 8:020104.
Hestenes, D., Wells, M., and Swackhamer, G. 1992. Force concept inventory. The Physics Teacher 30:141-166.
Hodson, D. 1988. Experiments in science and science teaching. Educational Philosophy and Theory 20:53-66.
Hsu, L., Brewe, E., Foster, T., and Harper, K., 2004. Resource Letter RPS-1: Research in problem solving. American Journal of Physics 72:1148-1156.
Huffman, D. 1997. Effect of explicit problem-solving instruction on high school students’ problem-solving performance and conceptual understanding of physics. Journal Research in Science Teaching 36(6):337-379.
Kim, E., and Pak, S. 2002. Students do not overcome conceptual difficulties after solving 1000 traditional problems. American Journal of Physics 70:759.
Knight, R. 2002. Five Easy Lessons: Strategies for Successful Physics Teaching. Addison-Wesley, San Francisco, Calif.
Kortemeyer, G. 2007. The challenge of teaching introductory physics to premedical students. The Physics Teacher 45:552-557.
Koslowski, B. 1996. Theory and Evidence: The Development of Scientific Reasoning. MIT Press, Cambridge, Mass.
Kost, L.E., Pollock, S.J., and Finkelstein, N.D. 2009. Characterizing the gender gap in introductory physics. Physical Review Special Topics—Physics Education Research 5:010101.
Laws, P. 1991. Calculus-based physics without lectures. Physics Today 44:24-31.
———. 1997. Workshop Physics Activity Guide. John Wiley and Sons, New York, N.Y.
Lee, O., and Fradd, S. 1998. Science for all, including students from non-English-language backgrounds. Educational Researcher 27:12-21.
Lehrer, R. 2009. Designing to develop disciplinary dispositions: Modeling natural systems. American Psychologist 64:759-771.
Lemke, J. 2001. Articulating communities: Sociocultural perspectives on science education. Journal of Research in Science Teaching 38:296-316.
Leonard, W.J., Dufresne, R.J., and Mestre, J.P. 1996. Using qualitative problem-solving strategies to highlight the role of conceptual knowledge in solving problems. American Journal of Physics 64:1495-1503.
Lindsey, B., Hsu, L., Sadaghiani, H., Taylor, J.W., and Cummings, K. 2012. Positive attitudinal shifts with the Physics by Inquiry curriculum across multiple implementations. Physical Review Special Topics—Physics Education Research 8:010102.
Lorenzo, M., Crouch, C., and Mazur, E., 2006. Reducing the gender gap in the physics classroom. American Journal of Physics 74:118.
Loverude, M.E. 2003. “Measuring the Effectiveness of Research-Based Curriculum at a University Serving a Diverse Student Population.” Presented at the 2003 Physics Education Research Conference, Madison, Wis.
Maloney, D., O’Kuma, T., Hieggelke, C., and Van Heuvelen, A. 2001. Surveying students’ conceptual knowledge of electricity and magnetism. American Journal of Physics 69:S12.
Manogue, C.A., Siemens, P.J., Tate, J., Browne, K., Niess, M.L., and Wolfer, A.J. 2001. Paradigms in physics: A new upper-division curriculum. American Journal of Physics 69(9):978-990.
May, D.B., and Etkina, E. 2002. College physics students’ epistemological self-reflection and its relationship to conceptual learning. American Journal of Physics 70:1249-1258.
Mazur, E. 1997. Peer Instruction: A User’s Manual. Prentice-Hall, Upper Saddle River, N.J.
McCullough, L. 2002. Gender, math, and the FCI. In Proceedings of the 2002 Physics Education Research Conference (S. Franklin, J. Marx, and K. Cummings, eds.). AIP Press, Melville, N.Y.
McDermott, L.C. 1991. Millikan Lecture 1990: What we teach and what is learned—Closing the gap. American Journal of Physics 59:301-315.
McDermott, L.C., and Redish, E.F. 1999. Resource letter: PER-1: Physics Education Research. American Journal of Physics 67:755-767, and references cited therein.
McDermott, L.C., Shaffer, P.S., Rosenquist, M.L., and Washington, P.E.G.U. 1996. Physics by Inquiry: An Introduction to Physics and the Physical Sciences (Volumes I and II). John Wiley and Sons, New York, N.Y.
McDermott, L.C., Shaffer, P.S., and Physics Education Group at the University of Washington. 1998. Tutorials in Introductory Physics. Prentice Hall, Upper Saddle River, N.J.
———. 2002. Tutorials in Introductory Physics. Pearson Publishing, Upper Saddle River, N.J.
McDermott, L.C., Heron, P., Shaffer, P., and Stetzer, M. 2006. Improving the preparation of K-12 teachers through physics education research. American Journal of Physics 74:763-767.
McGrath, D., Savage, C., Williamson, M., Wegener, M., and McIntyre T. 2008. Teaching Special Relativity using Virtual Reality. Proceedings of the UniServe Science Symposium on Visualisation and Concept Development, October 2008. Available at http://www.anu.edu.au/Physics/Savage/papers/uniserve.pdf; accessed on May 6, 2013.
McKagan, S.B., Perkins, K.K., and Weiman, C.E. 2010. Design and validation of the quantum mechanics conceptual survey. Physical Review Special Topics—Physics Education Research 6:020121.
McKinnon, J.W., and Renner, J.W. 1971. Are colleges concerned with intellectual development? American Journal of Physics 39:1047-1052.
Meltzer, D. 2002. The relationship between mathematics preparation and conceptual learning gains in physics: A possible “hidden variable” in diagnostic pretest scores. American Journal of Physics 70:1259-1268.
———. 2004. Investigation of students’ reasoning regarding heat, work, and the first law of thermodynamics in an introductory, calculus-based general physics course. American Journal of Physics 72:1432-1446.
———. 011. Research on the education of physics teachers. Pp. 3-14 in Teacher Education in Physics: Research, Curriculum, and Practice (D. Meltzer and P. Shaffer, eds.). American Physical Society, College Park, Md.
Meltzer, D., and Manivannan, K. 1996. Promoting interactivity in physics lecture classes. The Physics Teacher 34:72-76.
Meltzer, D., and Thornton, R. 2012. Resource letter ALIP-1: Active-Learning Instruction in Physics. American Journal of Physics 80:478.
Mestre, J. 2003. Transfer of Learning: Issues and Research Agenda. National Science Foundation, Washington, D.C. Available at www.nsf.gov/pubs/2003/nsf03212/nsf03212.pdf.
Mestre, J.P., ed., 2005. Transfer of Learning from a Modern Multidisciplinary Perspective. Information Age Publishing, Greenwich, Conn.
Metz, K.E. 2011. Young children can be sophisticated scientists. Phi Delta Kappan 92:68-71.
Minstrell, J. 1992. Facets of students’ knowledge and relevant instruction. Pp. 110-128 in The Proceedings of the International Workshop on Research in Physics Education: Theoretical Issues and Empirical Studies (Bremen, Germany, March 5-8, 1991) (R. Duit, F. Goldberg, and H. Niedderer, eds.). IPN (Institut für die Pädagogik der Naturwissenschaften), Kiel, Germany.
Miyake, A., Kost-Smith, L.E., Finkelstein, N.D., Pollock, S.J., Cohen, G.L., and Ito, T.A. 2010. Reducing the gender achievement gap in college science: A classroom study of values affirmation. Science 330:1234-1237.
Moore, J.C., and Rubbo, L.J. 2012. Scientific reasoning abilities of nonscience majors in physics-based courses. Physical Review Special Topics—Physics Education Research 8:010106.
National Task Force on Teacher Education in Physics. 2013. Transforming the Preparation of Physics Teachers: A Call to Action. American Physical Society. Available at http://www.ptec.org/webdocs/2013TTEP.pdf; accessed on April 23, 2013.
NCSES (National Center for Science and Engineering Statistics). 2011. Characteristics of Scientists and Engineers in the United States: 2006. National Science Foundation, Arlington, Va.
Nguyen, D.-H., and Rebello, N.S. 2011. Students’ understanding and application of the area under the curve concept in physics problems. Physical Review Special Topics—Physics Education Research 7:010112.
NRC (National Research Council). 2000. How People Learn: Brain, Mind, Experience, and School. The National Academies Press, Washington, D.C.
———. 2007. Taking Science to School: Learning and Teaching Science in Grades K-8. The National Academies Press, Washington, D.C.
———. 2010. Preparing Teachers: Building Evidence for Sound Policy. The National Academies Press, Washington, D.C.
———. 2012. Discipline-Based Education Research: Understanding and Improving Learning in Undergraduate Science and Engineering. The National Academies Press, Washington, D.C.
Ogilvie, C.A. 2009. Changes in students’ problem-solving strategies in a course that includes context-rich, multifaceted problems. Physical Review Special Topics—Physics Education Research 5: 020102.
Otero, V. 2004. Cognitive processes and the learning of physics, Part II: Mediated action. Proceedings of the 2003 International School of Physics “Enrico Fermi” (M. Vicentini and E.F. Redish, eds). IOS Press, Amsterdam, The Netherlands.
Otero, V., and Gray, K. 2008. Attitudinal gains across multiple universities using the Physics and Everyday Thinking curriculum. Physical Review Special Topics—Physics Education Research 4:020104.
Perkins, K.K., and C. Turpen. 2009. Student perspectives on using clickers in upper-division physics courses. Pp. 225-228 in 2009 Physics Education Research Conference. AIP Conference Proceedings 1179. AIP Press, Melville, N.Y.
Pollock, S.J. 2009. Longitudinal study of student conceptual understanding in electricity and magnetism. Physical Review Special Topics—Physics Education Research 5:020110.
———. 2012. Impacts of curricular change: 8 years of conceptual survey data from introductory physics. In 2012 Physics Education Research Conference (S. Rebello, P. Engelhardt, and A. Churukian, eds.). AIP Press, Melville, N.Y.
Pollock, S.J., and Finkelstein, N.D. 2008. Sustaining educational reforms in introductory physics. Physical Review Special Topics—Physics Education Research 4:010110.
Prather, E.E., Rudolph, A.L., Brissenden, G., and Schlingman, W.M. 2009. A national study assessing the teaching and learning of introductory astronomy. Part I. The effect of interactive instruction. American Journal of Physics 77:320-330.
Price, E., Tsui, S., Hart, A., and Saucedo, L. 2011. Don’t erase that whiteboard! Archiving student work on a photo sharing website. The Physics Teacher 49:334-336.
Redish, E., and Hammer, D. 2009. Reinventing college physics for biologists: Explicating an epistemological curriculum. American Journal of Physics 77:629-642.
Redish, E.F., and Steinberg, R.N. 1999. Teaching physics: Figuring out what works. Physics Today 52:24-30.
Redish, E.F., Saul, J.M., and Steinberg, R.N. 1998. Student expectations in introductory physics. American Journal of Physics 66: 212-224.
Reif, F. 1995. Millikan Lecture 1994: Understanding and teaching important scientific thought processes. American Journal of Physics 63:17-32.
Renner, J.W., and Lawson, A.E. 1973. Promoting intellectual development through science teaching. The Physics Teacher 11:273.
Ross, M.J., and Otero, V.K. 2012. Challenging traditional assumptions about secondary science through the PET curriculum. 2012 Physics Education Research Conference Proceedings (S. Rebello, P. Engelhardt, and A. Churukian, eds.). AIP Press, Melville, N.Y.
Schecker, H., and Gerdes, J. 1999. Messung von Kozeptualisierungsfaehigkeit in der Mechanik zur Aussagekraft des Force Concept Inventory. Zeitschrift für Didaktik der Naturwissenschaften 5:75-89.
Sherin, B.L. 1996. The Symbolic Basis of Physical Intuition: A Study of Two Symbol Systems in Physics Instruction. Dissertation. Available at http://www.sesp.northwestern.edu/docs/dissertation/386770764374ec34c2565.pdf; accessed on May 7, 2013.
———. 2001. How students understand physics equations. Cognition and Instruction 19(4):479-541.
Singh, C. 2001. Student understanding of quantum mechanics. American Journal of Physics 69:885.
———. 2005. Impact of peer interaction on conceptual test performance. American Journal of Physics 73:446-451.
Steinberg, R.N., and Sabella, M.S. 1997. Performance on multiple-choice diagnostics and complementary exam problems. The Physics Teacher 35:150-155.
Steinberg, R.N., Oberem, G.E., and McDermott, L.C. 1996. Development of a computer-based tutorial on the photoelectric effect. American Journal of Physics 64:1370.
Tuminaro, J., and Redish, E.F. 2007. Elements of a cognitive model of physics problem solving: Epistemic games. Physical Review Special Topics—Physics Education Research 3:020101.
Van Heuvelen, A. 1991. Learning to think like a physicist: A review of research-based instructional strategies. American Journal of Physics 59:891-897.
———. 1995. Experiment problems for mechanics. The Physics Teacher 33:176.
Van Heuvelen, A., Allen, L., and Mihas, P. 1999. Experiment problems for electricity and magnetism. The Physics Teacher 37(8):482.
van Weeren, J.H.P., de Mul, F.F.M., Peters, M.J., Kramers Pals, H., and Roossink, H.J. 1982. Teaching problem-solving in physics: A course in electromagnetism. American Journal of Physics 50(8):725-732.
Vokos, S., Shaffer, P.S., Ambrose, B.S., and McDermott, L.C. 2000. Student understanding of the wave nature of matter: Diffraction and interference of particles. American Journal of Physics 68:S42-S51.
Watkins, J., and Mazur, E. 2013. Retaining students in science, technology, engineering, and mathematics (STEM) majors. Journal of College Science Teaching 42(5).
Wright, D., and Williams, C.D. 1986. A WISE strategy for introductory physics. The Physics Teacher 24:211-216.
Yerushalmi, E., Henderson, C., Heller, K., and Heller, P., and Kuo, V. 2007. Physics faculty beliefs and values about the teaching and learning of problem solving, Part I: Mapping the common core. Physical Review Special Topics—Physics Education Research 3(2):020109.
Zeldin, A.L., and Pajares, F. 2000. Against the odds: Self-efficacy beliefs of women in mathematical, scientific, and technological careers. American Educational Research Journal 37:215-246.
Zollman, D.A. 1990. Learning cycles for a large-enrollment class. The Physics Teacher 28:20-25.
———. 1996. Millikan Lecture 1995: Do they just sit there? Reflections on helping students learn physics. American Journal of Physics 64:114-119.
Zollman, D.A., Rebello, N.S., and Hogg, K. 2002. Quantum mechanics for everyone: Hands-on activities integrated with technology. American Journal of Physics 70:252-529.
Zhu, G., and Singh, C. 2012. Surveying students’ understanding of quantum mechanics in one spatial dimension. American Journal of Physics 80(3):252-259.
























