Below is the uncorrected machine-read text of this chapter, intended to provide our own search engines and external engines with highly rich, chapter-representative searchable text of each book. Because it is UNCORRECTED material, please consider the following text as a useful but insufficient proxy for the authoritative book pages.
Free-Surface Effects on a Yawed Surface-Piercing Plate H. Maniar, I.N. Newman, H. Xu (Massachusetts Institute of Technology, USA) ABSTRACT Analytical and experimental results are presented for a thin vertical surface-piercing plate, moving in the plane of the free surface with constant velocity at a small angle of attack. The analytical development is a generalization of lin- ear thin-wing theory, using a normal dipole distribution on the plate and in the wake downstream of the trailing edge. A Kutta condition is imposed at the trailing edge. The linear free-surface boundary condition and far-field condition are satisfied by using for the dipole potential the transverse derivative of the classical ship-wave Green function for steady forward motion. Computations are performed for a rectangular planform with aspect ratio 0.5, and these are compared with experimental data. Re- sults are presented for the integrated force and moment distributed pressure, strength of the leading-edge singu- larity, and profile of the free surface alongside the plate. Attention is focused on a local nonuniformity at the intersection of the free surface and trailing edge. The nu- merical solution is not convergent at this point, with the Kutta condition imposed. Experiments are made to study this region. These show that there is a jump in the free surface across the wake behind the trailing edge, contrary to the assumed boundary conditions, but this jump exists only above a critical Froude number. The jump is accom- panied by a sharp transverse flow, contrary to the Kutta condition. 1. INTRODUCTION The classical model of a thin ship was introduced in wave-resistance theory by J. H. Michell, to represent the steady forward motion of a ship hull with transverse sym- metry. As in the analogous thickness problem of thin-wing theory, the solution can be constructed from a centerplane distribution of sources with local strength proportional to the longitudinal slope of the body. The thin-ship model has been used extensively for symmetric source-like appli- cations including the analyses of wave resistance in calm water, and seakeeping in head seas. 273 Relatively little work has been devoted to the lift- ing problem, where a thin body is yawed or cambered. This problem combines the fields of lifting surfaces and ship waves. Following the analogous lifting problem of a thin wing, a centerplane distribution of transverse dipoles, or an equivalent combination of vortices, can be used to represent the velocity potential. The kinematic boundary condition on the plate yields an integral equation over this surface for the unknown dipole moment. As in the thin- wing formulation a Kutta condition is appropriate at the trailing edge, and trailing vortices (or dipoles of moment independent of it:) must be distributed in the wake to sat- isfy the condition of pressure continuity across this surface. But unlike the usual thin-wing analysis, the dipole poten- tial must be generalized to satisfy the free-surface bound- ary condition; this substantially complicates the numerical analysis, and necessitates the development of special algo- rithms to achieve satisfactory results. From the practical standpoint several applications re- quire the solution of this problem. The most obvious are the yawed steady motion of a sailboat, which normally re- quires a side force to oppose the aerodynamic load on the sails. The same problem occurs in the yawed motion of a ship from the standpoint of maneuvering in calm water. Similar considerations apply to the struts on a hydrofoil vessel, and to the hulls of a SWATH or catamaran (which can experience lifting effects in straight motion without yaw due to the interactions between the hulls). In the unsteady generalization similar problems arise concerning the transverse modes of ship motions in waves. Finally, in an unlikely scenario which stimulated this investigation, the bow assembly of a ship was installed with the stem off- center, and questions arose subsequently concerning the hydrodynamic effects of this defect. It is appropriate to idealize this class of problems by considering the linearized case of a flat plate with rectan- gular planform. In this case the integral equation for the dipole moment was derived by Newman A, but lacking numerical results that work was never published. Sub- sequently in two independent studies Daoud [2] and Kern t34 attempted to obtain numerical results, but these efforts were not conclusive due to the limited computational re- sources of that time and the use of numerical integration
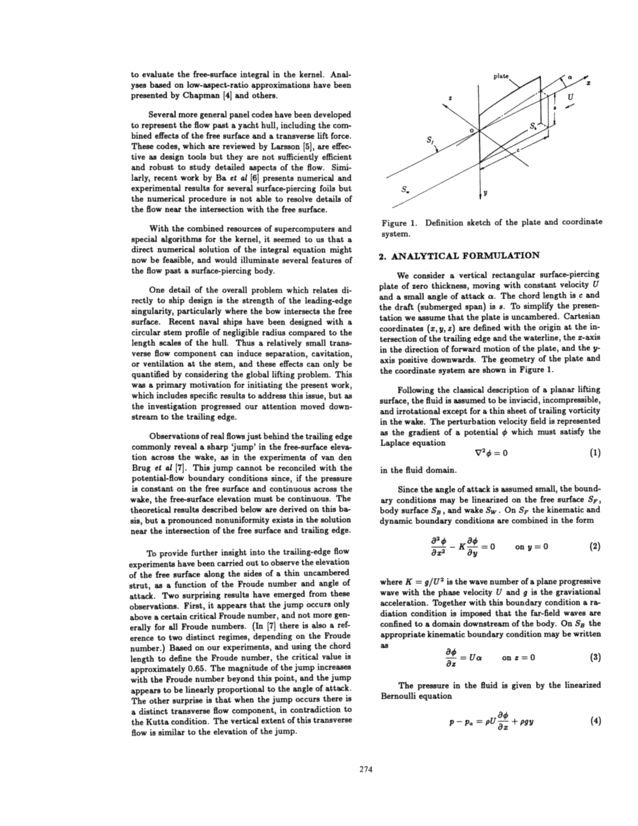
to evaluate the free-surface integral in the kernel. Anal- yses based on low-aspect-ratio approximations have been presented by Chapman [4] and others. Several more general panel codes have been developed to represent the flow past a yacht hull, including the com- bined effects of the free surface and a transverse lift force. These codes, which are reviewed by Larsson t5i, are e~ec- tive as design tools but they are not sufficiently efficient and robust to study detailed aspects of the flow. Simi- larly, recent work by Ba et al [64 presents numerical and experimental results for several surface-piercing foils but the numerical procedure is not able to resolve details of the flow near the intersection with the free surface. With the combined resources of supercomputers and special algorithms for the kernel, it seemed to US that a direct numerical solution of the integral equation might now be feasible, and would illuminate several features of the flow past a surface-piercing body. One detail of the overall problem which relates di- rectly to ship design is the strength of the leading-edge singularity, particularly where the bow intersects the free surface. Recent naval ships have been designed with a circular stem profile of negligible radius compared to the length scales of the hull. Thus a relatively small trans- verse flow component can induce separation, cavitation, or ventilation at the stem, and these effects can only be quantified by considering the global lifting problem. This was a primary motivation for initiating the present work, which includes specific results to address this issue, but as the investigation progressed our attention moved down- stream to the trailing edge. Observations of real flows just behind the trailing edge commonly reveal a sharp 'jump' in the free-surface eleva- tion across the wake, as in the experiments of van den Brug et al A. This jump cannot be reconciled with the potential-flow boundary conditions since, if the pressure is constant on the free surface and continuous across the wake, the free-surface elevation must be continuous. The theoretical results described below are derived on this ba- sis, but a pronounced nonuniformity exists in the solution near the intersection of the free surface and trailing edge. To provide further insight into the trailing-edge flow experiments have been carried out to observe the elevation of the free surface along the sides of a thin uncambered strut, as a function of the Froude number and angle of attack. Two surprising results have emerged from these observations. First, it appears that the jump occurs only above a certain critical Froude number, and not more gen- erally for all Froude numbers. (In t7; there is also a ref- erence to two distinct regimes, depending on the Froude number.) Based on our experiments, and using the chord length to define the Froude number, the critical value is approximately 0.65. The magnitude of the jump increases with the Froude number beyond this point, and the jump appears to be linearly proportional to the angle of attack. The other surprise is that when the jump occurs there is a distinct transverse flow component, in contradiction to the Kutta condition. The vertical extent of this transverse flow is similar to the elevation of the jump. i o S. ~ plated . ~ t1 I' Figure 1. Definition sketch of the plate and coordinate system. 2. ANALYTICAL FORMULATION We consider a vertical rectangular surface-piercing plate of zero thickness, moving with constant velocity U and a small angle of attack a. The chord length is c and the draft (submerged span) is s. To simplify the presen- tation we assume that the plate is uncambered. Cartesian coordinates (x, y, z) are defined with the origin at the in- tersection of the trailing edge and the waterline, the 1:-axis in the direction of forward motion of the plate, and the y- axis positive downwards. The geometry of the plate and the coordinate system are shown in Figure 1. Following the classical description of a planar lifting surface, the fluid is assumed to be inviscid, incompressible, and irrotational except for a thin sheet of trailing vorticity in the wake. The perturbation velocity field is represented as the gradient of a potential ~ which must satisfy the Laplace equation v24 = o (1) in the fluid domain. Since the angle of attack is assumed small, the bound- ary conditions may be linearized on the free surface SF' body surface SO, and wake Sw. On SF the kinematic and dynamic boundary conditions are combined in the form 2-K-= 0 on y = 0 (2) ~ BY where K = g/U2 is the wave number of a plane progressive wave with the phase velocity U and 9 is the graviational acceleration. Together with this boundary condition a ra- diation condition is imposed that the far-field waves are confined to a domain downstream of the body. On So the appropriate kinematic boundary condition may be written as BY = Ua on x = 0 (3) The pressure in the fluid is given by the linearized Bernoulli equation 274 p-Pa=PUB¢+PgY (4)
- - _ 1 ~ - - o a ~ ·7 c to a ~ ~ oo o.to onto free surface g O _ (6 X 6) panels (12 x 12) panels (24 x 24) panels (48 x 48) panels Figure 2. Results at a Etroude number F = 0.8 for the spanwise distribution of lift (C') and moment (Cm ). Symbols denote different discretizations of Sb with the indicated numbers of panel segments in the chordwise and spanwise directions, respectively. where Pa is the atmospheric pressure on free surface. Since the pressure must be continuous across the wake Sw, ex- tending downstream from the trailing edge, it follows that ~3 (~+ - 4-) = 0 (5) where superscripts denote the limiting values on z ~ 01. In accordance with the Kutta condition the velocity ~s assumed to be finite and continuous at the trailing edge. Finally, for large depths beneath the free surface, the ve- locity is assumed to vanish. From (4) the elevation of the free surface is given by IUB' t7f ~ A ~· (6) From (5) it follows that the free-surface elevation is con- tinuous across the wake. Since the only inhomogeneous boundary condition (3) implies a solution ~ which is odd in z, the free-surface elevation will vanish everywhere on the x-axis except along the two sides of the plate, where this elevation will be equal in amplitude and opposite in sign. Green's theorem can be used to reduce this boundary- value problem to an integral equation on SB. For this purpose we first define the Green function GFX,6) = r-r +H(X,() (7) where x-(x,y,z) is the field point, ~ = (6,,7,`) is the source point, and ~ and rO are, respectively, the distances between the field point and the source point or its image above the free surface: r = [(X-(~2 + (y_ ?7~2 + (z_ <~2]1/2 (8) r0 = t(X _ (~2 + (y + t7~2 + (z _ `~2lll2 The function H(X, (,) iS harmonic in the fluid domain, and will be defined subsequently to satisfy the free-surface boundary condition and radiation condition. If Green's theorem is applied to the control volume bounded by SF, SB, SW, and a closure at infinity, the only nonvanishing contribution is from the normal dipoles on SB U SW. The result is 41r /J(s~uS~ t+~71~° ~ ¢~,r1,o )] a dads (9) where SB+ and S+ denote the side of SB and Sw where z ~ 0+, and where [3G/~3n = ~G/3Z = -3G/~3~. Let- ting m((,,7) = ¢~(,'7,0+)-¢~(,'7,0-), equation (9) ex- presses the velocity potential in terms of a continuous nor- mal dipole distribution over the lifting surface and its wake with the unknown moment mum,. Differentiating both sides of this equation with respect to z, and imposing the boundary condition (3) on SB, the resulting equation may be written as 41r x - o it /~{s~ use mail t7) HIS | dude = Ua (10) This is a Fredholm integral equation of the first kind, to be solved for the unknown function mix, y). From the boundary condition (5) the unknown mix, y) is independent of x on Sw, m~x,y) = m(O,y) for-oo < x ~ O; O < y ~ s (11) Thus the unknown function m is confined to the domain of the lifting surface SB. Moreover, since the potential is continuous outside the surfaces SB and Sw, the difference in the potential between the two sides must vanish at the leading edge and the lower edge of SB. This gives the conditions mtc,y)=0 forO<y<s (12) mix, s) = 0 for O < x < c However, no similar conditions can be applied on the wa- terline. The Green function G is well known in ship-wave the- ory, and corresponds to the velocity potential of a sum 275
to o to of a (6 x 6) panels ~ (12 x 12) panels f. o (24 x 24) parcels + (48 x 48) panels C)= ' 0 90 1.00 ~.x o.,o 0 20 o.,o lower edge free surface 0.40 Figure 3. Spanwise distribution of the leading-edge singularity strength (C) and drag coefficient (C'`) for the same conditions described in Figure 2. merged source moving with constant horizontal velocity beneath the free surface. The following form, as given by Newman t8i, is convenient for numerical applications: 1 1 Gtx,6) =- -- r rO +Re[lim2i r cos add t°° e-`(Y+~)+ikl~-t| 8ec t4+k(x-A) tan ~ Jo k-K cos2 ~ + ie dk rnl2 + 4iH((-x) ~ sec2 ~ sin ~ - tr/2 e-8(Y+~1) sec '9+iK(:c-I) 8ec 19+iK|z-{ld. (13) Here H( f-z) is the unit step function, equal to 1 if ~ > x and 0 otherwise. The double integral in (13) represents a symmetrical nonradiating disturbance, and the single integral accounts for the wave field downstream. In the limits of zero or infinite Froude number the Green function reduces to (1/r ~ 1/rO), respectively. The solution of the integral equation (10) is then equivalent to that for the submerged plate plus its image above the free surface, in an otherwise unbounded fluid, where the angle of attack of the image is la respectively. Except for the Rankine singularity 1/r, which must be treated separately to account correctly for its limiting con- tribution on the boundary SB, the kernel of the integral equation (10) can be evaluated from the second normal derivative of G on the centerplane x = 0. From Laplace's equation the corresponding tangential derivatives can be used instead. Thus the values of the integrals in (13) are only required on the centerplane, where special algorithms described by Newman t8,9] are applicable. Following this approach the double integral in (13) is replaced by poly- nomial approximations, and the single integral is replaced by a pair of complementary Neumann expansions. Cor- responding analytic expressions have been derived for the second normal derivative of these integrals, and effective algorithms are described by Xu t10~. Using this approach the kernel in (10) can be evaluated without the computa- tional burden of numerical integration and with uniform accuracy of the results. (6 x 6) panels (12 x 12) panels o (24 x 24) panels + (48 x 48) panels - o.eo 0.90 l.X lower edge The single integral in (13) contains an essential sin- gularity along the x-axis, which corresponds physically to the short diverging waves with vanishing wavelength and unbounded amplitude downstream of a source in the free surface. Thus the limiting value of (13) is nonuniform for lx-id ~ 0, but if z-~ = 0 the value of the single integral is continuous as a function of the longitudinal and verti- cal coordinates. Thus when the source and field points are both restricted to the plane y = 0 the single integral can be evaluated without fundamental difficulties. On the other hand, the second derivative of the Green function does amplify a logarithmic singular component of the double integral when rO ~ 0. This singularity is weaker than the Rankine components 1/r and 1/rO, but it may be respon- sible for the nonuniform features of the solution at the intersection of the plate with the free surface. To obtain a numerical solution of the integral equa- tion (10) the domain SB is discretized in a similar manner to lifting-surface theory, generalizing the approach of Lan t11] with a nonuniform distribution of panels optimized to account for the square-root singularities at the leading edge and lower tip and for the more complicated behavior at the free surface. Further details regarding the analytic formulation and numerical analysis are given by Xu t10~. Standard algorithms [12] are used to evaluate the con- tribution from the Rankine singularities 1/r and 1/rO as distributions with constant density over each panel. The remaining contribution from the free-surface integrals is evaluated by one-point quadrature at the control point of each panel. The boundary condition is satisfied by collo ~4 3, ~ 276 . . . . . . em- - ~ Experimental data , ~-- Present results - Slender body approximation F f lgure 4. Comparison of the lateral-force coefficients at various Froude numbers. The experimental data is from van den Brug, et al (1971) and slender body approximation is due to Chapman (1976).
cation, leading to a system of N algebraic equations with the same number of unknowns. The contribution from the dipoles in the wake is evaluated by the same approach, with the wake discretized by rectangular panels and trun- cated at a sufficiently large distance downstream deter- mined so that the results are not affected. This scheme has been validated in the zero-Froude-number limit by comparison with numerical results in the aerodynamic lit- erature. The theoretical results shown here are for an aspect ratio equal to 0.5. This value was selected to permit com- parison with the experimental results of van den Brug A. Integrated quantities include the lift coefficient C1, yaw moment CM about the mid-chord point, drag coefficient CD, and the thrust coefficient CT from the leading-edge suction force. The lift force and yaw moment are evaluated directly by pressure integration over the discretized surface SB . The leading-edge suction force is derived by assuming the square-root singularity of the nondimensional pressure coefficient AC', = P. UP = U (P' Y) (14) to be of the form ACp ~ 2C(y)~/~ (15) near the leading edge. After integrating both equations with respect to x, C(y) = 2U~ km >fi~ (16) This limit is extracted by Aitken's extrapolation algo- rithm, and the sectional leading-edge thrust coefficient is evaluated from the relation Cty ~C2~' (17) Finally, the drag coefficient is derived from the rela- tion CD = CI,RX-CT. Note that CD is the total drag co- efficient including the sum of induced drag and wave drag. (Integration in the Treftz plane is not practical due to the difficulties of evaluating the wave field far downstream.) 1 ! ~ Aim of on 1 l . O 04) 0. t0 0 20 0.30 0 40 0 50 0 60 free surface Y (6 x 6) panels (12 x 12) panels (24 x 24) panels (48 x 48) panels I 0.70 O.BO 0.90 J.00 lower edge Figure 5. Spanwise distributions of pressure coefficients at the trailing edge for the same conditions aescr~oea In Figure 2. fir- _ . . _ .. . . Tests of numerical convergence have been made by comparing the results based on discretizations with 6 x 6, 12 x 12, 24 x 24, and 48 x 48 panels. Note that the latter computations involve a total of 2304 unknowns, and the solution of a linear system of equations with 2304 x 2304 = 5.3 x 106 coefficients to be evaluated from the derivatives of the Green function (13~. Convergence to two significant figures is achieved for the integrated force coefficients with the 24 x 24 = 576 discretization. Most of the computations with less than 1000 panels were performed on a VAX-750 computer. Those for larger numbers of panels were performed on a Cray-YMP. Results for the spanwise distribution of the lift and moment coefficients are shown in Figure 2. Figure 3 shows the spanwise distribution of the leading-edge singularity strength and drag coefficient. The leading-edge singularity vanishes at the free surface, since the pressure is constant on this surface. The other coefficients plotted in these two figures are all affected near the free surface by a local nonuniformity which manifests itself most strongly near the trailing edge, and is discussed further below. Figure 4 compares the total lift and moment coeffi- cients with the experimental data of van den Brug t7] and with the slender-body theory of Chapman [44. The re- sults appear to be satisfactory, with improved agreement from the present results compared to those of Chapman especially in the case of the moment. Near the trailing edge the solution is not convergent, as indicated in Figure 5. Here the pressure coefficient is derived from L'Hospital's rule in terms of the second derivative of m with respect to the angular coordinate ~ defined by the relation sing = 2//c. From the Kutta condition the pressure coefficient should vanish at the trailing edge, whereas the actual values computed in this manner are not precisely zero and the error appears to increase without limit as the free surface is approached from below. The perspectives in Figure 6 show in a revealing man- ner the complete pressure distribution on SB as a function of the Froude number. In the two limits of zero and infinity the result is well behaved near the trailing edge, and the same appears to be true for the Froude number F = 0.3, where the waves on the free surface are relatively short. However at F = 0.8 a local nonuniformity is apparent at the intersection of the free surface and trailing edge. This nonuniformity is confined to a few panels adjacent to the intersection point; as the number of panels is increased, the domain of the nonuniformity is reduced. To evaluate the free-surface profile along the intersec- tion of the plate, the unknown m must be differentiated numerically with respect to the horizontal coordinate x, and extrapolated vertically from the uppermost colloca- tion point to the free surface. The results are shown for various discretizations in Figure 7. For the case F = 0.3 a finer discretization has been used longitudinally, to ac- count for the shorter wavelength scale. Other results are shown later in conjunction with the experiments. 277
u ~ -~- Figure 6. Normalized pressure distributions on the plate, at the indicated Froude numbers. D (28 x 9) panels (56 x 18) panels (112 x 36) panels ~o o 11 ~o - ~- - ~ '0.00 0.10 0.20 1;, $.~. f F = 0.3 ,~' . . . . 0.60 0.70 0.60 0.90 I.W L.E. Figure 7. Free surface profile on the plate. Symbols denote different discretizations with the Indicated numbers of panel segments in the chordwise and spanwise directions. 278 o . , , ' 1 o . Q O -I · / ~ ~ ~ ~ ~ _ ~ (12 x 12) panes f o (24 x 24) panels {t + (48 x 48) panes o ~ o 0.00 O.lO 0.20 0.30 0.40 O.SO 0.60 T.E. 2 F=0.8 ! it, 1 . . . 0.70 O.W 0.90 L.E.
Figure 8. Side views of the experimental set-up and flow at an angle of attack c' = 6° and Froude number F = 0.69, just above the critical Froude number. In the left figure the angle of attack is such that the view is from the pressure side, and in the right figure from the suction side. The jump is indicated in the left figure by the bright area extending downstream from the trailing edge. Grid spacing is one inch, and the height of the jump is about 0.4 inches. 3. EXPERIMENTS Experiments have been carried out to observe the flow past a thin uncambered strut, mounted vertically and in- tersecting the free surface in the water tunnel of the MIT Marine Hydrodynamics Laboratory. This tunnel, which has a square cross section of 20 by 20 inches (0.51 x 0.51 meters), is normally used for tests of propellers and cap- tive bodies without a free surface. By lowering the water level it is possible to perform tests with a free surface, and relatively uniform inflow conditions can be achieved with a level free surface if the depth Froude number is less than approximately 0.5. The present tests were conducted at a nominal depth of 14 inches (0.35m), and at velocities up to 3.2 feet per second (1 m/s). Large windows on the sides and bottom of the tunnel facilitate observations of the flow. A suitable strut model with rectangular planform was constructed from aluminum plate, with chord 6.125 inches (156mm) and maximum thickness 0.25 inches (6mm). The profile was shaped to have an approximately parabolic leading-edge section, and a blunt trailing edge of thickness 0.04 inches (lmm). To facilitate observations a square grid with 1 inch spacing was established and threads were glued to the surface, as shown in Figure 8. The upper portion of the strut was attached to a vertical shaft which could be rotated to vary the angle of attack. The submerged span was adjusted by varying the elevation of the model and water depth, to obtain aspect ratios up to one. The inflow velocity was measured with a laser Doppler anemometer (LDA). Some efforts were made to use the LDA to survey the region near the trailing edge, and it was possible to conclude in this manner that there was no substantial separation. The principal measurements were visual and photo- graphic observations of the free-surface elevation on the two sides of the strut, which could be resolved to an accu- racy of about 1/16 inch (1.5mm). Near the leading edge a thin spray sheet makes it difficult to establish the free 279 surface elevation, and no attempt was made to record data over the forward 30~o of the chord. Measured values of the jump were based on the difference in elevation of the free surface on the two sides of the strut at the last grid line, 1/8 inch (3mm) upstream of the trailing edge. In the following discussion results are given in nondi- mensional form based on the chord length, and the inflow velocity is defined by the corresponding Froude number, restricted to a maximum value of 0.8 due to the depth ef- fect noted above. Except where otherwise noted the tests were conducted at an aspect ratio equal to one. Initial observations revealed that there exists a crit- ical Froude number FC ~ 0.65. For F < FC there is no observable jump, and the free surface is continuous across the wake downstream of the trailing edge. Conversely, a distinct jump exists if F > Fc. The height of the jump is nearly proportional to the angle of attack. Viewed from just below the plane of the free surface on the high- pressure side, as in the left photograph shown in Figure 8, the jump appears as a brightly illuminated triangular sur- face which extends 20-30% of the chord length downstream of the trailing edge. Viewed from the low-pressure side, as in the right photograph, the jump is apparent from the in- tersection point where the two sides of the jump cross and reverse their relative elevations in an oscillatory manner. Figure 9 shows the corresponding view from below. Most of the threads at the trailing edge are oriented in the expected manner to accord with the Kutta condition of smooth tangential flow past the trailing edge, but the uppermost two threads are sharply deflected indicating a pronounced cross-flow near the free surface. The vertical extent of this cross-flow is comparable to the jump height, increasing in proportion to the angle of attack. If the Froude number is increased gradually through the critical value FC, it is apparent that the jump is related primarily to a change in the flow on the low-pressure side of the strut. Figure 10 illustrates this regime by showing
~d ~ ~ ~ ~ ~ ~ ~ ~ ~ I; ~ ~ ~ ~ ~ ~ Figure 9. View from below in the same condition as Figure 8. Note the sharp deflection of the two uppermost threads at the trailing edge. a' L - or _ to U. MU o 0 1 it. E o _____. , 0.29 0.43 O.S7 0.7t O.8IS 1.00 a ~ ~ X/C Figure 10. Free-surface profiles on the pressure (upper) and suction (lower) sides of the plate at subcritical (0.63) and supercritical (0.74) Froude numbers, at an angle of attack ct = 6°. Elevation is normalized by the chord length and angle of attack. ~~~2~ ~ rid ~ ~ L to 1 ~ I . to 1 cb.oo - - . . 0.14 0.29 F = 0.63 - F = 0.69 - F = 0.74 · · ~ ~ 0.43 0.57 0.7t 0. - t.00 1 - x/c Figure 12. Difference in free-surface elevation between the two sides of the plate at the Froude numbers indicated, as a function of the chordwise position, aspect ratio 1.0, angle of attack 6°. cb.oo O. 14 0.29 0.43 0,57 0.71 0. - t.00 Aspect ratio Figure 13. Effect of aspect ratio on the jump height. Froude number = 0.74. °0,eo 0.68 O.7t _ . . 0.77 0.83 0. - 0. - t.00 froude number Figure 11. Normalized jump height versus Froude number for an aspect ratio 1.0. Height is normalized by the chord length. 280 '0.00 0.14 0," 0 43 0." 0.7t 0.0 t.00 ~ - X/C Figure 14. Comparison of computed and experimental free-surface elevation difference, aspect ratio 0.5, angle of attack 6°, Froude number 0.7.
separate free-~urface profiles on the two sides of the strut at Froude numbers close to the critical value. The eleva- tion of the free surface on the high-pressure side changes relatively slowly near Fc, but an abrupt shift occurs on the low-pressure side. For F < Fc there is a steep slope of the free surface on the low pressure side, with the el- evation rising to meet the flow from the opposite side at the trailing edge. Above the critical Froude number this effort is abandoned, in favor of independent flows on the two sides with more gradual slopes. Figure 11 shows the jump amplitude as a function of the Froude number. The tendency for the jump to vanish below a critical Froude number Fc is clear; the value of FC appears to be close to 0.65. The difference in elevation between the two sides of the strut for Froude numbers just below and above the critical value is shown in Figure 12. The difference is plot- ted here in order to remove the symmetric disturbance due to thickness. However for the supercritical case the nonsymmetric feature noted in connection with Figure 10 must be considered in attempting to distinguish between thickness and lifting effects. In Figure 13 the effect of aspect ratio on the jump elevation is plotted, indicating a nearly linear proportion- ality. Thus the jump elevation is nearly proportional to the span or draft, for aspect ratios less than one. In Figure 14, we compare the experimental and the- oretical values of the free-surface elevation difference as a function of position along the chord, for an aspect ratio equal to 0.5. The theoretical curve shown is for ~ = 0.7 whereas F-0.69 in the experiments. However other the- oretical results are similar at F = 0.6 and F-0.8, hence the small difference in Froude numbers is not significant to this comparison. The results are similar up to a point about 20% of the chord upstream of the trailing edge, with the calculations based on the theory 20-30% less than the experiments. Closer to the trailing edge, however, the two results are dissimilar. The experimental difference associ- ated with the jump remains positive, whereas the calcu- lated difference changes sign before reverting to the forced value of zero at the trailing edge. 4. CONCLUSIONS For a vertical surface-piercing lifting surface we have demonstrated that convergent numerical results can be de- rived for most parameters of practical importance. These include the integrated force and moment coefficients, and their spanwise distributions. Also included are calculated values of the leading-edge singularity which can be used to predict the occurrence of ventilation, cavitation, or sepal ration, and to design the stem radius of ships or surface- piercing struts on a rational basis to avoid these undesir- able phenomena. To obtain the present results special numerical tech- niques have been developed to evaluate the kernel of the integral equation, i.e. the second-derivative of the free- surface Green function, and the discretization of the lifting surface has been selected to improve the convergence rate. To demonstrate numerical convergence up to 4032 panels have been used. This level of computation is only feasible by using a supercomputer, but reasonable engineering ac- curacy can be achieved with 0~500) panels except for low Froude numbers where the wavelength is short. The numerical solution breaks down locally at the intersection of the trailing edge with the free surface. At this point the pressure distribution is singular, and it is unclear if this is the result of the numerical differentiation and extrapolation required to derive this parameter, or if there is a more fundamental cause. Special experiments have been conducted to study the flow near this singular point, and we find that a free- surface jump occurs when the Froude number exceeds a critical value Fc. For an aspect ratio of one FC ~ 0.65, based on the chord length. For aspect ratios in the range 0.25-1.0 the critical Froude number does not change sig- nificantly. For smaller values of the Froude number there is no evidence of a jump. In the supercritical regime where the jump occurs, a pronounced transverse velocity can be observed just behind the trailing edge, contrary to the Kutta condition. The subcritical regime with no jump appears to be compatible with the classical view of a vertical trailing vortex sheet in the wake which intersects the free surface. The boundary conditions of constant pressure on the free surface and continuous pressure across the vortex sheet require the free-surface elevation to be continuous across the wake. Extending this argument, the kinematic bound- ary condition on the free surface implies continuity of the vertical velocity component across the wale, and hence vanishing of the trailing vorticity on the free surface. On this basis the slope of the spanwise lift distribution should vanish at the free surface, as in the simpler limiting case of zero Froude number where a simple image solution applies. Our description of the supercritical regime is more speculative. If the jump is considered to be a vertical free surface, joined from below by the trailing vortex sheet, the pressure is continuous across the vortex sheet and hence the magnitude of the fluid velocity is the same on both sides of the sheet. This determines the velocity at the lower edge of the jump, and moving upward along the face of the jump the velocity is reduced to balance the hy- drostatic pressure. A discontinuity in the vertical velocity component across the wake is accommodated by the dif- ferent slopes of the free surface on the two sides of the jump, hence the trailing vorticity and slope of the span- wise lift distribution can be nonzero. The latter conditions must apply ultimately in the limit of infinite Froude num- ber, where the negative image solution implies that the spanwise lift is zero (but its slope is nonzero) at the free surface. A large negative pressure is required locally to offset the surface tension associated with the sharp curvature at the base of the jump, and this may explain the sharp cross flow just behind the trailing edge near the free surface. There is no indication that the jump observed in ex- periments is related to the numerical instability in the same region, which is present for lower E`roude numbers. 281
Moreover the numerical predictions of the free-surface ele- vation and pressure distribution near the free surface actu- ally change sign just ahead of the trailing edge, increasing the longitudinal gradient in a manner precisely opposite to the experimental flow. Since the computations are de- pendent on numerical differentiation and extrapolation, it is impossible to be definitive on this point. Further research is required to resolve these questions. We hope that independent experiments will be conducted to confirm and extend our observations, and to provide a more detailed velocity survey of the jump region. Further progress with the numerical analysis may also lead to a more robust algorithm for calculating the pressure coeffi- cient and free-surface elevation near the trailing edge. 5. ACKNOWLEDGMENT This work was supported by the Office of Naval Re- search, Contract N00014-88-K-0057. The computations with large numbers of panels were performed at the Pitts- burgh Supercomputer Center. The experiments were con- ducted in the water tunnel of the MIT Marine Hydrody- namice Laboratory. 6. REFERENCES 1. Newman, J.N., "Derivation of the Integral Equation for a Rectangular Lifting Surface," unpublished manu- script, 1961. 2. Daoud, N., "Force and Moments on Asymmetric and Yawed Bodies on a Free Surface," Ph.D. Thesis, 1973, Uni- versity of California, Berkeley. 3. Kern, E.C., '~Wave Effects of a free Surface Piercing Hydrofoil," Ph.D. Thesis, 1973, Massachusetts Institute of Technology. 4. Chapman, R.B., Free Surface Effects for Yawed Sur- face Piercing Plates," Journal of Ship Research, Vol. 20, No. 3, 1976, pp 125-136. 5. Larsson, L., Scientific Methods in Yacht Design," An- nual Review of Fluid Mechanics, Vol. 22, 1990, pp 349- 385. 6. Ba, M., Coirier, J., & Guilbaud, M., "Theoretical and Experimental Study of the Hydrodynamic Flow around Yawed Surface-Piercing Bodies," 2nd International Sym- posium on Performance Enhancement for Marine Appli- cations, Newport, RI, 1990. 7. van den Brug, J.B., Beukelman, W., and Prins, G.J., "Hydrodynamic Forces on a Surface Piercing Flat Plate," Report No. 325, Shipbuilding Laboratory, Delft Univer- sity of Technology, 1971. 8. Newman, J.N., `'Evaluation of the Wave Resistance Green Function: Part 1. - The Double Integral," Journal of Ship Research, Vol. 31, No. 2, 1987, pp 79-90. 9. Newman, J.N., "Evaluation of the Wave Resistance Green Function: Part 2. - The Single Integral on the Centerplane," Journal of Ship Research, Vol. 31, No. 3, 1987, pp 145-150. 10. Xu, H., Potential flow solution for a yawed surface- piercing plate," submitted for publication, 1989. 11. Lan, C.E., `'A Quasi-Vortex-Lattice Method in Thin Wing Theory," Journal of Aircraft, Vol. 11, No. 9, 1974, pp 518-527. 12. Newman, J.N., "Distributions of Sources and Dipoles over a Quadrilateral Panel," Journal of Engineering Math- ematics Vol. 20, 1986, pp 113-126. 282
DISCUSSION Arthur M. Reed David Taylor Research Center, USA I have two questions concerning this paper: (1) Due to the small size of the experimental fail, could you please comment on the issue of scale effects (surface tension and Reynolds Number) with regard to the Classical frauds where a jump in the free surface at the trailing edge occurs; (2) In the case of SWATH ships, we see that there is a jump at the trailing edge for IF ~ 0.3 - 0.35, where the SWATH strut aspect ratio is 0.10 - 0.15 and the Angle of attack" of the strut is due to the flow induced by the opposite strut and the two hulls. Please comment on the effect of aspect ratio on critical IF and critical IF for SWATH ships. AUTHORS' REPLY We only can speculate regarding the questions raised by Dr. Reed concerning the effects of surface tension, Reynolds number, and aspect ratio. Surface tension may be significant in balancing the pressure field, as noted in Section 4. However, we envisage no critical importance of this parameter with respect to the occurrence of a jump. A similar view applies to the Reynolds number. The aspect ratio may be more important; for small values of this parameter the draft, rather than the length, may be the most significant length scale (despite our observations to the contrary in a relatively narrow range). In this event it would be expected that the critical Froude number for a swath strut (based on length) would be lower than the value we observe, by a factor approximately equal to the square root of the aspect ratio. The cause of the cross-flow should not affect the existence of the jump, and thus the observations noted by Dr. Reed appear to be consistent with our own. We hope that other experimental observations will be made with thin lifting surfaces of larger dimensions to clarify these issues. DISCUSSION Theodore Wu California Institute of Technology, USA My sincere congratulations to Prof. Nick Newman and his coauthors for giving us such an interesting paper. In respect to the surface-tension effects that may be involved in this problem, I would like to report a demonstration of flow instability (seemingly with a hysteresis) made a couple of decades ago by a colleague of mine, Taras Kicenuik. Starting with a surface-piercing strut at yaw to a uniform stream of water, held steadily without flow separation from the strut, a mere scratching of the water surface with a fine musical wire across any upstream station would at once lead to a rapid ventilation along the suction-side of the strut down to its lower tip, thus opening up a long and deep pocket of air ventilation, which can be readily closed up with some manual patching of the free surface. (I will try to find if any technical report has been issued on this experimental observation.) ANCHORS' REPLY Professor Wu has recalled a closely-related experiment which illustrates the effect of hysteresis on ventilation. We assume that the Froude number and/or angle of attack in that work were substantially larger than in our own experiments. We saw no indication of ventilation in the indicated ranges of Froude number and angle of attack. We did not observe any signs of hysteresis with respect to the jump at the trailing edge, either in the context of changing the Froude number or the angle of attack, and when these parameters were fixed there were no indications of instability in the height of the jump at the trailing edge. 283