3
Exposure Assessment in Environmental Epidemiology
Exposure to chemical and physical agents in the environment can produce a wide range of adverse health consequences. Environmental epidemiology attempts to determine whether a hazard exists—that is, whether there is a causal relation between exposure to certain chemical or physical agents and adverse health effects—and to measure and characterize any causal relations (to assess the exposure-response relationship). Typically, a continuum between level of exposure and the size or probability of health effects is assumed. Emphasis is placed on characterizing the associations across the continuum, and quantitatively defining the relation is a central feature of the epidemiologic investigation. Assessment of exposure is then a crucial component of environmental epidemiologic research. The estimation of exposure in relation to health effects is frequently difficult, and it has generally received inadequate attention. However, a field of exposure assessment is emerging.
Exposure assessment for purposes of environmental epidemiology may differ from exposure assessment for site remediation, mitigation, control, and risk assessment. The differences are sometimes subtle but may have substantial impact on the conduct of studies and associated allocation of resources. Investigations for the purpose of risk assessment, for example, generally include information on the source and identity of chemical agents, the concentration of each toxicant in various media, and the toxicity of identified toxicants as defined in experimental studies. Mathematical modeling may be used to define breakdown, transport, and ultimate location as well as the potential health risk. Environmental epidemiology, on the other hand, is more often hypothesis-based research
that seeks to examine specific populations or communities to clarify the relation between health and physical, biologic, and chemical factors.
Volume 1 of this report (NRC, 1991a) described some limitations and problems in the quantitative estimation of exposure when the focus of a study is possible adverse consequences of chemical exposure from hazardous-waste sites. This chapter reviews some aspects of exposure assessment or analysis that are important in environmental epidemiology and that illustrate the central role of exposure assessment. This chapter also discusses opportunities to improve analysis of exposure.
The importance of exposure assessment has been underscored in several reports (NRC 1988, 1991a,b). An International Society of Exposure Analysis has been formed, and the Science Advisory Board of the Environmental Protection Agency (EPA) recommended that EPA develop a 5-year program on exposure assessment (EPA, 1988).
The National Human Exposure Assessment Survey (NHEXAS) is a federal interagency program to design and implement an exposure surveillance system for the US population. The overall goal is to obtain periodic and systematic measurements of population exposures to multiple chemicals, including data on important environmental media, pathways, and routes, so that we can accurately determine current status, document historical trends, and predict possible future directions for exposures to hazardous chemicals (Sexton, 1991; Sexton et al., 1995) The NHEXAS has 3 specific objectives: (1) to document the occurrence, distribution, and determinants of exposures to hazardous environmental agents, including geographic and temporal trends, for the US population; (2) to understand the determinants of exposure for potentially at-risk population subgroups, as a key element in the development of cost-effective strategies to prevent or reduce exposures (risks) deemed to be unacceptable; and (3) to provide data and methods for linking information on exposures, doses, and health outcomes that will improve environmental health surveillance, enhance epidemiologic investigations, promote development of predictive models, and ultimately lead to better decisions (Sexton et al., 1995). As pointed out by Burke and Sexton (1995), "NHEXAS represents perhaps the most comprehensive exposure surveillance initiative ever undertaken ... it has been designed to address the information needs of regulators and improve the scientific basis for risk assessment, risk management, and risk communication." The "consolidated report" of an EPA-appointed consensus team on NHEXAS concludes as follows: "The implementation of NHEXAS can be considered a turning point in environmental policy. It represents the first concerted effort to understand and track total individual exposures on a national scale'' (Burke et al., 1992). A complete description of the NHEXAS phase I field studies, as well as a summary of the rationale and justification for
NHEXAS was published in the Journal of Exposure Analysis and Environmental Epidemiology (1995; 5:229-444).
EPA has made a significant contribution to exposure assessment by the issuance of guidelines for exposure analysis (EPA, 1992). The guidelines describe general concepts of exposure assessment and have application to risk assessment, trends analysis, and epidemiology. Those guidelines and the NRC report Human Exposure Assessment for Airborne Pollutants (NRC, 1991b) are major contributions to the assessment of the impact of toxic agents on potentially exposed populations. They provide a broad overview of the need for exposure assessment. This chapter is not intended to repeat the contents of those documents, but will focus on certain specific issues in exposure assessment for use in environmental epidemiology.
Principal Concepts That Underlie the Content of This Chapter
It is relevant to state the assumptions that the committee used as the basis and context for this chapter.
- The effective application of exposure assessment methods may improve the results of any epidemiologic investigation. As in any line of epidemiologic investigation, an improvement in exposure assessment can reduce bias and improve statistical power to detect adverse effects associated with exposure to environmental contaminants.
- However, important findings may derive from environmental-epidemiologic investigation even when the exposure assessment uses only simple and crude tools to characterize the exposure of a given population. Overreliance on sampling of exposure of individuals may not be cost-effective and may limit the size of the study, with little improvement over the findings based on indirect methods.
Concepts and Methods in Exposure Assessment
This section reviews some of the basic concepts inherent in exposure assessment. For a more detailed discussion, the reader is referred to the NRC report Human Exposure Assessment for Airborne Pollutants (NRC, 1991b), the EPA guidelines for exposure assessment (EPA, 1992), and the Agency for Toxic Substances and Disease Registry (ATSDR) Guidance Manual (ATSDR, 1994). Epidemiological research uses various exposure metrics. The choice of a specific metric will depend on the type of study in question, the resources available to the investigator, the conceptual framework behind the investigation, and above all, biologic considerations. In deciding which exposure metric is best in a particular study,
one must be clear about basic concepts of exposure analysis. Exposure assessment for use in environmental epidemiology must attend to 5 primary issues: (1) the definition and characterization of the potentially exposed population; (2) the collection of quantitative information on population exposure, temporal characteristics, and dose-response relations; (3) the medium and the microenvironment of principal concern in terms of exposure; (4) the use of information collected in one population in assessing potential risk to others; and (5) the biologic plausibility of any hypotheses based on mechanistic considerations that can assist and help guide the exposure assessment.
ATSDR (1994) has developed a definition of exposure as "an event that occurs when there is contact at a boundary between a human being and the environment with a contaminant of a specific concentration for an interval of time; the units of exposure are concentration multiplied by time." NRC (1991b) and EPA (1992) have also developed definitions of potential dose, applied dose, internal dose, and biologically effective dose for purposes of exposure assessment. The terms are illustrated in figure 3-1. Potential dose is the amount of the chemical ingested, inhaled, or in material applied to the skin. Applied dose is the amount of a chemical that is absorbed or deposited in the body of an exposed organism. Internal dose is the amount of a chemical that is absorbed into the body and available for interaction with biologically significant molecular targets. Biologically effective dose is the amount of a chemical that has interacted with a target site over a given period so as to alter a physiologic function.
A concept important to any type of study is that of total exposure. Assessment of total exposure has received considerable attention in recent years (Lioy, 1990; NRC, 1991a; Wallace, 1991; Wallace et al., 1986, 1987, 1988). Total-exposure assessment consists of estimating possible exposure from all media (soil, water, air, and food) and all routes of entry (inhalation, ingestion, and dermal absorption). NRC (1991a) and Lioy (1990) have developed a conceptual framework for human total exposure assessment that may serve as a guide for assessing human exposure to environmental contaminants. The framework is outlined in table 3-1. This framework accounts for all exposures to a specific agent or group of agents that an individual may have had, regardless of the environmental medium. Total-exposure assessment has particular relevance in environmental epidemiology insofar as it facilitates identification of the principal medium or microenvironment of concern and provides information on potentially confounding exposures.
NRC (1991a,b) described the different measurement and estimation techniques used in exposure assessment. Categories were defined that include direct measurement of exposure (personal monitoring, biologic monitoring, and biomarkers); indirect measures (microenvironmental
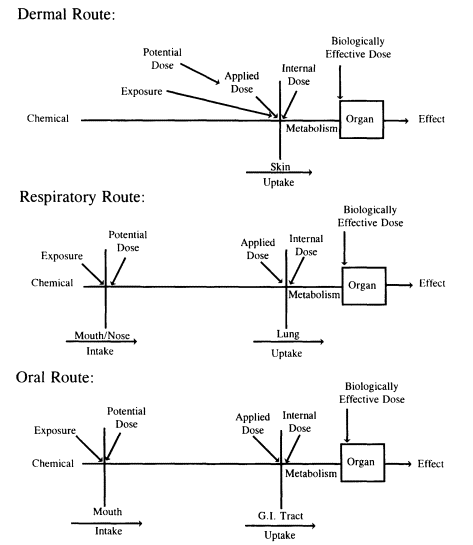
Figure 3-1
Schematic of dose and exposure.
monitoring coupled with exposure models, where microenvironmental monitoring is defined as the monitoring of contaminant concentrations in locations or media in which exposure occurs); that include mathematical modeling, questionnaire/diaries, or spatial factors, e.g., residence in a country or region or distance from a source of chemical contamination (figure 3-2). EPA (1992) has also provided examples of types of measure-
ments needed to characterize exposure-related media and associated parameters (table 3-2). Ryan (1991) has reviewed aspects of human exposure modeling that are useful for understanding the concept of exposure assessment.
When direct measurement of exposure is possible, it generally provides more-accurate information than indirect assessment of a particular individual's contact with a specific contaminant over time. The tradeoff is often between accurately measuring exposures over short periods, often outside the period of disease etiology, and indirect methods of assessing exposure over lengthier, more-relevant intervals. Personal monitoring has been widely used in workplace settings and can provide a measure of exposure across a range of microenvironments where individuals reside or work, though it is generally limited to a single chemical compound. However, personal sampling is often expensive, may demand extensive analytic capability and methodologies, and requires care in selecting study subjects. Biologic monitoring provides direct measures that integrate all routes of exposure to contaminants. Biologic monitoring may also provide more-precise estimates of target-organ dose, if appropriate toxicokinetic and metabolic information is available.
Indirect sampling uses exposure data available for defined areas or other microenvironments, generally from monitoring with time-activity information. Exposures in each microenvironment are weighted for the average time spent there and added to assess total personal exposure. Validation of the specific applicability of indirect monitoring is an important requirement for the successful use of this method. Some important epidemiologic studies have emphasized indirect measures of exposure as the primary linkage to health outcome. Some of these studies are reviewed below.
Exposure-Data Needs for Epidemiology Studies
NRC (1991a) has discussed exposure assessment in relation to the type of study being conducted: "The type of exposure assessment and the acceptable level of uncertainty in the data vary according to whether the assessment is designed to generate or test hypotheses about exposure, test instruments, make risk assessment decisions, or make regulatory decisions." Gann (1986) has asked, "What kind of exposure data do epidemiologists need?" He argues that the answer depends on the development of a well-defined research question. Bailar (1989) points out that the definition of dose-response relations is usually critical to establishing causality. It is expected that, when it is possible to examine ordered categories of exposure, higher doses will have greater effects so that "the dose-response relationship is monotonic.'' Thus, "departures from mono-
TABLE 3-1 Parameters Required to Calculate Potential and Internal Dose
|
Airborne contaminant |
Water contaminant |
|
I. Concentrations (µg/m3,ppb) |
I. Concentration (µg/L,ppm) |
|
A. Microenvironments |
A. Tap water |
|
B. Personal |
B. Water uses |
|
|
C. Effluent |
|
II. Patterns of exposure |
1. Industrial |
|
A. Intensity "episode" concentrations versus normal levels (average) |
2. Commercial |
|
|
3. Residential |
|
B. Frequency and duration of contact |
4. Uncontrolled dumps |
|
III. Transport |
II. Patterns of exposure |
|
A. Dispersion and advection |
A. Drinking |
|
B. Other meteorology related to removal rates (washout, fallout) |
B. Swimming |
|
|
C. Cooking |
|
C. Indoor ventilation and removal rates |
D. Bathing |
|
|
E. Laundry |
|
IV. Chemistry |
F. Showering |
|
A. Formation rates |
|
|
B. Transformation rates |
III. Solubility of contaminant |
|
V. Deposition rate (µg/cm2) |
IV. Volatility of contaminant |
|
A. Environmental |
|
|
B. Lung |
V. Transport |
|
|
A. Groundwater |
|
VI. Contact |
B. Surface water |
|
A. Inhalation (dependent on exercise regime)(m3/time) |
C. Domestic supply |
|
B. Dermal deposition and permeability (µg/cm2/time) |
VI. Chemistry |
|
|
A. Formation rates |
|
C. Ingestion (food, soil)(µg/g/time) |
B. Transformation rates |
|
|
C. Degradation |
|
VII. Absorption |
|
|
A. Within tissue |
VII. Contact rate (µg/L/time) via exposure route |
|
B. Into the blood and other fluids |
|
|
|
A. Ingestion |
|
|
B. Skin |
|
|
C. Inhalation (volatilized) |
|
|
VIII. Absorption |
|
|
A. Dermal deposition and permeability |
|
|
B. Gastrointestinal tract |
|
Source: Reprinted with permission from Lioy, 1990. Copyright 1990 American Chemical Society. |
|
|
Soil and sediment |
Food (commercial and homegrown produce) |
|
I. Concentrations (µg/g) |
I. Concentrations (µg/g) |
|
A. Dusts |
A. Plants |
|
1. Outdoor |
B. Vegetables and fruit |
|
2. Indoor |
C. Milk |
|
B. Contaminated soil |
D. Animals and fish |
|
1. Uncontrolled dumps |
E. Cooked foods |
|
3. Landfills |
F. Beverages and water-based foods (µg/L) |
|
2. Airborne deposition |
|
|
4. Resuspension |
|
|
|
II. Patterns of exposure |
|
II. Patterns of exposure |
A. Rate (µg/L/time) (µg/g/time) |
|
A. Frequency and duration |
B. Frequency |
|
B. Intensity of contact |
C. Origin of food |
|
|
1. Homegrown |
|
III. Percolation rate |
2. Commercial distribution |
|
A. Soil composition |
3. Local farms |
|
B. Water table |
4. Processed foods |
|
C. Solubility |
|
|
D. Transport |
|
|
|
III. Source of contamination |
|
IV. Volatilization |
A. Naturally occurring contaminants |
|
A. Contaminant |
B. Airborne deposition |
|
B. Soil composition |
C. Fertilization |
|
C. Top soil and cover |
D. Pest control |
|
|
E. Waste dumps |
|
V. Contact rate via exposure route |
F. Water supply |
|
A. Dermal deposition and permeability |
G. Preparation and cooking techniques |
|
B. Lung |
|
|
C. Gastrointestinal tract (pica) |
IV. Contact rate |
|
1. Population |
A. Gastrointestinal (GI) |
|
2. Abnormal ingestion behavior |
B. Inhalation (cooking only) |
|
VI. Body parameter |
V. Absorption through GI tract |
|
A. Lung volume |
|
|
B. Exposed skin surface (condition of skin) |
|
|
VII. Absorption |
|
|
A. Soil composition |
|
|
B. Contact and absorption rates |
|
|
All media: Can be supplemented by measuring a biological marker of accumulated single-medium or multimedia exposures in blood, urine, feces, and so forth. Many of these usually are nonmedia specific. Body weight: Used for lifetime exposure and dose calculation. |
|
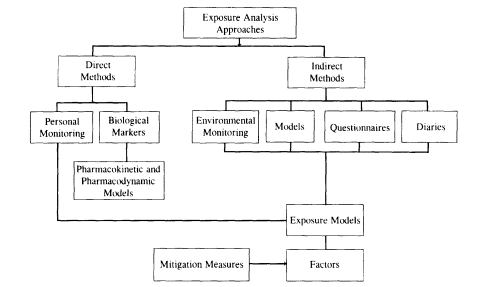
Figure 3-2
Possible approaches for exposure assessment.
tonicity raise questions about causality." Therefore, a key purpose of exposure assessment is often to support evaluation of dose-response relations.
Descriptive Epidemiologic Studies
Exposure data for descriptive epidemiologic studies must fairly apply to the population from which the disease is arising. This requires estimation of the whole probability distribution of exposure, not just means, with special attention to accurate estimation of the upper end of exposures. This is especially important if many or most persons are thought to be exposed at levels below some "threshold" where effects first appear. This is best achieved by random sampling, and sampling theory will dictate the nature and size of the sample, as well as any repetitions over time or circumstance, from which exposure will be assessed.
Unless exposure information over time is already available or obtainable, data from an investigation that measures exposure "now" must be used to infer exposure levels at earlier times, e.g., the times when cases of the disease were induced. If the distribution of induction times is not known, the use of current exposures may be highly uncertain.
In some instances, disease rates in an exposed population are compared with disease rates in unexposed population units, but inferences are stronger if the investigator classifies risk by some population grada-
tion of exposure. Sometimes, if exposure is measured in only a few areas, analysis may have to be limited to simple "exposed-control" comparisons. Sometimes, as may often happen in studies of pollutants in air or diet, there are no unexposed population units, and low-(or lower-) exposure groups fulfill the role of a "baseline" group.
The sophistication of the exposure information collected will in general depend on resources. Environmental modeling may be necessary to deal with area and temporal variation. Analytic methods to cope with these problems are discussed in chapter 6.
Analytic Epidemiologic Studies
The types of analytic studies used in environmental epidemiology were summarized in chapter 2. Here the particular exposure requirements of these studies are considered.
Case-control Studies
In case-control studies, the past exposures of cases and controls will have to be estimated, using historical records, if available, or current exposure measurements extrapolated back in time. Quantitative measures of exposure can reduce misclassification and allow the development of a dose-response curve.
Cohort Studies
Requirements for historical cohort studies are similar to those for case-control studies. For prospective cohort studies, there may be a need to estimate the extent of continuing exposure. In view of the large numbers required for cohort studies, resource constraints may make it impossible to do more than measure current or recent exposure.
As we pointed out previously (NRC, 1991a), the identification of potentially hazardous exposures in a group often results in cessation of exposure. This does not remove the need for characterization of past exposures.
Nested Case-control and Case-cohort Studies
Sometimes in a cohort study it is possible to collect specimens that could characterize exposure (e.g., biologic markers), where the expense largely resides in the analysis rather than specimen collection. When stored specimens are available (see NRC, 1991c, for a discussion of quality assurance associated with specimen archives) and can be analyzed after
TABLE 3-2 Examples of Types of Measurements to Characterize Exposure-Related Media and Parametera
|
Type of measurement (sample) |
Usually attempts to characterize (whole) |
|
A. For Use in Exposure Scenario Evaluation: |
|
|
1. Fixed-Location Monitoring |
Environmental medium; samples used to establish long-term indications of media quality and trends. |
|
2. Short-Term Media Monitoring |
Environmental or ambient medium; samples used to establish a snapshot of quality of medium over relatively short time. |
|
3. Source Monitoring |
Release rates to the environment from sources (facilities). Often given in terms of relationships between release amounts and various operating parameters of the facilities. |
|
4. Food Samples (also see #11 below) |
Concentrations of pollutants in food supply. |
|
5. Drinking Water Samples |
Concentrations of pollutants in drinking water supply. |
|
Type of measurement (sample) |
Examples |
Typical information needed to characterize exposure |
|
A. For Use in Exposure Scenario Evaluation: |
||
|
1. Fixed-Location Monitoring |
National Stream Quality Accounting Network (NASQAN),b water quality networks, air quality networks. |
Population location and activities relative to monitoring locations; fate of pollutants over distance between monitoring and point of exposure; time variation of pollutant concentration at point of exposure. |
|
2. Short-Term Media Monitoring |
Special studies of environmental media, indoor air. |
Population location and activities (closely matched to variations in concentrations); fate of pollutants between measurement point and point of exposure; time variation of pollutant concentration at point of exposure. |
|
3. Source Monitoring |
Stack sampling, effluent sampling, leachate sampling from landfills, incinerator ash sampling, fugitive emissions sampling, pollution control device sampling. |
Fate of pollutants from point of entry into the environment to point of exposure; population location and activities; time variation of release. |
|
4. Food Samples (also see #11 below) |
FDA Total Diet Study Program,c market basket studies, shelf studies, cooked-food diet sampling. |
Dietary habits of various age, sex, or cultural groups. Relationship between food items sampled and groups (geographic, ethnic, demographic) studied. Relationships between concentrations in uncooked versus prepared food. |
|
5. Drinking Water Samples |
Ground Water Supply Survey,d Community Water Supply Survey,e tap water. |
Fate and distribution of pollutants from point of sample to point of consumption. Population served by specific facilities and consumption rates. For exposure due to other uses (e.g., cooking and showering), need to know activity patterns and volatilization rates |
|
Type of measurement (sample) |
Usually attempts to characterize (whole) |
|
6. Consumer Products |
Concentration levels of various products. |
|
7. Breathing Zone Measurements |
Exposure to airborne chemicals. |
|
8. Microenvironmental Studies |
Ambient medium in a defined area, e.g., kitchen, automobile interior, office setting, parking lot. |
|
9. Surface Soil Sample |
Degree of contamination of soil available for contact. |
|
10. Soil Core |
Soil including pollution available for groundwater contamination; can be an indication of quality and trends over time. |
|
11. Fish Tissue |
Degree of contamination of edible fish tissue. |
|
B. For Use in Point-of-Contact Measurement |
|
|
1. Air Pump/ Particulates and Vapors |
Exposure of an individual or population via the air medium. |
|
Type of measurement (sample) |
Examples |
Typical information needed to characterize exposure |
|
6. Consumer Products |
Shelf surveys, e.g., solvent concentration in household cleanersf |
Establish use patterns and/or market share of particular products, individual exposure at various usage levels, extent of passive exposure. |
|
7. Breathing Zone Measurements |
Industrial hygiene studies, occupational surveys, indoor air studies. |
Location, activities, and time spent at monitoring locations. Protective measures/avoidance. |
|
8. Microenvironmental Studies |
Special studies of indoor air, house dust, contaminated surfaces, radon measurements, office building studies. |
Activities of study populations relative to monitoring locations and time exposed. |
|
9. Surface Soil Sample |
Soil sampling at hazardous waste sites. |
Fate of pollution on/in soil; activities of potentially exposed populations. |
|
10. Soil Core |
Soil sampling at hazardous waste sites |
Fate of substance in soil; speciation and bioavailability, contact and ingestion rates as a function of activity patterns and age. |
|
11. Fish Tissue |
National Shellfish Surveyg |
Relationship of samples to food supply for individuals or populations of interest; consumption habits; preparation habits. |
|
B. For Use in Point-of-Contact Measurement |
||
|
1. Air Pump/ Particulates and Vapors |
TEAM study,h carbon monoxide,i Breathing zone sampling in industrial settings. |
Direct measurement of individual exposure during time sampled, including relationships between individual and the population. |
|
|
|
Exposures, relationships between concentrations at times sampled and at other times and relationship between sampled individuals and other populations. To make these links, activities of the sampled individuals compared to populations characterized are needed in some detail |
|
Type of measurement (sample) |
Usually attempts to characterize (whole) |
|
2. Passive Vapor Sampling |
Same as above |
|
3. Split Sample Food/ Split Sample Drinking Water |
Exposures of an individual or population via ingestion. |
|
4. Skin Patch Samples |
Dermal exposure of an individual or populations. |
|
C. For Use in Exposure Estimation from Reconstructed Dose: |
|
|
1. Breath |
Total internal dose for individuals or population (usually indicative of relatively recent exposures). |
|
2. Blood |
Total internal dose for individuals or population (may be indicative of either relatively recent exposures to fat-soluble organics or long-term body burden for metals). |
|
3. Adipose |
Total internal dose for individuals or population (usually indicative of long-term averages for fat-soluble organics). |
|
4. Nails, Hair |
Total internal dose for individuals or population (usually indicative of past internal exposure in weeks to months range; can sometimes be used to evaluate exposure patterns) |
|
5. Urine |
Total internal dose for individuals or population (usually indicative of elimination rates); time from exposure to appearance in urine may depend on chemical |
|
|
|
Examples |
Typical information needed to characterize exposure |
|
Same as above |
Same as above. |
|
TEAM studyj |
Same as above. |
|
Pesticide Applicator Surveyk |
Same as above; Skin penetration. |
|
Measurement of volatile organic chemicals (VOCS), alcohol. (Usually limited to volatile compounds.) |
Relationship between individuals and population; exposure history (i.e., steady-state or not), pharmacokinetics (chemical halflife), possible storage reservoirs within the body, relationship between breath content and body burden. |
|
Lead studies, pesticides, heavy metals (usually best for soluble compounds, although blood lipid analysis may reveal lipophilic compounds.) |
Same as above; relationship between blood content and body burden. |
|
NHATSF,l dioxin studies, PCBs (usually limited to lipophilic compounds.) |
Same as above; relationship between adipose content and body burden. |
|
Heavy metal studies (usually limited to metals). |
Same as above; relationship between nails, hair content and body burden. |
|
Same as above; relationship between urine content and body burden. |
those subjects have been followed long enough to classify them as cases or controls, precision almost as great as in the full cohort study may be obtained. The case-cohort design can allow similar efficiency in exposure assessment, often at far less cost than a full-scale cohort study.
Issues in Exposure Assessment
Risk Assessment and Environmental Epidemiology
Better measures of exposure are clearly desirable in epidemiology. Exposure data exist on a continuum ranging from measures of emission, to measures of ambient concentration, to microenvironmental measures weighted by time spent in each environment, to personal monitoring, to measures of internal dose and biomarkers. Pirkle et al. (1995) provide a summary with examples of 6 different uses of biomarker data: to identify priority exposures, to evaluate effectiveness of risk-mitigation strategies, to identify at-risk subpopulations, to recognize trends, to establish reference ranges, and to provide integrated dose measurements. Several of these measures may be available to evaluate a given exposure. Activity patterns may also allow extrapolation from microenvironmental data to aggregate risk, though with some additional error. While time-activity studies can often model the mean dose delivered to a population with reasonable accuracy, it is more difficult to predict accurately the distribution, or even the variance, about that mean. Epidemiologic studies that associate the ambient pollutant directly with the outcome inherently incorporate the distribution of population activity patterns.
More-accurate exposure assessment can increase the power of an environmental-epidemiology study to find an association. However, increased precision is likely to be more costly, and better information for each subject may reduce the number of subjects who can be studied. This tradeoff between sample size and precision per subject means that statistical power will not necessarily increase monotonically with improvements in the accuracy of the exposure assessment. Spending additional resources to obtain better measurements of risk factors other than the pollutant of interest may also increase the power of the study by reducing misclassification of confounders and modifiers, but the additional costs are another set of tradeoffs. For any given budget, the optimal tradeoff is usually not knowable in advance, though studies of multifactorial outcomes and low relative risks almost always require large sample sizes. This need for a large sample puts a premium on using inexpensive methods, such as questionnaire data on activity patterns and other exposure-modifying behavior, rather than expensive but more-accurate methods.
Measurement Error
The term measurement error is different from exposure misclassification because the former implies a continuous variable, while the latter suggests a dichotomous one. Exposure to environmental toxicants is a continuous variable in the real world, and one of the most important improvements in exposure assessment is for studies to move from dichotomizing exposure into continuous, or at least multilevel, measurements.
For example, consider a study relating air pollution to respiratory illness. The outdoor ambient-air pollution concentration is available from community monitoring. The analysis of these data will seek to correlate variations in air pollution with variations in respiratory outcome. Variations in recorded levels of air pollution may be thought of as having the following components: measurement error associated with the monitoring instrument, variation in the amount of time individuals spent outdoors, geographic variation in the outdoor concentration of the pollutant in the vicinity of the monitor, variations in the indoor/outdoor ratio, and individual variations in delivered dose. Critical issues include the size of each error component and the cost of reducing each component.
Misclassification
One of the most important advantages of improved exposure assessment derives from its impact on misclassification. Small errors in exposure assignment may have dramatic results on estimation of effect. Because of the limited scope of exposure assessments in most environmental epidemiology, misclassification is likely to be a substantial problem. In general, the internal validity of an epidemiologic investigation can be reduced by misclassification of study subjects. Copeland et al. (1977) emphasize that bias from misclassification will be ''a function of the sensitivity and specificity of the classification procedure, the disease frequency, and exposure frequency." And in all case-control studies the bias depends on whether misclassification is the same or different in cases and controls, that is, nondifferential or differential in cases and controls.
In general, nondifferential misclassification causes measures of effect to be biased toward the null value. Such misclassification produces an underestimate of the effect, whereas differential misclassification can result in bias either toward or away from the null value. Copeland et al. (1977) argue that classification errors cannot be ignored and that investigators should attempt to estimate the magnitude of the errors. Dosemici et al. (1990) have shown that the predominant view that "nondifferential misclassification of exposure can only bias an estimate of a true positive odds ratio downward and not away from or beyond the null value" may
sometimes be wrong. These authors acknowledge that the problem they identify is not common, but they do suggest that caution is needed in interpreting results in the presence of nondifferential misclassification. (See Sexton et al., 1995, for a discussion of problems of misclassification associated with exposure measurement.)
The Need for Improvement in Exposure Assessment
Better measures of exposure can improve the ability of a study to assess adverse effects from environmental agents. Such improvements lead to an increase in the power of the study and reduction in bias, but also to increased cost. The health outcomes and exposure analysis must be considered together to arrive at a balanced prioritization of study requirements.
A wide array of exposure-assessment tools is available to the epidemiologic investigator, ranging from personal monitoring to the use of diaries or other indirect means. All the tools have potential value when used logically and reasonably. A continuing dialogue is necessary between scientists whose emphasis is on exposure assessment and epidemiologic investigators. In this regard, Lioy (1991a) has stressed the need for continued dialogue to focus on critical questions that will reduce ambiguity in terminology and conceptual design and improve the experimental design of both health and exposure studies. The development of the EPA guidelines on exposure assessment (EPA, 1992) and NHEXAS (Burke et al., 1992) should stimulate further discussion on these issues.
Landrigan (1983) has commented on the advantages of improved exposure characterization by using individual versus grouped data in a study of the health effects of arsenic in drinking water. The average concentration of arsenic in well water was a poor indicator of individual exposure because some of the persons studied had supplemented their consumption or changed completely to drinking bottled water. When estimates of bottled water consumption were incorporated into individual-exposure assessment, the dose-effect relationship was strengthened.
Kennedy et al. (1991), in a cross-sectional study of pulp and paper workers exposed to chlorine gas, found no differences from workers in other industries. However, when those pulp and paper workers who had an acute gas exposure were considered, symptom and forced-expiratory-volume (FEV1 and FEV25-75) differences were found. The authors concluded that accidental chlorine or chlorine dioxide exposures in pulp mills are associated with increased respiratory symptoms and airflow obstruction, particularly among nonsmokers and former smokers.
Monster and Smolders (1984) studied teachers and pupils at a kindergarten near a factory with emissions of tetrachloroethane. The levels of
tetrachloroethane in the air exhaled by teachers and school-children were significantly greater than in a control group. The study demonstrated the applicability of biologic monitoring, where the exposure derives from the environment instead of the workplace.
Levine et al. (1985) monitored air at a hazardous-waste remedial-action site. This study demonstrated that, when workers remained in fixed job locations, "occupational inhalation exposure monitoring must consider contaminants generated upwind of the job location." Biologic monitoring would have been useful in this population of workers.
The selection of individual monitoring versus area or population monitoring of exposure is a matter of continuing concern. In particular, overreliance on "central-site monitoring" for assessment of exposure to air pollutants may be undesirable because of the possibility that personal exposures may differ from those estimated by central-site monitoring. Unrecognized variation among individuals in true air-pollution exposure contributes to variability among individuals in estimated slopes. While important findings have derived from air-pollution studies that make use of ambient-air monitoring data, investigations of more-precise questions such as determinants of variations in response may be improved through the use of personal monitors that are now becoming available.
The improved accuracy of personal monitoring of exposure generally comes at the expense of a substantial increase in cost and may therefore have its greatest value in the validation of other, less-expensive models of exposure. Freeman et al. (1991) have developed a location and activity log for assessing personal exposure to air pollutants and conducted pilot studies to validate the approach. Schwab et al. (1991) have suggested that the use of self-reported exertion levels in time-activity diaries has useful application to exposure assessment, particularly with respect to estimating the relation between exposure assessment and dose assessment. More studies of this nature are needed. The value of time-activity logs has been illustrated in detail by Sexton and Ryan (1989).
New instruments with the sensitivity and specificity necessary to conduct personal air-monitoring exposure assessments are also needed, and there have been a number of new developments of sampling instruments. Many of these instruments appear to be reasonable in cost (NRC, 1991b).
Hasabelnaby et al. (1989) used microenvironmental monitoring to characterize the exposure of preadolescent children to fine-particle air pollution. The authors concluded that microenvironmental monitoring is useful for estimating personal exposure in preadolescent children because they generally do not smoke and are not exposed occupationally. They also spend statistically significant periods at home, in school, or outdoors near their homes. These authors used data from the Six Cities Study to obtain more-accurate measures of exposure to passive smoking. They
included detailed information about a range of possible sources of exposures to environmental tobacco smoke. With the refined models developed in the study, a more-precise relation was identified between lung function and environmental tobacco smoke. This work illustrates the efficacy of using a population subset with improved exposure assessment in order to improve the sensitivity of the investigation.
Peters (1991) has conducted an epidemiologic investigation to identify the chronic effects of ambient air pollutants in southern California. The design for this 10-year study emphasizes exposure assessment. This emphasis is particularly warranted because of the magnitude of the health concerns and the potential cost of controls. The study is in 3 phases. Phase I considers the resources available to determine spatial and temporal patterns of pollutants, identify the locations that allow discrimination of pollutants, develop a sampling strategy, and determine cost-effective methods for estimating personal exposure. Phase II will consist of a cross-sectional study of health outcomes that can be related to personal exposure data. Five comparison groups are to be compared by their exposure to ozone, acid, and particles in varied exposure categories. Children will be the subject population. In phase III, the investigators will follow successive cohorts through either the duration of the study or high-school graduation. Changes in pulmonary function and incidence of disease will be compared with the exposures that have been assessed prospectively. This study will have a detailed exposure assessment for use in the evaluation of health effects associated with air-pollutant exposure. It will permit some estimation of the value of extensive exposure characterization in terms of both cost-resource use and the ability to define causal factors and dose-response relations in air-pollution studies.
Lioy et al. (1992) and Stern et al. (1992) have investigated population exposure to chromium waste, with particular reference to residential exposure. Lioy et al. sought to identify microenvironments that can lead to important chromium exposure. Chromium levels were determined for indoor air, outdoor air, and house dust. Surface dust was identified as the best index of potential chromium exposure. This study illustrates the role of total-exposure monitoring in the selection of exposure media for an epidemiologic study. Stern et al. (1992) compared chromium levels in urine with exposures estimated by environmental monitoring. The authors found an association between chromium in household dust and urine levels of chromium that was consistent with residential exposure to chromate-production waste. These 2 papers are excellent models of a systematic approach to exposure assessment.
Investigators from the National Cancer Institute have investigated methodologic issues in exposure assessment for case-control studies of cancer and herbicides-pesticides (Blair and Zahm, 1990a,b, 1992; Brown et
al., 1991). These authors conclude that improvements in exposure assessment are necessary if epidemiologic investigations are to provide reliable information on the relations between cancer incidence and pesticide exposure. These studies are noteworthy in their attempt to identify problems of reliability and validity of exposure assessments in case-control studies of cancer and pesticide exposure. For example, Brown et al. (1991) compared interview data from farmers with data from their wives or other surrogates and found excellent agreement between direct and surrogate interviews regarding the use of specific pesticides.
Blair and Zahm (1990a) concluded their study by stating, "Exposure misclassification undoubtedly occurs. Most errors from misclassification, however, are likely to be nondirectional in nature and would bias risk estimates toward the null and dilute exposure-response relationships. Methodologic investigations are needed to evaluate the reliability of current exposure assessment procedures and to develop new resources to improve assessment techniques." These recommendations are valuable, but it seems more likely that new resources are needed to enhance the best use of existing but underutilized techniques. These recommendations further illustrate the need for greater interaction between epidemiologists who recognize the importance of exposure assessment and investigators whose area of emphasis is exposure determination.
Air-Pollution Studies and Exposure Assessment
For more than 4 decades, researchers have studied the impact of air pollution, most notably respirable particles and other priority pollutants, on human health. Carefully designed studies have provided a wealth of important information about relations between ambient exposures and adverse health consequences. A brief review of some of these studies illustrates the importance of microenvironmental monitoring and indirect measures of exposure characterization.
Studies by Abbey et al. (1991), Euler et al. (1987, 1988), and Mills et al. (1991) illustrate the benefit of ambient-air monitoring in epidemiologic characterization of health-related effects of air pollution. Rather than relying on the mean concentration of ambient air pollutants only, these authors evaluated the numbers of hours that ambient air pollutants exceeded thresholds. For each participant in the studies, the levels of air pollutants at ZIP-code centroids were estimated for each month of residence (Abbey et al., 1989).
Neas et al. (1991) related annual average nitrogen dioxide concentration, measured for indoor pollution as a continuous variable and as 4 ordered categories, to respiratory symptoms and pulmonary function in children. A 15-ppb increase in the mean concentration was associated
with an increase in lower respiratory symptoms. These authors discuss the importance of misclassification of exposure in diluting the findings of the effects of nitrogen dioxide exposure.
In a large-scale analysis of ambient daily air pollution and mortality, Schwartz and Marcus (1990) found a strong relation between air-pollution levels and daily mortality over the period 1958-1972 in London, England. Inclusion of terms for changes in temperature and humidity increased the strength of the relation between particles and mortality. The inclusion of weather data in the analysis illustrates how indirect measures of exposure can strengthen existing associations.
Information derived from daily health diaries can be particularly important. For example, diaries can capture variability in the occurrence of symptoms, which can be related to variation in air-pollutant concentrations. Schwartz et al. (1991) described the advantages of linking ambient-air measurements and individual indicators of household exposure to daily diaries of respiratory symptoms. Diary studies record the health status of each study participant repeatedly over time and can define the impact of short-term changes in the environment on human health. In this analysis of data from the Six Cities Study of Air Pollution and Health and from a nurses' diary study in Los Angeles, exposure information was obtained from air-monitoring stations as well as from data on the type of cooking stove and history of parental and roommate smoking. A statistically significant relation between air pollution and reported symptoms remained after the effects of autocorrelation and heterogeneity were addressed. The reader is referred to volume 1 of the present report (NRC, 1991a, pp. 166-167) and the report Human Exposure Assessment for Airborne Pollutants (NRC, 1991b, pp. 157-159) for a more detailed discussion of the use of diaries.
The Health Interview Survey (HIS) of a sample of thousands of US residents was used by Ostro and Rothschild (1989) to assess the relation between acute respiratory morbidity and air pollution. The large number of subjects in this study aided in the identification of statistically significant associations. The exposure information was derived from EPA's SAROAD monitoring network of ambient-air pollution. Portnoy and Mullahy (1986) have also used the HIS data to advantage. In each study, statistically significant findings were documented despite the limited exposure assessment from ambient monitoring.
Some investigators (Bates and Sizto, 1987; Bates et al., 1990; Samet et al., 1981) have reported a relation between visits to hospital emergency departments for respiratory ailments and various types of air pollution. The statistically significant relation between ozone concentration and asthma visits was identified by a multiple regression analysis that controlled for temperature. These authors conclude that, among regions with
periodic accumulation of ozone in the ambient environment, an exposure-response relationship may be discernible.
Exposure Assessment at Hazardous-Waste Sites
Volume 1 of this report (NRC, 1991a) reviewed the exposure assessments conducted in environmental-epidemiologic investigations of hazardous wastes. The report concluded that repositories of potentially dangerous substances can be found at many hazardous-waste sites but that future risks to public health could be determined only with more-detailed information about human exposure. The report concluded that exposure assessment must be improved if we are to understand the associations among contaminants, exposures, and adverse health consequences.
One of the problems in epidemiologic studies of hazardous-waste sites has been the limited nature of the exposure assessments (NRC, 1991a). Most studies have dichotomized subjects into exposed and unexposed categories or used surrogates of exposure, such as distance from a site or residence in a defined geographic area. Only a few have estimated quantitative exposure of groups, and we know of none that have used individual exposure measurements. Problems in the accuracy of exposure data have been discussed in detail (Walter, 1991; Morgenstern, 1982; Greenland and Morgenstern, 1989). Clearly, there can be serious errors in making the assumption that the exposure level assigned to a geographic subunit applies to everyone in that unit.
Investigations of hazardous-waste sites have generally focused on which chemicals are present at or under the site (NRC, 1991a), especially the possible contamination of groundwater, and have given little attention to evaluating chemical movement from the site. Thus, information is skimpy on exposure to the population in homes or businesses near the site in question. The routine monitoring of groundwater for purposes of site remediation and problem mitigation entails significant costs (e.g., hydrogeologic characterization, boring of wells, and soil and water sampling). Government agencies have rarely assessed population exposure to chemicals from the sites.
Assessment of Past Exposure
In environmental epidemiology, information on past exposures is usually not available, and current exposure may not fairly represent the past because of technologic developments, public or government awareness of a possible problem, or other changes. Because recall bias or other types of information bias may be important in these studies, documented information on past exposure is particularly useful. In some studies,
water-consumption records have been used to estimate past exposures (Lagakos et al., 1986; Wrensch et al., 1990 a,b; Whorton et al., 1988). Mathematical modeling, toxicokinetic models, and biomarkers may improve our ability to estimate past exposures, especially where the body burden of xenobiotic chemicals is related to toxic insult.
In occupational epidemiology, it is sometimes possible to reconstruct an industrial environment for exposure estimation; exposure-monitoring records of workers may be available, and even employment records by job category may be of value. (See Applied Occupational and Environmental Hygiene, June 1991). Checkoway and colleagues (1991) have discussed methods to assess or rectify misclassification of historical exposures in occupational epidemiology (table 3-3). This work examines some indirect methods for determining possible bias in estimates of the health effects that result from nondifferential misclassification of exposure and considers misclassification of confounders. Checkoway et al. (1991) suggest that such direct approaches as simulating past exposures are often infeasible. They prefer indirect approaches for evaluating the effects of exposure and confounder misclassification.
Complex Mixtures
Waste sites may expose persons to multiple chemicals (NRC, 1991a). Three important issues arise in studying exposures to complex mixtures in epidemiologic investigations: (1) quantification of exposure, (2) characterization of potential combined or interactive effects associated with exposure to multiple chemicals, and (3) identification of subpopulations that are especially sensitive to exposure from certain complex mixtures. These problems are multiplied where the mixture may vary from one site or time to another or when the mixture is not well characterized. Both problems are common in waste sites. An entire issue of the journal Toxicology (volume 105, 1995) is devoted to an examination of chemical mixtures.
Workplace studies often focus on exposure to a single chemical agent, e.g., lead in battery manufacturing or silica in a foundry. However, for the most part, epidemiologic studies of the environment must address the issue of complex mixtures. NRC (1988) has reviewed the issues and approaches to assessing the health impact of complex mixtures. One approach involves characterization of complex mixtures by toxicologic investigation. To date, toxicologic characterization of complex mixtures has received inadequate attention as a complement to environmental investigation. The advantage of toxicologic investigation is that exposure can be set by the investigator (Ozonoff and Wartenberg, 1991). Toxicologic studies are needed to characterize both mechanisms and interactive effects and to quantitate the exposure-effect relations of complex mixtures.
Studies of exposure to the complex mixtures found in indoor air have implications for other aspects of environmental epidemiology. Samet and Lambert (1991) have pointed out that a full understanding of the health effects of indoor air pollution will require information on the effects of pollutant mixtures: "Studies of complex mixtures need to be designed with consideration of the potential patterns of combined effects of the combined pollutants. The biological effect of one pollutant may be modified by the presence of other pollutants."
Hammond (1991) and NRC (1988) have discussed the use of markers to measure exposure to complex mixtures. The study of interactive effects in environmental settings will be assisted by toxicologic evaluation and by the use of biomarkers of exposure and effect.
Indexes of Exposure
Volume 1 of this report (NRC, 1991a) discussed the concept of "dose" in the context of exposure assessment. In general, inadequate attention has been given to evaluation of the appropriate time relations between biologic models derived from mechanistic considerations and the exposure-assessment strategy. The appropriateness of determining the time course of exposure (e.g., peak exposure versus cumulative exposure) is an important methodologic issue that has received some attention in occupational epidemiology (Checkoway and Rice, 1992; Wegman et al., 1992). However, there has been little effort in environmental epidemiology to relate the levels and effects of exposure to biologic mechanisms. This may be because information on biologic mechanisms is limited or absent. It can force the analyst into ad hoc approaches to exposure assessment.
Checkoway and Rice (1992) reviewed indexes of exposure in occupational epidemiology and characterized dose surrogates by exposure intensity, exposure duration, and cumulative exposure. The appropriateness of any of these measures as dose indicators depends on the pathogenesis of the disease under consideration. Acute health outcomes have generally been associated with peak exposure intensity, whereas cumulative exposure has often been used to address chronic disease. Research using toxicokinetic models has demonstrated nonlinear relations between exposure and effect because of nonlinearities in the biologic processes (Hattis, 1990; Smith, 1992). Smith (1992) drew several conclusions about the use of cumulative exposure as a surrogate of dose for insoluble respirable dusts: (1) short, intense exposures can produce substantially higher long-term tissue doses than is implied by their cumulative exposures, and this may partially account for the observation of a disproportionately high pulmonary-disease risk for short-term workers; (2) populations with widely ranging combinations of intensity and duration may
TABLE 3-3 Crude Odds Ratios For Symptom Incidence: Exposed Compared with Control Populations
|
Symptom |
McColl(1) |
OIIa(2) |
Del Amo(3) |
Montrose (3) |
Springfellow(5) |
Purity(4) |
|
Nervousness |
1.6-5.5* |
- |
- |
- |
- |
- |
|
Headache |
1.6-7.1* |
1.8-4.6b |
2.2b |
1.2 |
1.1,1.2 |
1.05 |
|
Sleeplessness |
1.7-7.9* |
1.9-5.3b |
2.2b |
1.1 |
- |
- |
|
Fatigue |
2.6-7.0* |
1.8-3.1b |
3.0b |
1.2 |
1.3, 1.2 |
- |
|
Dizziness |
2.4-8.0* |
1.0-2.2b |
3.3b |
1.5 |
1.7,1.4 |
- |
|
Nausea |
2.1-24.5* |
2.9b |
1.6 |
- |
||
|
Loss of appetite |
1.5-17.3* |
1.5 |
1.0 |
- |
- |
|
|
Stomachache |
1.1-10.2* |
- |
- |
- |
- |
- |
|
Sinus congestion |
1.4-4.4* |
1.4-2.7b |
3.3b |
2.1b |
1.1, 1.1 |
- |
|
Blurred vision |
- |
- |
- |
- |
1.6, 2.2b |
- |
|
Eye irritation |
1.6-4.8* |
1.4-3.7b |
3.3b |
1.8b |
1.2,1.3 |
- |
|
Nose irritation |
2.0-7.5* |
- |
- |
- |
- |
- |
|
Runny nose |
2.8-5.6 |
- |
- |
- |
- |
- |
|
Sore throat |
1.8-5.9* |
1.7-2.9b |
3.5b |
2.1b |
- |
- |
|
Cough |
1.6-4.0* |
- |
- |
- |
1.2, 2.1b |
- |
|
Asthma |
- |
1.3-2.8b |
1.9 |
1.8 |
- |
- |
|
Allergies |
1.4-4.2* |
- |
1.9 |
1.8 |
- |
- |
|
Wheezing |
2.8-15.5* |
- |
- |
- |
1.2, 0.9 |
- |
|
Skin irritation |
1.1-5.0* |
3.4b |
2.3b |
- |
1.7 |
|
Symptom |
McColl(1) |
OIIa(2) |
Del Amo(3) |
Montrose (3) |
Springfellow(5) |
Purity(4) |
|
Chest pains |
1.7-4.4* |
- |
- |
- |
1.2,1.3 |
- |
|
Earaches |
1.4-3.8* |
1.5-3.1b |
3.5b |
1.6 |
1.6, 2.2 |
- |
|
Frequent urination |
- |
- |
- |
- |
1.7, 1.7b |
- |
|
Difficulty breathing |
- |
1.7-3.3b |
1.7b |
1.2 |
- |
0.8 |
|
Toothache |
- |
1.5-2.3b |
2.3b |
1.4 |
- |
1.0 |
|
Muscle aches |
- |
2.1-3.7b |
1.9b |
1.4 |
- |
1.1 |
|
Weak in extremities |
- |
- |
- |
- |
19b, 1.5 |
- |
|
Numbness in limbs |
- |
1.3-2.9b |
2.2b |
1.4 |
1.8, 1.1 |
- |
|
High environmental worry |
9% |
32% |
18% |
18% |
- |
- |
|
Worry followed illnessc |
0.5% |
7% |
8% |
8% |
- |
- |
|
Number in control area |
354 |
928 |
212 |
194 |
203 |
1,801 |
|
Total number in ''exposed" area |
703 |
1,349 |
444 |
430 |
402 |
157 |
|
|
show elevated overall risk, but no dose-response relation with cumulative exposure, because of misclassification in the high-dose categories; and (3) populations that have many subjects with low-intensity exposures may show an increased risk only in the highest group because there is no overloading of the clearance process at low exposure levels.
Smith (1992) argues that the exposure-dose relation should be examined for nonlinearity before cumulative exposure is used as a dose index. This matter has generally not been addressed in environmental studies. For example, where bioactivation of reactive molecules produces toxic metabolites, effects may show poor correlations with cumulative exposure and strong correlations with years of exposure, because metabolism is generally saturated and metabolite output is independent of exposure intensity.
Checkoway and Rice (1992) considered cumulative exposure to silica and concluded that "disentanglement of peak and cumulative exposure effects should be accomplished most effectively in investigations of relatively short-term sequelae of substances with short retention times in the body."
Subjective Symptoms and Exposure Assessment
An important issue in epidemiologic studies of hazardous-waste sites is the relation between positive findings as evidenced by increased subjective symptoms in a defined population and the general lack of exposure assessment that would link the findings to specific exposures. Some authors have suggested that the increased prevalence of subjective symptoms may derive from recall bias or psychogenic factors, whereas others have argued that symptoms may result from exposures of particularly susceptible members of a population. In volume 1, it was suggested that longitudinal studies of symptoms in response to changes in exposure could help to resolve this problem, if subjects have no knowledge of their level of exposure that could bias reporting.
Several studies have found that subjective symptoms are associated with exposures from hazardous-waste sites (Baker et al., 1988; Neutra et al., 1991). Issues of cumulative versus peak exposure appear to be relevant in these studies. In general, investigation of the averaging time of exposure indexes should be a priority in environmental epidemiology. Unfortunately, when environmental epidemiology is driven by citizen-generated concern, the data available to the investigator may be so limited that precise questions cannot be addressed. The actual chemicals to which a community population is exposed are often poorly or inadequately identified, complex mixtures may be the rule rather than the exception, and exposure routes are not well defined, so the ability to ad-
dress such complex issues as dose rate is seriously compromised (NRC, 1991a).
Neutra et al. (1991) examined the results of 5 epidemiologic studies of symptom rates observed around hazardous-waste sites. Table 3-3 lists the 5 studies and the odds ratios for symptoms with increased prevalence that reached statistical significance in these studies. The health complaints were often subjective, and the circumstances leading to the studies resulted in intense media scrutiny and even litigation. Table 3-4 lists 8 hypotheses that have been suggested to explain the higher prevalence of symptoms in exposed persons, including reporting bias. Neutra et al. (1991) conclude that excess symptoms are reported by residents who complain of odors or are worried about environmental chemicals, and they suggest the possibility that "autonomic, stress-mediated mechanics or behavioral sensitization is active in the genesis of these symptoms."
One report on the Stringfellow hazardous-waste site (Baker et al., 1988) suggested caution in the interpretation of the data: "Our experience indicates the fundamental need for health studies of toxic-waste disposal sites to be based on environmental monitoring and modeling of past exposures sufficient to identify potential exposure to specific chemicals at an individual or household level."
The study by Neutra et al. (1991) is one in a series that have examined the health effects associated with hazardous-waste disposal sites and the relation between odor and increased symptoms. A study at the Casmalia hazardous-waste site in California indicated that respiratory effects were associated with airborne releases of odorous materials from the site (Breslow et al., 1989). A report from Finland (Jaakkola et al., 1990) indicated that malodorous emissions from kraft paper pulp mills are associated with eye, nasal, and respiratory symptoms, although reporting bias could not be ruled out (NRC, 1991a). However, a study from Lowell,
TABLE 3-4 Suggested Causes of Higher Symptom Rates Near Hazardous-Waste Sites Rates Near Hazardous-Waste Sites
|
Classical toxic reaction |
|
Immunologic or other physiogenic "hazardous-waste syndrome" |
|
Behavioral sensitization |
|
Psychosomatic reaction to stress |
|
Mass psychogenic illness |
|
Reporting bias |
|
Confounding factors |
|
Odor, as an effect modifier |
|
Source: Neutra et al., 1991. |
Mass., that reported an increased prevalence of respiratory and constitutional symptoms concluded that recall bias was not a factor (Ozonoff et al., 1987).
Neutra et al. (1991) proposed protocols to test hypotheses that symptoms are associated with low-level chemical exposures and that these symptoms are early warnings of serious immunologic and neurologic dysfunction. They suggest that these would be "million-dollar studies" because they test paradigm-breaking hypotheses, and they would need to be replicated to be believed by the community in question (if that were ever to occur). However, million of dollars in tort liabilities may be associated with these sites, and the issues require resolution.
These issues clearly require careful attention to exposure evaluation and characterization. The cautions raised by Baker et al. (1988) are entirely appropriate. Improved exposure assessment is an absolute requirement if these costly and scientifically important issues are to be resolved.
Use of Biologic Markers of Exposure
The use of biologic markers has been reviewed in detail by NRC (1991a,b). Three types of biologic markers may be used to provide information on exposure (Hulka and Wilcosky, 1988; Schulte 1989; Stevens et al., 1991): markers of internal dose, such as blood lead; markers of biologically effective dose, such as blood DNA and protein adducts; and markers of biologic effects, such as chromosomal micronuclei.
The central, critical issue in the use of biologic markers of exposure, not yet adequately addressed, is criteria for their validation. NRC (1991b, p. 129) has reviewed the use of biologic markers and concluded: "A major limitation of using biological markers for exposure assessments stems from the fact that most are in a developmental stage and not fully validated or field-tested." NRC (1991b) also raised issues of the ambiguity of many markers, the variability of markers, and the difficulty of establishing links between exposure and effect. Criteria governing the validation and use of biologic markers were described in detail in that report.
Designing and Conducting a Study with Biologic Markers
When environmental-monitoring data are available for classifying individuals, it may not be necessary to perform biologic monitoring. Biologic monitoring of a sample of subjects can be used to validate environmental-monitoring variables. If there is a close correspondence between exposure and the biologic marker, then further use of the more-costly biologic monitoring may not be needed and the exposure classification scheme may be considered validated. If the correspondence is not close,
it may be that exposure from diverse sources or by various rates is not covered by environmental monitoring. For example, classification of residents by distance from an arsenic smelter may not adequately reflect the arsenic concentrations in their diets, and environmental monitoring may not provide good estimates of total arsenic exposure.
Biologic markers are often assumed to be good indicators of exposure because they represent the integrated exposure from various sources and through various routes. However, to assess this assumption requires correlation of the marker with the potentially less-adequate environmental measure. There is no "gold standard." Perfect correspondence between the marker and the exposure could mean that neither is better than the other or that there are no other routes, sources, or host factors that intervene. On the other hand, it may mean that the marker is not an accurate reflection of these other intervening factors. It is important to determine whether the marker shows an exposure-response relation, whether all potential routes are accounted for, and whether susceptibility or host factors are addressed.
Host factors, including behavioral factors and genetic characteristics, may influence the amount of a toxic agent that interacts with critical macromolecules in cells and tissues. This is the "biologically effective dose." The biologically effective dose assesses exposure from all routes and sources as well as some aspects of effect modification, possibly including host characteristics for uptake, metabolism, absorption, and excretion. However, the marker may not necessarily encompass all these factors. Thus, even when biomarkers are useful, the best appraisal of exposure may still include ambient and environmental measurements as well as biologic measurements.
Numerous biomarker-related issues may arise during the conduct of studies, including questions of specimen collection, transport, storage, and assay; measurement error of technical variables in the assay; biologic variability; and assay interpretation and communication of results.
In cohort studies, biologic markers may be measured in subsets of populations, such as in a nested case-control or case-cohort approach, to assess etiologic questions and mechanisms and to identify high-risk subpopulations. In these situations, biologic markers of exposure may be useful to (1) distinguish exposure subgroups, (2) determine whether there is a relation between exposure and dose, or (3) evaluate the relation between exogenous exposure and internal or biologically effective dose.
Biologic markers may also be useful to identify the effect of an intervention. For example, does reduction of environmental emissions result in a reduction in the level of DNA adducts? Research studies to assess interventions need to include assessment of baseline levels of biomarkers in order to interpret the effect of the interventions.
Interpretation and Generalization of Study Results
Biologic markers of exposure can be of use after a study has been completed. For example, if researchers wish to see how well the results of a completed study might apply to a broad population, they may sample the population for the distribution of a particular marker to determine whether exposures are constant over a wide range of geographic conditions, demographic descriptors, and occupations. Even if the original study did not measure such factors, biologic markers may clarify what exposures such target groups may have experienced.
It may also be possible to perform individual risk assessments using biologic measures of exposure. A classic example of individual risk assessment is the use of serum-cholesterol measurement to predict disease risk (Truett et al., 1967). With more-recent technology, one might attempt precise individual risk assessment by studying an individual's specific spectrum of gene mutations from specific exposures to a carcinogen.
Data Gaps, Research Recommendations, and Resource Limitations
Few biologic markers of exposure have been validated. As indicated above, validation of a marker of exposure requires an understanding of the dosimetric characteristics pertaining to the time between exposure and the ascertainment of the markers, the degree to which the marker represents exposure, and the nature and shape of the exposure-marker relation (Schulte 1989; Stevens et al., 1991). Little is known about the prevalence, range of variability, persistence, and confounding factors of many candidate exposure markers. Such information must be collected before these can be used with confidence in environmental-epidemiologic studies. Markers of the biologically effective dose require additional research to assess the role of host factors, particularly genetic susceptibilities, as effect modifiers.
Dosimetric Modeling
More attention is being devoted to characterizing the quantity and timing of toxic chemical agents' reaching target tissue, because concentrations measured by microenvironmental monitoring or even personal dosimetry may not accurately reflect target-tissue dose. This has led to greater emphasis on mathematical models derived from biologic mechanisms of toxicity. This approach has historically been the focus of pharmacologists who have sought to develop appropriate models to characterize the relation between drug efficacy and dose. Mathematical
modeling of tissue dose, biologically effective dose, or internal dose is receiving greater attention in addressing toxicologic issues. However, there has been little attention to the relation between these models, hereafter referred to as dosimetric models, and classic epidemiologic models that estimate disease risk. Kriebel (1991) has discussed the importance of this approach to epidemiologic investigation: "Often it is difficult or impossible to accurately estimate the exposure experience of each member of a cohort, and so various kinds of proxy variables must be used. It is well known that the use of these proxies can introduce misclassification of exposure, often leading to underestimation of the magnitude of exposure-disease associations. Even when accurate exposure data are available, serious bias may still occur if these data are used in a mis-specified epidemiologic model to estimate an exposure-response relationship."
Kriebel discusses several tenets for the use of dosimetric models. These tenets result in a 2-phase approach to epidemiologic investigation: first, is the development of a mathematical model to estimate individual doses; and second, the use of epidemiologic models to estimate the risk of disease associated with these estimated doses, with appropriate control for confounding. The advantage of this approach for environmental epidemiology is that the dosimetric model can quantify a hypothesis about uptake processes and metabolism of the chemicals in question and may provide insight into the biologic mechanisms of effect. This facilitates the overall design of the epidemiologic model and subsequent analysis. That is, the dosimetric model informs the exposure assessor and epidemiologist and provides a way to reduce misclassification and improve the precision of the study. These concepts have been applied by Hattis (1990), Smith (1992), Hodgson and Jones (1990), Vineis and Terracini (1990), Vincent and Mark (1988), Pinto et al. (1978), and Kriebel and Smith (1990).
Smith (1985) developed a compartmental dosimetric model of dust deposition for an occupational-epidemiologic study of pulmonary fibrosis in silicon carbide workers. In discussing the Smith model, Kriebel (1991) asks whether the considerable effort that goes into the construction and use of such a model was justified. He raises 3 criteria that could be used to evaluate any particular model: (1) the better model will fit the data better, (2) the better model will accommodate such secondary characteristics of the exposure-response relation as interactions with other agents (effect modifiers) and time (such as latency), and (3) the predictions of the better model can be generalized to other exposure situations. Smith (1991) also discusses the use of toxicokinetic modeling for epidemiologic purposes and argues that the use of toxicokinetic models can differentiate among hypotheses about the mechanisms that underlie the relation between exposure and effects.
The greatest overall contribution made by a 2-stage approach to epi-
demiology (as described by Kriebel, 1991) is to provide a framework within which all available data, including toxicologic information and experimental data, can be viewed. In fact, animal data may be essential to the development of some models. Ultimately, dosimetric models are mathematical expressions of formal hypotheses about the underlying physiologic processes that are the basis of the exposure-response relation (Kriebel, 1991).
Training in Environmental-Exposure Assessment
Human exposure assessment is inadequately addressed in most environmental-epidemiology studies, and one of the roots of this problem is the lack of training at the graduate level. There is a major need, in the United States and elsewhere, for the development of training programs in exposure assessment. There are extensive master's-degree programs for industrial hygiene as a result of the National Institute for Occupational Safety and Health's Educational Resource Center program and other extramural project grants for training. There is at least one similar training program that has environmental-exposure assessment as its focus—in the Department of Environmental Science at Rutgers University (Lioy, 1991b). Exposure assessment is addressed in courses on environmental risk assessment, but even there the context is more focused on risk assessment and site remediation than on epidemiologic investigation of public-health hazards. Training in exposure assessment must be multidisciplinary, with a multimedia approach, and should address all the major uses of exposure information—including risk assessment, epidemiology, environmental control, and exposure assessment—and industrial hygiene, toxicology, pollution prevention, and standard-setting. It would be useful to examine the relations among needs for training in these areas to define a new curriculum that would better address current and future needs.
Given the costs, resource requirements, and political sensitivity of many environmental-epidemiologic studies, the failure to provide training for environmental assessment will need to be addressed by policy-makers and educators if we are to have substantial improvement in environmental epidemiology and risk assessment.
Conclusions
Exposure assessment is important in all environmental-epidemiologic studies. A wide range of exposure-assessment strategies and techniques are available for use in environmental-epidemiologic investigation. Associations have been clarified by improved use of exposure assessment even where indirect methods have been used.
Both direct measures (personal and biologic monitoring and biomarkers) and indirect measures (microenvironmental monitoring, diaries, and mathematical modeling) can be used for exposure assessment in environmental epidemiology. Each of these techniques has advantages and disadvantages. Their optimal use depends on the nature of the study, the biologic hypothesis, and resource constraints. No approach should be singled out as being the only acceptable strategy, e.g., personal monitoring. All approaches have validity and will improve the study if used appropriately.
Better exposure assessment in environmental epidemiology will increase the power of studies to find associations. However, within a fixed budget, spending more money on exposure assessment per subject will reduce the number of subjects who can be studied and hence could reduce statistical power. The tradeoff between precision and the cost of larger samples means that power will not increase monotonically with improvements in the accuracy of the exposure assessment. In studies of multifactorial outcomes and low relative risks, a large sample is almost always required. This means that inexpensive methods for modest improvement of rough and inexpensive exposure assessment may be more valuable than more-accurate but expensive methods. This includes very inexpensive methods, such as the use of questionnaire data on activity patterns.
Studies of large populations exposed to mixtures of air pollutants should incorporate detailed estimates of exposure, including detailed activity logs (including transit to work or school), the kind of air conditioning in the home and workplace, and the use of personal monitors to validate models in subsets of the population under study.
The problems of exposure measurement in persons living close to hazardous-waste sites were discussed in volume 1. Most studies have been structured around an "exposed-unexposed" classification or have used surrogates of exposure, such as distance from the waste site. Estimation of past exposures is particularly difficult and unreliable. Misclassification is likely to be a crucial problem in studies of this nature, and improved characterization of exposure is a priority.
The estimation of cumulative doses is an important component of many occupational-exposure studies, though such measures may not be valid even in occupational settings. The exposure-dose relation should be examined for nonlinearity before cumulative estimates are calculated. The relation between cumulative exposure and peak exposure is unknown in many environmental-epidemiologic studies, particularly those involving hazardous-waste site exposures or community exposures to episodic pollution.
The characterization of complex mixtures is a continuing problem for
exposure assessment. Four priorities in addressing complex mixtures are quantification of exposure to complex mixtures, characterization of combined or interactive effects, toxicologic characterization of the complex mixture in question, and identification of subpopulations that may be especially sensitive to one or more of the components of certain complex mixtures.
Biologic markers of exposure can strengthen environmental-epidemiologic studies. Unfortunately, few such markers are yet feasible in field studies, and few have been adequately validated. Efforts to improve and refine such indicators are important. The feasibility and value of banking blood samples for future analysis should be considered as studies are designed. Biomarkers of changes induced in the immune system of human subjects are needed.
Health effects are often subtle, and risks are difficult to estimate. As a result, more attention is being given to the estimation of target-tissue dose in ways that reduce misclassification and improve precision. Development of mathematical models to estimate target-tissue dose (toxicokinetic modeling) that may be combined with epidemiologic models to estimate risk is a new and important area of research.
Emphasis should be given to the development of training programs in environmental-exposure assessment. Improvement in the development and use of new techniques in exposure assessment is a high priority in environmental epidemiology.
Data should of course be generated and collected under rigorous conditions of quality control. Bias must be minimized, and variance must be both minimized and estimated when quantitative conclusions are to be drawn. Measures of and checks on data quality should be prominent in every manuscript and report, and authors must not be reticent in bringing out the mechanisms of their study—and there will always be weaknesses. Because of the difficulties of conducting epidemiologic studies, both descriptive and analytic, it is rare for any one study to be definitive, and this is especially true in environmental epidemiology. Every public presentation, written or oral, including reports to scientific colleagues, should contain prominent caveats about overinterpretation.
References
Abbey, D.E., G.L. Euler, J.K. Moore, F. Petersen, J.H. Hodgkins, and A.R. Magie. 1989. Applications of a method for setting air quality standards based on epidemiological data. J. Air Pollut. Control Assoc. 39:437-445.
Abbey, D.E., P.K. Mills, F.F. Petersen, and W.L. Beeson. 1991. Long-term ambient concentrations of total suspended particulates and oxidants as related to incidence of chronic disease in California Seventh-Day Adventists. Environ. Health Perspect. 94:43-50.
ATSDR (Agency for Toxic Substances and Disease Registry). 1994. Environmental Data Needed for Public Health Assessments: A Guidance Manual. NTIS PB-179827. Atlanta: USDHHS, ATSDR, Division of Health Assessment and Consultation.
Bailar, J.C. 1989. Inhalation hazards: the interpretation of epidemiologic evidence. Pp. 3948 in D. V. Bates et al., eds. Assessment of Inhalation Hazards, New York: Springer-Verlag.
Baker, D.B., S. Greenland, J. Mendlein, and P. Harmon. 1988. A health study of two communities near the Stringfellow waste disposal site. Arch. Environ. Health 43:325-334.
Bates, D.V., and R. Sizto. 1987. Hospital admissions and air pollution in southern Ontario: the acid summer haze effect. Environ. Res. 43:317-331.
Bates, D.V., M. Baker-Anderson, and R. Sizto. 1990. Asthma attack periodicity: a study of hospital emergency visits in Vancouver. Environ. Res. 51:51-70.
Blair, A., and S. Zahm. 1990a. Methodologic issues in exposure assessment for case-control studies of cancer and herbicides. Am. J. Ind. Med. 18:285-293.
Blair, A., and S. Zahm. 1990b. Herbicides and cancer: a review and discussion of methodologic issues. Pp. 132-145 in P. Band, ed. Recent Results in Clinical Cancer Research. Vol. 120. Occupational Epidemiology. New York: Springer-Verlag.
Blair, A., and S. Zahm. 1992. Epidemiology Studies Of Cancer Among Agricultural Populations. Presented at Third International Symposium: Issues in Health, Safety and Agriculture. Saskatoon, Saskatchewan, Canada, May 10-15, 1992.
Breslow, L., et al. 1989. Report of Santa Barbara Commission on Health Consequences of the Casmalia Resources Waste Disposal Facility. Report prepared for Santa Barbara Department of Health Services, California.
Brown, L.M., M. Dosemeci, A. Blair, and L. Burmeister. 1991. Comparability of data obtained from farmers and surrogate respondents on use of agricultural pesticides. Am. J. Epidemiol. 134:348-355.
Burke, T.A., and K. Sexton. 1995. Integrating science and policy in a national human exposure assessment survey. J. Exposure Anal. Environ. Epidemiol. 5:283-296.
Burke, T.A., J. Creason, P. Ryan, and M. Schwab. 1992. An Approach to the Design of the National Health Exposure Assessment Survey (NHEXAS): A Consolidated Report. Preliminary draft for NHEXAS Advisory Committee. 52 pp.
Checkoway, H., and C.H. Rice. 1992. Time-weighted averages, peaks, and other indices of exposure in occupational epidemiology. Am. J. Ind. Med. 21(1):25-33.
Checkoway, H., D.A. Savitz, and N.J. Heyer. 1991. Assessing the effects of nondifferential misclassification of exposures in occupational studies. App. Occup. Environ. Hyg. 6:528-533.
Copeland, K.T., H. Checkoway., A.J. McMichael, and R.H. Holbrook. 1977. Bias due to misclassification in the estimation of relative risk. Am. J. Epidemiol. 105:488-495.
Dosemici, M., S. Wacholder, and J.H. Lubin. 1990. Does nondifferential misclassification of exposure always bias a true effect toward the null value? Am. J. Epidemiol. 132:746-748.
EPA (US Environmental Protection Agency). 1988. Future Risk: Research Strategies for the 1990s. SAB-EC-88-040. Washington, DC: US Environmental Protection Agency Science Advisory Board. 19 pp.
EPA (US Environmental Protection Agency). 1992. Guidelines for exposure assessment: notice. Federal Register, Part VI, Vol. 57, No. 104.
Euler, G.L., D.E. Abbey, J. E. Hodgkin, and A.R. Magie. 1987. Chronic obstructive pulmonary disease symptom effects of long-term cumulative exposure to ambient levels of total suspended particulates and sulfur dioxide in California Seventh-Day Adventist residents. Arch. Environ. Health 42:213-222.
Euler, G.L., D.E. Abbey, J.E. Hodgkin, and A.R. Magie. 1988. Chronic obstructive pulmonary disease symptom effects of long-term cumulative exposure to ambient levels of total oxidants and nitrogen dioxide in California Seventh-Day Adventist residents. Arch. Environ. Health 43:279-285.
Freeman, N.C., J.M. Waldman, and P.J. Lioy. 1991. Design and evaluation of a location and activity log used for assessing personal exposure to air pollutants. J. Expo. Anal. Environ. Epidemiol. 1:327-338.
Gann, P. 1986. Use and misuse of existing data bases in environmental epidemiology: the case of air pollution. Pp. 109-122 in F. Kopfler and G.F. Craun, eds. Environmental Epidemiology. Chelsea, MI: Lewis.
Greenland, S., and H. Morgenstern. 1989. Ecological bias, confounding, and effect modification. Int. J. Epidemiol. 18:269-274. [Published erratum appears in Int. J. Epidemiol. 1991 20:824.]
Hammond, S.K. 1991. The uses of markers to measure exposures to complex mixtures. Pp. 53-66 in L.M. Rappaport, and T. Smith, eds. Exposure Assessment for Epidemiology and Hazard Control. Chelsea, MI: Lewis.
Hasabelnaby, N.A., J.H. Ware, and W.A. Fuller. 1989. Indoor air pollution and pulmonary performance: investigating errors in exposure assessment. Stat. Med. 8:1109-1126; discussion 1137-1138.
Hattis, D. 1990. Pharmacokinetic principles for dose-rate extrapolation of carcinogenic risk from genetically active agents. Risk Anal. 10:306-316.
Hodgson, J.T., and R.D. Jones. 1990. Mortality of a cohort of tin miners 1941-86. Br. J. Ind. Med. 47:665-676. [Published erratum appears in Br. J. Ind. Med. 1990 47:846.]
Hulka, B.S., and T. Wilcosky. 1988. Biological markers in epidemiologic research. Arch. Environ. Health 43:83-89.
Jaakkola, J.K., V. Vilkka, O. Marttiila, P. Jappinen, and T. Haahtela. 1990. The South Karelia air pollution study: the effects of malodorous sulfur compounds from pulp mills on respiratory and other symptoms. Am. Rev. Respir. Dis. 142:1344-1350.
Kennedy, S.M., D.A. Enarson, R.G. Janssen, and M. Chan-Yeung. 1991. Lung health consequences of reported accidental chlorine gas exposure among pulpmill workers. Am. Rev. Respir. Dis. 143(1):74-79.
Kriebel, D. 1991. The Dosimetric Model in Epidemiology. Invited paper presented at 8th International Symposium: Epidemiology in Occupational Health, September 10-12, Paris, France. Organized under auspices of International Commission on Occupational Health, Scientific Committee on Epidemiology. 21 pp. Proceedings in press.
Kriebel, D., and T.J. Smith. 1990. A nonlinear pharmacologic model of the acute effect of ozone on the human lungs. Environ. Res. 51:120-146.
Lagakos, S.W., B.J. Wessen, and M. Zelen. 1986. An analysis of contaminated well water and health effects in Woburn, Massachusetts. J. Am. Stats. Assoc. 81:583-596.
Landrigan, P.J. 1983. Epidemiologic approaches to persons with exposures to waste chemicals. Environ. Health Perspect. 48:93-97.
Levine, S.P., R. J. Costillo, C.L. Geraci, and K.A. Conlin. 1985. Air monitoring at the drum bulking process of a hazardous waste remedial action site. Am. Ind. Hyg. Assoc. J. 46:192-196.
Lioy, P.J. 1990. Assessing total human exposure to contaminants: a multidisciplinary approach. Environ. Sci. Technol. 24:938-945.
Lioy, P.J. 1991a. Arguments on the Adequacy of Exposure Indices and Convergence with Health Indices of the Adverse Effects of Air Pollution. Paper given at the 91st Annual AWMA Meeting, Vancouver, British Columbia.
Lioy, P.J. 1991b. Human exposure assessment: a graduate level course. J. Expo. Anal. Care Environ. Epidemiol. 1:271-281.
Lioy, P.J., N.C.G. Freeman, I. Walnman, A.H. Ster, R. Boesch, T. Howell , and S.I. Shupack. 1992. Microenvironmental analysis of residential exposure to chromium laden wastes in and around New Jersey homes. Risk Anal. 12:287-299. [Published erratum appears in Risk Anal. 1992. 12:463.]
Mills, P., D. Abbey, W. L. Beeson, and F. Petersen. 1991. Ambient air pollution and cancer in California Seventh-Day Adventists. Arch. Environ. Health 46:271-280.
Monster, A.C., and J.F.F. Smolders. 1984. Tetrachloroethene in exhaled air of persons living near pollution sources. Int. Arch. Occup. Environ. Health 53:331-336.
Morgenstern, H. 1982. Uses of ecologic analysis in epidemiologic research. Am. J. Pub. Health 72:1336-1344.
Neas, L.M., D.W. Dockery, J.H. Ware, J.D. Spengler, F.E. Speizer, and B.G. Ferris, Jr. 1991. Association of indoor nitrogen dioxide with respiratory symptoms and pulmonary function in children. Am. J. Epidemiol. 134:204-219.
Neutra, R., J. Lipscomb, K. Satin, and D. Shusterman. 1991. Hypotheses to explain the higher symptom rates observed around hazardous waste sites. Environ. Health Perspect. 94:31-38.
NRC (National Research Council). 1988. Complex Mixtures: Methods for In Vivo Toxicity Testing. Washington, DC: National Academy Press. 227 pp.
NRC (National Research Council). 1991a. Environmental Epidemiology, Vol. 1. Public Health and Hazardous Wastes. Washington, DC: National Academy Press.
NRC (National Research Council). 1991b. Human Exposure Assessment for Airborne Pollutants. Washington, DC: National Academy Press.
NRC (National Research Council). 1991c. Monitoring Human Tissues for Toxic Substances. Washington, DC: National Academy Press. 211 pp.
Ostro, B., and S. Rothschild. 1989. Air pollution and acute respiratory morbidity: an observational study of multiple pollutants. Environ. Res. 50:238-247.
Ozonoff, D., and D. Wartenberg. 1991. Toxic exposures in a community setting: the epidemiologic approach. Pp. 77-88 in Molecular Dosimetry and Human Cancer. Boston: CRC Press.
Ozonoff, D., M.E. Colten, A. Cupples, T. Heeren, A. Sachatzkin, T. Mangione, M. Dresner, and T. Colton. 1987. Health problems reported by residents of a neighborhood contaminated by a hazardous waste facility. Am. J. Ind. Med. 11:581-597.
Peters, J. 1991. Epidemiologic Investigation to Identify Chronic Effects of Ambient Air Pollution in Southern California. Proposal to California Air Resources Board.
Pinto, S.S., V. Henderson, and P.E. Enterline. 1978. Mortality experiences of arsenic-exposed workers. Arch. Environ. Health 33:325-331.
Pirkle, J. L., L. L. Needham, and K. Sexton. 1995. Improving exposure assessment by monitoring human tissues for toxic chemicals. J. Exposure Anal. Environ. Epi. 5:405-424
Portnoy, P., and J. Mullahy. 1986. Urban air quality and acute respiratory illness. J. Urban Economics 20:21-28.
Ryan, P. 1991. An overview of human exposure modeling. J. Exp. Anal. Environ. Epidemiol. 1:453-474.
Samet, J.M., and W.E. Lambert. 1991. Epidemiologic approaches for assessing health risks from complex mixtures in indoor air. Environ. Health Perspect. 95:71-74.
Samet, J.M., F.E. Speizer, Y. Bishop, J.D. Spengler, and B.G. Ferris, Jr. 1981. The relationship between air pollution and emergency room visits in an industrial community. J. Air Pollution Control Assoc. 31:236-240.
Schulte, P. 1989. A conceptual framework for the validation and use of biological markers. Environ. Res. 48:129-144.
Schwab, M., A.P. Treblanche, and J.D. Spengler. 1991. Self-reported exertion levels on time/ activity diaries: application to exposure assessment. J. Expo. Care Anal. Environ. Epidemiol. 1:339-356.
Schwartz, J., and A. Marcus. 1990. Mortality and air pollution in London: a time series analysis. Am. J. Epidemiol. 131:185-194.
Schwartz, J., D. Wypij, D. Dockery, J. Ware, S. Zeger, J. Spengler, and B. Ferris, Jr. 1991. Daily diaries of respiratory symptoms and air pollution: methodological issues and results. Environ. Health Perspect. 90:181-187.
Sexton, K. 1991. National Human Exposure Assessment Survey. EPA (Environmental Protection Agency). Position paper. Washington, DC: Office of Health Research.
Sexton, K., and P.B. Ryan. 1989. Human Exposure to Air Pollution: Methods, Measurements, and Models. In: A. Watson, RR Bates, and D. Kennedy, eds. Air Pollution, the Automobile, and Public Health. Washington, DC: National Academy Press.
Sexton, K., M. A. Callahan and E. F. Bryan. 1995. Estimating exposure and dose to characterize health risks: the role of human tissue monitoring in exposure assessment. Environ. Health Perspect. 103 (Suppl.): 13-29.
Smith, T.J. 1985. Development and application of a model for estimating alveolar and interstitial dust levels. Ann. Occup. Hyg. 29:495-516.
Smith, T.J. 1991. Pharmacokinetic models in the development of exposure indicators in epidemiology. Ann. Occup. Hyg. 35:543-560.
Smith, T.J. 1992. Occupational exposure and dose over time: limitations of cumulative exposure . Am. J. Ind. Med. 21:35-51.
Stern, A.H., N.C.G. Freeman, P. Pleban, R.R. Boesch, T. Wainman, T. Howell, S.I. Shupack, B.B. Johnson, and P.J. Lioy. 1992. Residential exposure to chromium waste-urine biological monitoring in conjunction with environmental exposure monitoring. Environ. Res. 58:147-162.
Stevens, D.K., R.J. Bull, C.H. Nauman, and J.N. Blancato. 1991. Decision model for biomarkers of exposure. Regul. Toxicol. Pharmacol. 14:286-296.
Truett, J., J. Cornfield, and W. Kannel. 1967. A multivariate analysis of the risk of coronary heart disease in Framingham. J. Chronic Dis. 20:511-524.
Vincent, J.H., and D. Mark. 1988. The Measurement of Aerosols in Relation to Risk Assessment. International Workshop on Exposure Assessment for Epidemiology and Hazard Control, Woods Hole, Massachusetts, 1988.
Vineis, P., and B. Terracini. 1990. Biochemical epidemiology of bladder cancer. Epidemiology 1:448-452.
Wallace, L.A. 1991. Personal exposure to 25 volatile organic compounds. EPA's 1987 team study in Los Angeles, California. Toxicol. Ind. Health. 7:203-208.
Wallace, L.A., E.D. Pellizzari, T.D. Hartwell, R. Whitmore, C. Sparacino, and H. Zelon. 1986. Total Exposure Assessment Methodology (TEAM) study: personal exposures, indoor-outdoor relationships, and breath levels of volatile organic compounds in New Jersey. Environ. Int. 12:369-388.
Wallace, L.A., E.D. Pellizzari, T.D. Hartwell, C. Sparacino, R. Whitmore, L. Sheldon, H. Zelon, and R. Perritt. 1987. The TEAM (Total Exposure Assessment Methodology) study: personal exposures to toxic substances in air, drinking water, and breath of 400 residents of New Jersey, North Carolina, and North Dakota. Environ. Res. 43:290-307.
Wallace, L.A., E.D. Pellizzari, T.D. Hartwell, R. Whitmore, H. Zelon, R. Perritt, and L. Sheldon. 1988. California TEAM study: breath concentrations and personal air exposures to 26 volatile compounds in air and drinking water of 188 residents of Los Angeles, Antioch, and Pittsburgh, California. Atmos. Environ. 22:2141-2164.
Walter, S.D. 1991. The ecologic method in the study of environmental health. II . Methodologic issues and feasibility. Environ. Health Perspect. 94:67-73.
Wegman, D.H., E.A. Eisen, S.R. Woskie, and X. Hu. 1992. Measuring exposure for the epidemiologic study of acute effects. Am. J. Ind. Med. 21(1):77-89.
Whorton, M.D., R.W. Morgan, O. Wong, S. Larson, and N. Gordon. 1988. Problems associated with collecting drinking water quality data for community studies: a case example, Fresno County, California. Am. J. Pub. Health. 78:43-46.
Wrensch, M., S. Swan, P.J. Murphy, J. Lipscomb, K. Claxton, D. Epstein, and R. Neutra. 1990a. Hydrogeologic assessment of exposure to solvent-contaminated drinking water: pregnancy outcomes in relation to exposure. Arch. Environ. Health 45:210-216.
Wrensch, M., S. Swan, J. Lipscomb, D. Epstein, L. Fenster, K. Claxton, P.J. Murphy, D. Shusterman, and R. Neutra. 1990b. Pregnancy outcomes in women potentially exposed to solvent-contaminated drinking water in San Jose, California. Am. J. Epidemiol. 131:283-300.










































